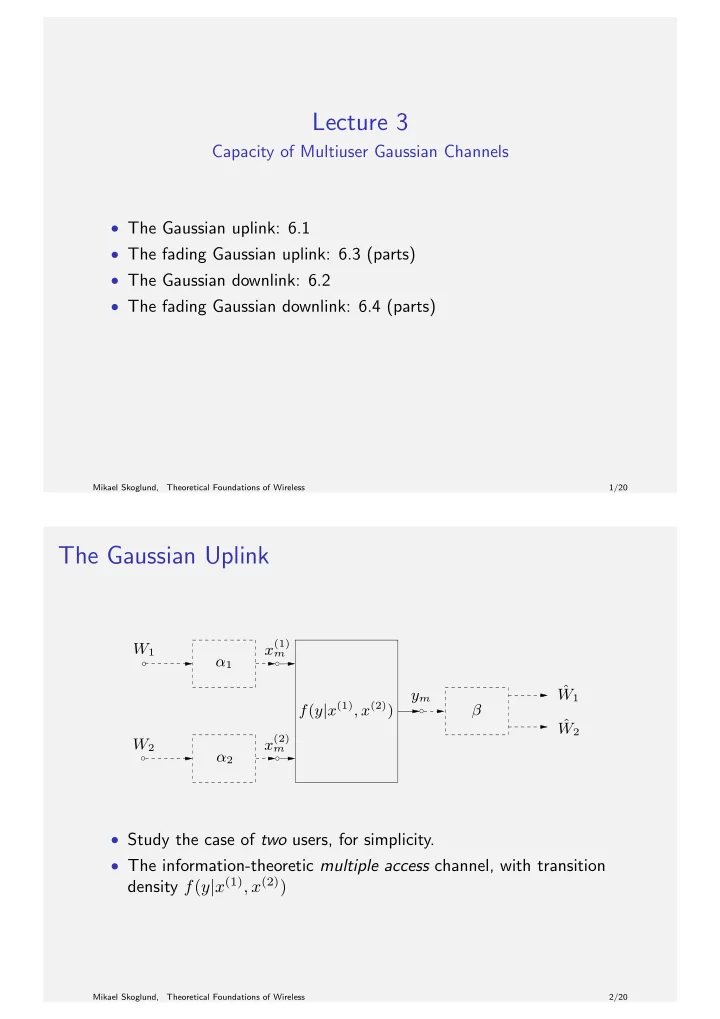

Lecture 3 Capacity of Multiuser Gaussian Channels • The Gaussian uplink: 6.1 • The fading Gaussian uplink: 6.3 (parts) • The Gaussian downlink: 6.2 • The fading Gaussian downlink: 6.4 (parts) Mikael Skoglund, Theoretical Foundations of Wireless 1/20 The Gaussian Uplink x (1) W 1 m α 1 ˆ y m W 1 f ( y | x (1) , x (2) ) β ˆ W 2 x (2) W 2 m α 2 • Study the case of two users, for simplicity. • The information-theoretic multiple access channel, with transition density f ( y | x (1) , x (2) ) Mikael Skoglund, Theoretical Foundations of Wireless 2/20
• The Gaussian multiple access channel: y m = x (1) m + x (2) m + w m , where { w m } is i.i.d complex Gaussian CN (0 , σ 2 ) • Coding : • Data: W 1 ∈ I 1 = { 1 , . . . , M 1 } and W 2 ∈ I 2 = { 1 , . . . , M 2 } , • uniformly distributed and independent • Encoders: α 1 : I 1 → C n and α 2 : I 2 → C n • Power constraints: n n 1 1 m | 2 ≤ P 1 , m | 2 ≤ P 2 X | x (1) X | x (2) n n m =1 m =1 • Rates: R 1 = log M 1 /n and R 2 = log M 2 /n • Decoder: β : C n → I 1 × I 2 , β ( y n 1 ) = ( ˆ W 1 , ˆ W 2 ) • Error probability: ( ˆ W 1 , ˆ P ( n ) ` ´ W 2 ) � = ( W 1 , W 2 ) = Pr e Mikael Skoglund, Theoretical Foundations of Wireless 3/20 • Capacity : Two (or more) rates, R 1 and R 2 = ⇒ cannot consider one maximum achievable rate = ⇒ study sets of achievable rate-pairs ( R 1 , R 2 ) , • achievable rate-pair : ( R 1 , R 2 ) is achievable if ( α 1 , α 2 , β ) n exist such that P ( n ) → 0 as n → ∞ e • capacity region : C = the closure of the set of all achievable ( R 1 , R 2 ) Mikael Skoglund, Theoretical Foundations of Wireless 4/20
• Capacity-region of the Gaussian uplink : ( R 1 , R 2 ) ∈ C if and only if � � 1 + P 1 R 1 ≤ log σ 2 � 1 + P 2 � R 2 ≤ log σ 2 � 1 + P 1 + P 2 � R 1 + R 2 ≤ log σ 2 Mikael Skoglund, Theoretical Foundations of Wireless 5/20 • The two-user Gaussian multiple access region (figure from the textbook). Noise variance σ 2 = N 0 . Mikael Skoglund, Theoretical Foundations of Wireless 6/20
• Achieving capacity : • Independent ’Gaussian codebooks’ C 1 and C 2 , rates R 1 and R 2 , powers P 1 and P 2 • Users encode W 1 and W 2 independently using C 1 and C 2 • To achieve point ’B’ in the figure, use interference cancellation , • decode user 1 treating the codeword of user 2 as noise • subtract the codeword of user 1 • decode user 2 • Change order to achieve ’A’ • Points on the segment AB achieved by time sharing • The points on AB are ’optimal’ Mikael Skoglund, Theoretical Foundations of Wireless 7/20 • Orthogonal multiple access ; take TDMA for simplicity, let α ∈ [0 , 1] : • user 1 uses the channel a fraction α of time • user 2 uses the channel a fraction (1 − α ) of time gives the region � 1 + P 1 � R 1 ≤ α log ασ 2 � � P 2 R 2 ≤ (1 − α ) log 1 + (1 − α ) σ 2 Any orthogonal scheme will give an equivalent expression Mikael Skoglund, Theoretical Foundations of Wireless 8/20
R 2 general T/F-DMA R 1 Capacity region for P 1 = P 2 . Note that T/F-DMA is only optimal when α/ (1 − α ) = P 1 /P 2 . Mikael Skoglund, Theoretical Foundations of Wireless 9/20 • K - users , • capacity region : straightforward generalization. . . • sum capacity : some of the rates K P „ k P k « X R k < C sum = log 1 + σ 2 k =1 are always achievable ⇒ sum rates < C sum achievable • symmetric capacity : C sym = largest R such that R 1 = R 2 = · · · = R K = R are in the capacity region. With P 1 = P 2 = · · · = P K = P we get „ « C sym = 1 1 + KP K log σ 2 • can be achieved with orthogonal access Mikael Skoglund, Theoretical Foundations of Wireless 10/20
The Fading Gaussian Uplink • Slow fading, perfect CSIR, no CSIT : • Received signal y m = h 1 x (1) m + h 2 x (2) m + w m { w m } is i.i.d complex Gaussian CN (0 , σ 2 ) , and h i , i = 1 , 2 , are fixed channel gains, drawn according to f ( h 1 , h 2 ) • Conditional capacity region : ( R 1 , R 2 ) ∈ C ( h 1 , h 2 ) iff 1 + | h 1 | 2 P 1 „ « R 1 ≤ log σ 2 1 + | h 2 | 2 P 2 „ « R 2 ≤ log σ 2 1 + | h 1 | 2 P 1 + | h 2 | 2 P 2 „ « R 1 + R 2 ≤ log σ 2 Mikael Skoglund, Theoretical Foundations of Wireless 11/20 • Outage probability : The probability that coding at rates ( R 1 , R 2 ) fails, p ul � � out = Pr ( R 1 , R 2 ) / ∈ C ( h 1 , h 2 ) • ε - outage capacity region : The closure of the set { ( R 1 , R 2 ) : p ul out ( R 1 , R 2 ) ≤ ε } Mikael Skoglund, Theoretical Foundations of Wireless 12/20
• Fast fading, perfect CSIR, no CSIT : • Received signal y m = h (1) m x (1) m + h (2) m x (2) m + w m { w m } is i.i.d complex Gaussian CN (0 , σ 2 ) , and h ( i ) m , i = 1 , 2 , are jointly stationary and ergodic ⇒ ( R 1 , R 2 ) ∈ C iff " !# 1 + | h (1) m | 2 P 1 R 1 ≤ E log σ 2 " !# 1 + | h (2) m | 2 P 2 R 2 ≤ E log σ 2 " !# 1 + | h (1) m | 2 P 1 + | h (2) m | 2 P 2 R 1 + R 2 ≤ E log σ 2 • Fast fading, perfect CSIR, perfect CSIT : Next lecture Mikael Skoglund, Theoretical Foundations of Wireless 13/20 The Gaussian Downlink Channel ˆ y (1) W 1 m β 1 ( W 1 , W 2 ) x m α f ( y (1) , y (2) | x ) y (2) ˆ W 2 m β 2 • The information-theoretic broadcast channel, with transition density f ( y (1) , y (2) | x ) Mikael Skoglund, Theoretical Foundations of Wireless 14/20
• Degraded broadcast channel , y 1 x y 1 y 2 x ⇔ f ( y 1 , y 2 | x ) f ( y 1 | x ) f ( y 2 | y 1 ) y 2 • A (general) broadcast channel is degraded if it can be split as in the figure. That is, y 2 is a “noisier” version of x , and f ( y 1 , y 2 | x ) = f ( y 2 | y 1 ) f ( y 1 | x ) Mikael Skoglund, Theoretical Foundations of Wireless 15/20 • The Gaussian broadcast (downlink) channel, y ( i ) m = x m + w ( i ) m , i = 1 , 2 • { w ( i ) m } i.i.d zero-mean Gaussian, E [ | w ( i ) m | 2 ] = σ 2 i • the channel is degraded (why?) • Coding : • Data: W 1 ∈ I 1 = { 1 , . . . , M 1 } and W 2 ∈ I 2 = { 1 , . . . , M 2 } • Encoder: α : I 1 × I 2 → C n , codewords x n 1 ( w 1 , w 2 ) • Power constraint: n 1 | x m | 2 ≤ P X n m =1 • Rates: R 1 = log M 1 /n and R 2 = log M 2 /n • Decoders: β 1 : C n → I 1 , β 2 : C n → I 2 • Error probability: P ( n ) ( ˆ W 1 , ˆ ` ´ W 2 ) � = ( W 1 , W 2 ) = Pr e Mikael Skoglund, Theoretical Foundations of Wireless 16/20
• Capacity , • achievable rate-pair : ( R 1 , R 2 ) is achievable if ( α, β 1 , β 2 ) n exist such that P ( n ) → 0 as n → ∞ e • capacity region : C = the closure of the set of all achievable ( R 1 , R 2 ) • Capacity region for the Gaussian downlink , • assume σ 1 < σ 2 ⇒ the pair „ « 1 + αP R 1 < log σ 2 1 „ 1 + (1 − α ) P « R 2 < log αP + σ 2 2 can be achieved for any α ∈ [0 , 1] Mikael Skoglund, Theoretical Foundations of Wireless 17/20 • Superposition coding achieves capacity : • Assume σ 1 < σ 2 (user 1 is the ’good’ user) • Let P 1 = αP and P 2 = (1 − α ) P • Generate two independent ’Gaussian codebooks’ C 1 and C 2 with powers P 1 and P 2 and rates R 1 and R 2 • Code w 1 into x (1) m using C 1 and w 2 into x (2) m using C 2 , transmit x m = x (1) m + x (2) m — superposition coding • β 2 assumes { x (1) m } is noise, and decodes only w 2 using C 2 • β 1 first decodes w 2 based on y (1) m and subtracts the correct x (2) m to y (1) m = y (1) m − x (2) m = x (1) m + w (1) form ¯ m , then β 1 decodes w 1 based on y (1) ¯ m • interference cancellation • works since user 1 has a better channel ⇒ must be able to order users according to their ’goodness’ Mikael Skoglund, Theoretical Foundations of Wireless 18/20
• The two-user Gaussian broadcast region (figure from the textbook). Mikael Skoglund, Theoretical Foundations of Wireless 19/20 The Fading Gaussian Downlink • Fast fading , perfect CSIR, no CSIT: • Received signal y ( i ) m = h ( i ) m x m + w ( i ) m with the h ( i ) m ’s, i = 1 , . . . , M , jointly stationary and ergodic, • general case unsolved !, the channel is non-degraded. . . • the symmetric case , when the the h ( i ) m ’s are identically distributed, i = 1 , . . . , K , the capacity region is K " 1 + | h ( i ) !# m | 2 P X R k ≤ E log σ 2 k =1 • Fast fading , perfect CSIR, perfect CSIT: Next lecture Mikael Skoglund, Theoretical Foundations of Wireless 20/20
Recommend
More recommend