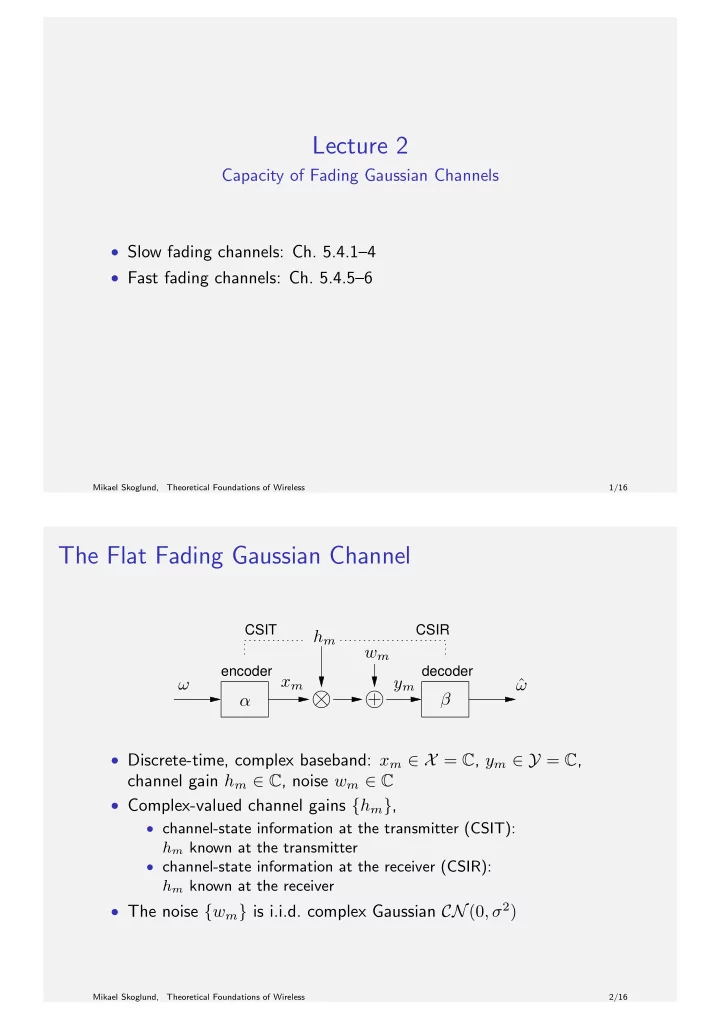
Lecture 2 Capacity of Fading Gaussian Channels Slow fading - PDF document

Lecture 2 Capacity of Fading Gaussian Channels • Slow fading channels: Ch. 5.4.1–4 • Fast fading channels: Ch. 5.4.5–6 Mikael Skoglund, Theoretical Foundations of Wireless 1/16 The Flat Fading Gaussian Channel CSIT CSIR h m w m encoder decoder x m y m ω ˆ ω β α • Discrete-time, complex baseband: x m ∈ X = C , y m ∈ Y = C , channel gain h m ∈ C , noise w m ∈ C • Complex-valued channel gains { h m } , • channel-state information at the transmitter (CSIT): h m known at the transmitter • channel-state information at the receiver (CSIR): h m known at the receiver • The noise { w m } is i.i.d. complex Gaussian CN (0 , σ 2 ) Mikael Skoglund, Theoretical Foundations of Wireless 2/16

Slow fading, perfect CSIR, no CSIT • Slow fading (non-ergodic, quasi-static, block fading): h m = h , m = 1 , . . . , n , with h drawn according to a pdf f h • Coding : • Equally likely information symbols ω ∈ I M = { 1 , . . . , M } • An ( M, n ) code with power constraint P n o x n 1 (1) , . . . , x n 1 Codebook C = 1 ( M ) , with n | x m ( i ) | 2 ≤ P, i ∈ I M n − 1 X m =1 ω = i ⇒ x n 1 = α ( i ) = x n 2 Encoding : 1 ( i ) y n ω = β ( y n 3 Decoding : 1 received, h m = h known ⇒ ˆ 1 ; h ) Mikael Skoglund, Theoretical Foundations of Wireless 3/16 • Conditional capacity , conditioned on a value h m = h � � 1 + | h | 2 P C ( h ) = log σ 2 • Outage probability , � � p out ( R ) = Pr C ( h ) < R • probability that a code of rate R will not work • Definition of ε -capacity : • The rate R is ε - achievable if there exists a sequence of ( ⌈ 2 nR ⌉ , n ) codes such that n →∞ P ( n ) lim ≤ ε e where P ( n ) = Pr(ˆ ω � = ω ) e • The ε - capacity C ε is the supremum of the ε -achievable rates Mikael Skoglund, Theoretical Foundations of Wireless 4/16

• Let γ = | h | 2 , F γ ( x ) = Pr( γ ≤ x ) • ε -capacity (’ ε -outage capacity’) of the slow fading channel , γ ( ε ) P � 1 + F − 1 � C ε = log σ 2 • Since, when trying a ’Gaussian codebook’ of rate R , ( C ( h ) > R ⇒ P ( n ) → 0 e P ( n ) ⇒ → p out ( R ) e C ( h ) < R ⇒ P ( n ) → 1 e • F γ such that F γ ( x ) > 0 for any x > 0 ⇒ C = lim ε → 0 + C ε = 0 • The ’ordinary’ (Shannon) capacity C is zero! • true e.g. for exponential γ (Rayleigh fading) Mikael Skoglund, Theoretical Foundations of Wireless 5/16 Parallel slow fading channels, perfect CSIR, no CSIT • General block fading model : Assume n = LT c and h m = g ℓ , m = t + ( ℓ − 1) T c , ℓ = 1 , . . . , L , t = 1 , . . . T c and { g ℓ } i.i.d, • the channel is constant for T c channel uses = the “coherence interval,” i.i.d realizations in different intervals • Coding : • Block length n = LT c — coding over L coherence intervals, n o x n 1 (1) , . . . , x n • Codebook : C = 1 ( M ) , power constraint P ω = i ⇒ x n 1 = α ( i ) = x n • Encoding : 1 ( i ) y n 1 received, h n ω = β ( y n 1 ; h n • Decoding : 1 known ⇒ ˆ 1 ) Mikael Skoglund, Theoretical Foundations of Wireless 6/16

• ε -capacity , the general block fading model: • R = n − 1 log M is ε -achievable if there exists a sequence of ( ⌈ 2 nR ⌉ , n ) codes such that lim P ( n ) ≤ ε e when T c → ∞ for a fixed and finite L , with n = LT c • C ε is the supremum of the ε -achievable rates Mikael Skoglund, Theoretical Foundations of Wireless 7/16 • With L „ « 1 ) = 1 1 + | g ℓ | 2 P X C ( g L log σ 2 L ℓ =1 and C ( g L ` ´ p out ( R ) = Pr 1 ) < R it can be shown that C ε = p − 1 out ( ε ) • p out decays as ( P/σ 2 ) − L ⇒ L -fold diversity! • the transmitter does not need to know { g ℓ } • coding needs to span L different coherence intervals ⇒ long delays Mikael Skoglund, Theoretical Foundations of Wireless 8/16

Fast fading, perfect CSIR, no CSIT • Fast fading (ergodic fading): Assume { h m } is an i.i.d process (or more generally, stationary and ergodic), • each time-instant m gives a new value for h m • Coding : n o x n 1 (1) , . . . , x n • Codebook : C = 1 ( M ) , power constraint P ω = i ⇒ x n 1 = α ( i ) = x n • Encoding : 1 ( i ) y n 1 received, h n ω = β ( y n 1 ; h n • Decoding : 1 known ⇒ ˆ 1 ) Mikael Skoglund, Theoretical Foundations of Wireless 9/16 • Capacity (ergodic capacity), � � �� 1 + | h m | 2 P C = E log σ 2 • { h m } stationary ⇒ C does not depend on m • no CSIT required • This is the same value as obtained for C ε , any ε ∈ (0 , 1) , in the block fading channel model when letting L → ∞ , • coding accross (infinitely) many coherence intervals necessary ⇒ long delays Mikael Skoglund, Theoretical Foundations of Wireless 10/16

Fast fading, perfect CSIR, perfect CSIT • { h m } known causally at the transmitter • Coding : ω = i and h m 1 known ⇒ x m = α ( i ; h m • Encoding : 1 ) , with power constraint n 1 ) | 2 ≤ P, i ∈ I M n − 1 X E | x m ( i ; h m m =1 y n 1 received, h n ω = β ( y n 1 ; h n • Decoding : 1 known ⇒ ˆ 1 ) Mikael Skoglund, Theoretical Foundations of Wireless 11/16 • Capacity (ergodic capacity), � � �� 1 + | h m | 2 P ( h m ) C = E log σ 2 where � 1 � + λ − σ 2 P ( x ) = | x | 2 and where λ is chosen such that E [ P ( h m )] = P • Waterfilling over time . . . Mikael Skoglund, Theoretical Foundations of Wireless 12/16

• Separate power control is optimal z m x m ω π m ‘Gaussian codebook’ h m π ( · ) • Fixed (rate and power) ’Gaussian codebook’ { z n 1 ( i ) } , with n 1 | z m | 2 ≤ 1 X n m =1 • Based on the CSIT h m , multiply with π m = √ P m where » 1 – + σ 2 P m = λ − | h m | 2 and transmit x m = π m z m — achieves capacity Mikael Skoglund, Theoretical Foundations of Wireless 13/16 • Optimal power control, • Notable gains only at low SNR’s. . . Mikael Skoglund, Theoretical Foundations of Wireless 14/16

• Channel inversion : • Assume real-valued transmission: y m = h m ( π m z m ) + w m • Use λ π m = h m with λ chosen such that E [ π 2 m ] = P ⇒ y m = λz m + w m ⇒ 1 + λ 2 C = 1 ` ´ 2 log σ 2 • Simple, but suboptimal in general • Can give huge power peaks (when h m ≈ 0 ) • For e.g. h m Rayleigh distributed, E [ h − 2 m ] = ∞ ⇒ inversion does not work Mikael Skoglund, Theoretical Foundations of Wireless 15/16 • Capacities, fast fading; plot from • Goldsmith and Varaiya, “Capacity of fading channels with channel side information,” IEEE Trans. on Inform. Theory , Nov. 1997 Mikael Skoglund, Theoretical Foundations of Wireless 16/16