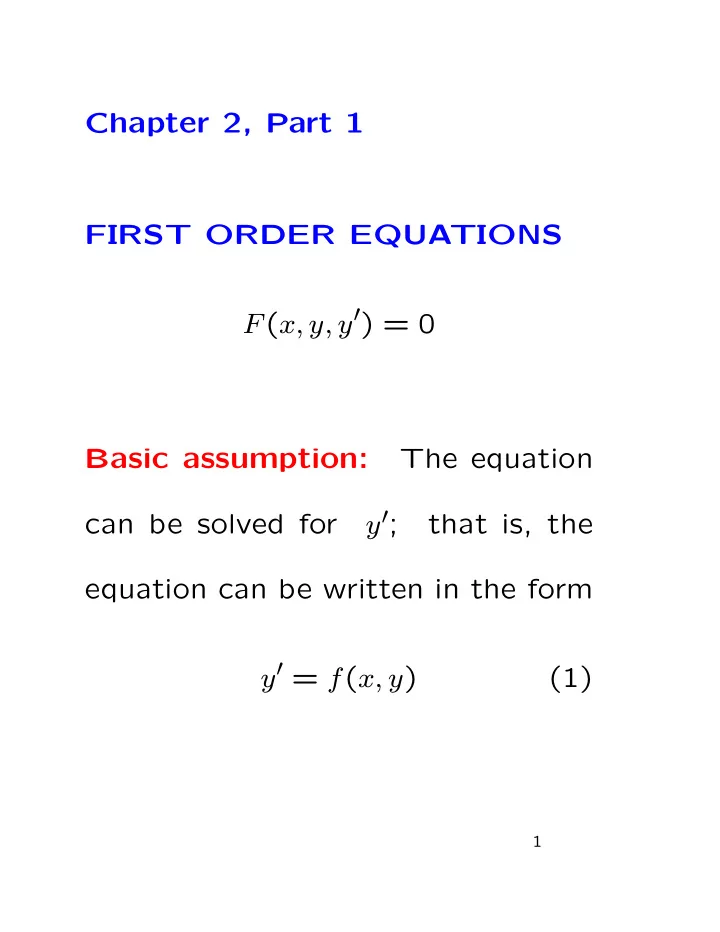
Chapter 2, Part 1 FIRST ORDER EQUATIONS F ( x, y, y ) = 0 The - PDF document

Chapter 2, Part 1 FIRST ORDER EQUATIONS F ( x, y, y ′ ) = 0 The equation Basic assumption: y ′ ; can be solved for that is, the equation can be written in the form y ′ = f ( x, y ) (1) 1

Assumed Background Material: Techniques of integration, including: • Substitution (the most common technique) • Integration-by-parts • Integrals involving trig functions • Partial fraction decomposition 2

2.2. First Order Linear Equa- tions Equation (1) is a linear equation if f has the form f ( x, y ) = P ( x ) y + q ( x ) where and are continuous P q functions on some interval I . Thus y ′ = P ( x ) y + q ( x ) 3

Standard form: The standard form for a first order linear equation is: y ′ + p ( x ) y = q ( x ) where p and q are continuous functions on the interval I ( Note: A differential equation which is not linear is called nonlinear .) 4

Examples: Find the general solution: 1. y ′ = 3 y 5

Find the general solution: 2. y ′ + 2 xy = 4 x 6

Solution Method: Determine that the equa- Step 1. tion is linear and write it in standard form y ′ + p ( x ) y = q ( x ) . 7

y ′ + p ( x ) y = q ( x ) . � p ( x ) dx : Multiply by e Step 2. 8

� p ( x ) dx y � p ( x ) dx � ′ = q ( x ) e � e Integrate: Step 3. � p ( x ) dx y = � p ( x ) dx dx + C. � e q ( x ) e Solve for y : Step 4. � p ( x ) dx � p ( t ) dt dx + Ce − � p ( x ) dx . � y = e − q ( t ) e 9

� p ( x ) dx � p ( x ) dx dx + Ce − � p ( x ) dx . � y = e − q ( x ) e is the general solution of the equa- tion. � p ( x ) dx is called an inte- e Note: grating factor 10

Find the general solution: 3. xy ′ = ln x x 2 − 3 y 11

Find the general solution: 4. 2 xy ′ = − 2 y + 2 � x 2 − 1 12

Solve the initial-value problem: 5. y ′ +(cot x ) y = 2 cos x, y ( π/ 2) = 3 13

Find the general solution: 6. y ′ + 2 xy = 2 tan x Answer: 2 e x 2 tan x dx + Ce − x 2 y = e − x 2 � 14

Answers: y = Ce 3 x 1. y = 2 + Ce − x 2 2. y = ln x x 2 − 1 x 2 + C 3. x 3 � x 2 − 1 y = 2 + 1 + C 4. x 2 x 2 y = 5 − cos 2 x 5. 2 sin x 15

The term “linear:” Differentiation: As you know: for differentiable func- tions f and g dx [ f ( x ) + g ( x )] = d d dx + dg f dx and for any constant c dx [ c f ( x )] = c d d f dx 16

Integration: For integrable functions f and g : � � � [ f ( x ) + g ( x )] dx = f ( x ) dx + g ( x ) dx and, for any constant c � � c f ( x ) dx = c f ( x ) dx 17

Any “operation” L which satisfies L [ f ( x ) + g ( x )] = L [ f ( x )] + L [ g ( x )] and L [ c f ( x )] = c L [ f ( x )] is a “linear” operation. 1. Differentiation is a linear oper- ation. 2. Integration is a linear operation. 18

L [ y ] = y ′ + p ( x ) y Set L [ y 1 + y 2 ] = ( y 1 + y 2 ) ′ + p ( y 1 + y 2 ) = y ′ 1 + y ′ 2 + py 1 + py 2 = y ′ 1 + py 1 + y ′ 2 + py 2 = L [ y 1 ]+ L [ y 2 ] L [ cy ] = ( cy ) ′ + p ( cy ) = cy ′ + cpy = c ( y ′ + py ) = cL [ y ] 19

L [ y ] = y ′ + p ( x ) y , Thus, if then L [ y 1 + y 2 ] = L [ y 1 ] + L [ y 2 ] L [ c y ] = c L [ y ] L [ y ] = y ′ + p ( x ) y is a linear opera- tion; L is a linear operator. Hence the term linear differential equa- tion. 20

2.3. Separable Equations y ′ = f ( x, y ) is a separable equation if f has the factored form f ( x, y ) = p ( x ) h ( y ) where p and h are continuous functions. Thus y ′ = p ( x ) h ( y ) is the ”standard form” of a separa- ble equation. 21

Example: Find the general solution of: y ′ = 2 x + 2 xy 2 22

Solution Method Establish that the equa- Step 1. tion is separable. Divide both sides by h ( y ) Step 2. to “separate” the variables. 1 h ( y ) y ′ = p ( x ) q ( y ) y ′ = p ( x ) or which, in differential form, is: q ( y ) dy = p ( x ) dx. the variables are “separated.” 23

Integrate Step 3. � � q ( y ) dy = p ( x ) dx + C Q ( y ) = P ( x ) + C Q ′ ( y ) = q ( y ) , P ′ ( x ) = p ( x ) where 24

Note: Q ( y ) = P ( x ) + C is the general so- lution. Typically, this is an implicit relation; you may or may not be able to solve it for y . 25

Examples: Find the general solution: 1. y ′ = xy 2 + 4 x 2 y 26

Find the general solution: 2. dy � dx = 4 x y − 2 27

Singular Solutions dy � dx = 4 x y − 2 28

10 8 6 4 2 0 - 3 - 2 - 1 0 1 2 3 29

Find the general solution: 3. dy dx − xy 2 = − x 30

Find the general solution: 4. e x − y dy dx = 1 + e x 31

The equation 5. y ′ = x ( y +2) y ′ − xy = 2 x or is both linear and separable. Find the general solution both ways. 32

Answers y = tan( x 2 + C ) 1. √ y − 2 = x 2 + C 2. y = 1 + Ce x 2 3. 1 − Ce x 2 y = ln [ln(1 + e x ) + C ] 4. y = Ce x 2 / 2 − 2 5. 33

2.4. Related Equations & Trans- formations A. Bernoulli equations An equation of the form y ′ + p ( x ) y = q ( x ) y k , k � = 0 , 1 is called a Bernoulli equation . 34

The change of variable v = y 1 − k transforms a Bernoulli equation into v ′ + (1 − k ) p ( x ) v = (1 − k ) q ( x ) . which has the form v ′ + P ( x ) v = Q ( x ) , a linear equation. 35

Examples: Find the general solution: 1. y ′ − 4 y = 2 e x √ y 36

Find the general solution: 2. xy ′ + y = 3 x 3 y 2 37

Find the general solution: 3. xyy ′ = x 2 + 2 y 2 38

Answers Ce 2 x − e x � 2 � y = 1. 2 y = 2. Cx − 3 x 3 y 2 = Cx 4 − x 2 3. 39

B. Homogeneous equations y ′ = f ( x, y ) (1) is a homogeneous equation if f ( tx, ty ) = f ( x, y ) 40

If (1) is homogeneous, then the change of dependent variable y ′ = v + xv ′ y = vx, transforms (1) into a separable equa- tion: y ′ = f ( x, y ) → v + xv ′ = f ( x, vx ) = f (1 , v ) which can be written f (1 , v ) − v dv = 1 1 x dx ; the variables are separated. 41

Examples: Find the general solution: 1. y ′ = x 2 + y 2 2 xy 42

Find the general solution: 2. dx = x 2 e y/x + y 2 dy xy 43

Find the general solution: 3. xyy ′ = x 2 + 2 y 2 44

Answers y 2 = x 2 − Cx 1. y + x = e y/x [ Cx − x ln x ] 2. y 2 = Cx 4 − x 2 3. 45