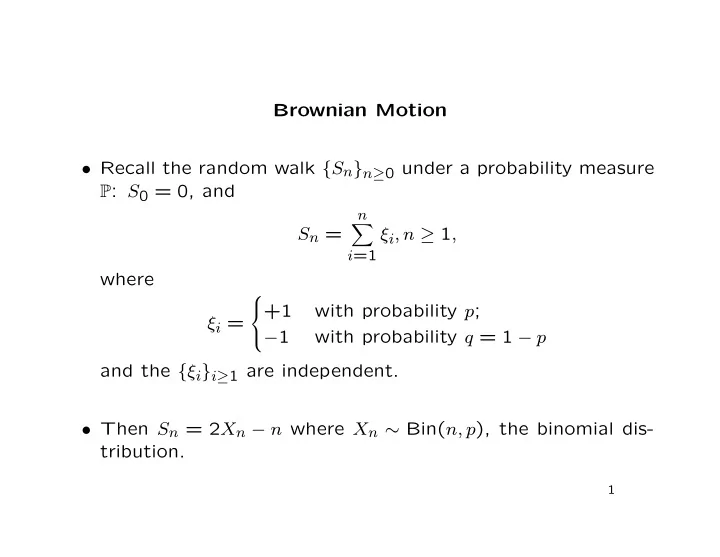
Brownian Motion Recall the random walk { S n } n 0 under a - PowerPoint PPT Presentation

Brownian Motion • Recall the random walk { S n } n ≥ 0 under a probability measure P : S 0 = 0, and n � S n = ξ i , n ≥ 1 , i =1 where  +1 with probability p ;  ξ i = − 1 with probability q = 1 − p  and the { ξ i } i ≥ 1 are independent. • Then S n = 2 X n − n where X n ∼ Bin( n, p ), the binomial dis- tribution. 1

• Results from the binomial distribution show that p − 1 � � E [ S n ] = n ( p − q ) = 2 n 2 and var( S n ) = 4 npq. • In the symmetric case, p = q = 1 2 , and S 2 � � E [ S n ] = 0 , var( S n ) = E = n. n • More generally, cov( S m , S n ) = m ∧ n. 2

• In fact, { S n } n ≥ 0 is a P -martingale (with respect to the natural filtration). • If 0 ≤ i ≤ j ≤ k ≤ l , then j � S j − S i = ξ m m = i +1 and l � S l − S k = ξ m m = k +1 are independent. 3

• Also, if j − i = l − k = m , say, then S j − S i and S l − S k are both sums of m ξ ’s, and hence both have the same distribution as S m . • So, under P , { S n } n ≥ 0 has stationary and independent incre- ments . 4

• Now for t ∈ R , t > 0, write S ⌊ nt ⌋ X ( n ) = √ n , t where ⌊ nt ⌋ is the integer part of nt . � � X ( n ) • Then E = 0, and t = 1 � � X ( n ) , X ( n ) cov n ⌊ ns ⌋ ∧ ⌊ nt ⌋ ≈ s ∧ t. s t • By the Central Limit Theorem, if n is large then X ( n ) is t approximately N (0 , t ). 5

• Brownian motion is defined as the stochastic process with these limiting characteristics. • Specifically, a real-valued stochastic process { W t } t ≥ 0 is a P - Brownian motion (or a P -Wiener process) if for some σ > 0: – for each s ≥ 0 and t > 0, W s + t − W s ∼ N (0 , σ 2 t ); – for each n ≥ 1 and times 0 ≤ t 0 ≤ · · · ≤ t n , the increments { W t r − W t r − 1 } are independent; – W 0 = 0; – W t is continuous in t ≥ 0. 6

• If σ = 1, { W t } t ≥ 0 is standard Brownian motion. • Notes: – continuity (with P -probability 1) is in fact a consequence of the first two parts of the definition. – the first two parts mean that { W t } t ≥ 0 , like the random walk, has stationary and independent increments. 7

• A process with stationary and independent increments is called a L´ evy process. – Brownian motion is the L´ evy process with Gaussian (nor- mally distributed) increments. – Brownian motion is the only L´ evy process with almost surely continuous sample paths. – Another L´ evy process is the counting process { N t } t ≥ 0 of a Poisson point process. 8

• Other properties of Brownian motion: – Although almost surely continuous, it is almost surely nowhere differentiable; – Brownian motion will, with P -probability 1, eventually reach any real value, no matter how large, and will return to 0, also with P -probability 1; – Once Brownian motion hits a value, it immediately hits again infinitely often; – Brownian motion is self-similar . 9