1
play

1 Static Equilibrium From Static Eq. to Dynamic Eq. System of mass - PDF document

Apr 22, 2023 •383 likes •426 views

Static/ Dynamic Deformation I ntroduction to Static deformation Dynamic deformation Static/ Dynamic Deformation with Mass-Spring Systems undeformed shape f internal + = f inertia = 0 f external Min Gyu Choi deformed

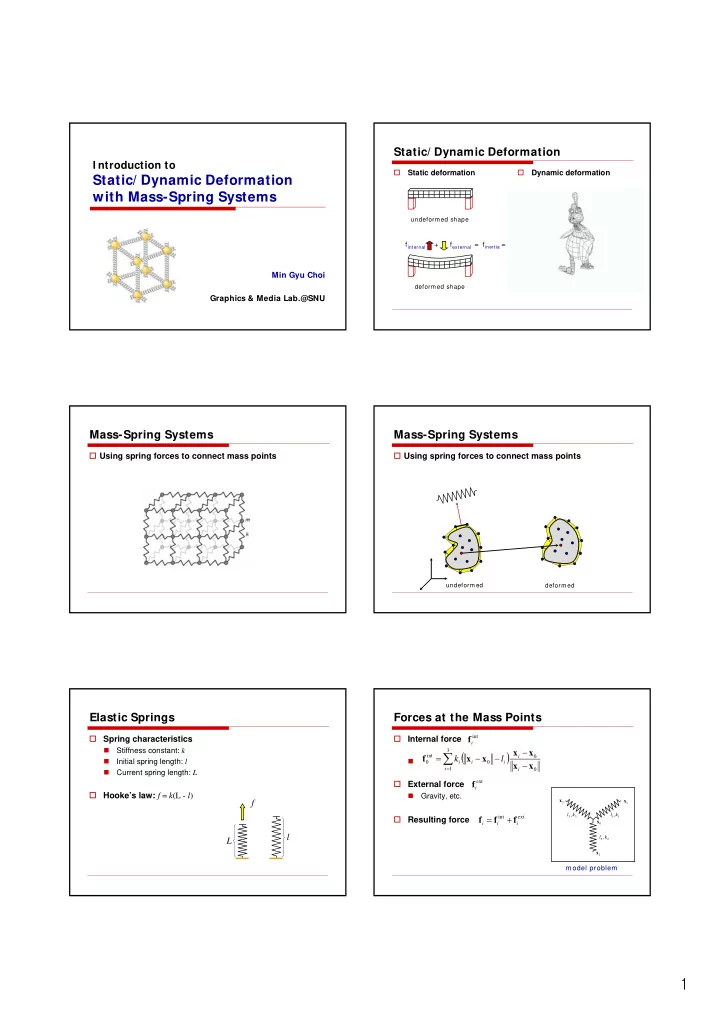
  1. Static/ Dynamic Deformation I ntroduction to � � Static deformation Dynamic deformation Static/ Dynamic Deformation with Mass-Spring Systems undeformed shape f internal + = f inertia = 0 f external Min Gyu Choi deformed shape Graphics & Media Lab.@SNU Mass-Spring Systems Mass-Spring Systems � Using spring forces to connect mass points � Using spring forces to connect mass points undeformed deformed Elastic Springs Forces at the Mass Points � Spring characteristics � Internal force f int i � − Stiffness constant: k ( ) x x 3 ∑ = − − f int x x i 0 k l � � Initial spring length: l − 0 i i 0 i x x = i 1 � i 0 Current spring length: L f � External force ext i � Hooke’s law: f = k (L - l ) � Gravity, etc. x x f 3 1 l 3 , k l 1 , k = + � Resulting force f f int f ext 3 1 x i i i 0 l l 2 , k L 2 x 2 model problem 1

  2. Static Equilibrium From Static Eq. to Dynamic Eq. � System of mass points � Static equilibrium ext = ( ) ext = = + f K x f = + f K x f = ( ) 0 ( t ) ( t ) 0 f int K x ( ) � Equation of motion x x 3 1 � Static equilibrium 2 x d ( t ) l 3 , k l 1 , k = M f 3 1 ( t ) x 2 ext = 0 dt = + f K x f ( ) 0 l 2 , k 2 x 2 model problem From Static Eq. to Dynamic Eq. Mass-Spring Dynamics � Static equilibrium � Equation of motion for mass point i at time t ext = ext = ( ) = + f K x f f = K x + f ( ) 0 ( t ) ( t ) 0 mass position damping coefficient 2 x x d ( t ) d ( t ) + = f m i c i ( t ) � Equation of motion with damping i 2 i i dt dt acceleration damping spring + external 2 x x d ( t ) d ( t ) M + C = f ( t ) 2 2 nd order differential equation dt dt � � f is used for acceleration and damping � Without damping term, simply f = ma Numerical I ntegration Damping Revisited � 2 nd order ODE ⇒ two coupled 1 st order ODEs � Point damping − v f v x x 2 d ( t ) ( t ) c ( t ) d ( t ) d ( t ) = + = f i i i i i i m c ( t ) i i i 2 dt m dt dt i � Damping force in opposite direction to the velocity � Damped spring ⎧ x d ( t ) = v i velocity ( t ) ⎪ ⎪ i dt ⎨ − v f v d ( t ) ( t ) c ( t ) ⎪ = i i i i acceleration ⎪ ⎩ dt m � Damping force proportional to the velocity of the spring i 2

  3. Topology and Stability � Stability with respect to deformation Finite Element Method with Lumped Mass Formulation stable but not general not stable much more resistant in direction than in Min Gyu Choi Requires well-chosen stable topologies! Graphics & Media Lab.@SNU Mass-Spring Systems vs. FEM Mass-Tensor Systems � � � � Mass-spring dynamics Elasto-dynamic equations Mass-spring dynamics Elasto-dynamic equations x x x x 2 2 d ( t ) d ( t ) d ( t ) d ( t ) + = + = f + + = f + + = m i c i ( t ) M u & & C u & Ku f m i c i ( t ) M & u & C & u Ku f i i i i i i 2 2 dt dt dt dt � � � � Explicit integration Explicit integration Explicit integration Explicit integration ∆ = + ∆ ∆ = + ∆ x x & & x ( ) x & x x & ( ) h ( ) h ( ) ∆ = + ∆ ∆ = + ∆ u u & u & u u & u & i i i h i i i h ( ) ( ) h ( ) h ( ) ∆ = − M ∆ u & = f − C u & − Ku ∆ = − M ∆ & u = f − C u & − Ku x & f & x h x & f x & h c c i i i i i i i i m m i i stable topology matrix inversion appropriate constants small displacements Providing force from strain energy rather than spring energy! Lumped Mass Formulation Lumped Mass Formulation � � � � Mass-spring dynamics Elasto-dynamic equations Mass-spring dynamics Elasto-dynamic equations 2 x x 2 x x d ( t ) d ( t ) d ( t ) d ( t ) + = + = i i f + + = i i f + + = m c ( t ) M u & & C u & Ku f m c ( t ) M u & & C & u Ku f i i i i i i 2 2 dt dt dt dt � � � � Explicit integration Explicit integration Explicit integration Explicit integration ∆ = + ∆ ∆ = + ∆ x x & x & ( ) x & x x & ( ) h ( ) h ( ) ∆ u = u + ∆ u ∆ u = u + ∆ u & & & & i i i h i i i h ( ) ( ) h ( ) h ( ) ∆ = − − ∆ = − − ∆ = − M u & f C u & Ku ∆ = − M u & f C u & Ku & x f x & h & x f x & h c c i i i i i i i i m m i i Mass Lum ping ≅ ( ) ( ) ∆ u = u + ∆ u ∆ = + ∆ h & & u u & u & h ( ) ( ) ∆ = − − − D ∆ u = f − C u − Ku u & D 1 f C u & Ku & h & h mass lumping 3 × m m 3 3

  4. Mass-Spring vs. Lumped Mass FEM Visco-Elastic Formulation � � � Green’s strain tensor Mass-spring dynamics Elasto-dynamic equations 2 x x ∂ ∂ d ( t ) d ( t ) x x + = f i i M u + C u + Ku = f ε = ⋅ − δ m c ( t ) & & & = − ε J T J I i 2 i i dt dt ∂ ∂ ij ij u u [ ] i j = ∂ ∂ J x u Large displacements Large displacements i j 3 × 3 are supported inherently. ∂ ∂ x x ε = ⋅ − δ ∂ ∂ ij ij u u i j Damped spring Strain rate tensor ∂ ∂ ∂ ∂ x x & x & x ν = ⋅ + ⋅ x ( u ∂ ∂ ∂ ∂ ) ij u u u u u i j i j Adopt nonlinear visco-elastic formulation! Visco-Elastic Formulation Visco-Elastic Formulation � Green’s strain tensor � Stress tensor ∂ x ∂ x ε = λ + µ ν = φ + ψ σ ε I ε σ ν I ν ε = ⋅ − δ = − tr( ) 2 tr( ) 2 ε J T J I ∂ ∂ ij ij u u [ ] i j = ∂ ∂ J x u elastic stress tensor viscous stress tensor i j 3 × 3 � Strain rate tensor � Strain energy [ ] & J = ∂ ∂ & x u i j 3 × 3 ε ∂ ∂ ∂ ∂ x x x x 1 1 d & & T + ε ∫ σ ε ν ∫ σ ν = ij ε Ω = ij ν Ω & & ν = ij = ⋅ + ⋅ = U d U d ν J J J T J ij ij ij ∂ ∂ ∂ ∂ 2 2 dt u u u u Ω Ω i j i j elastic potential energy damping potential energy Finite Element Discretization � Refer to the paper James F. O’Brien and Jessica K. Hogdins Graphical Modeling and Animation of Brittle Fracture Computer Graphics ( Proc . SIGGRAPH ’99), pp. 137-146 4

Recommend

More recommend