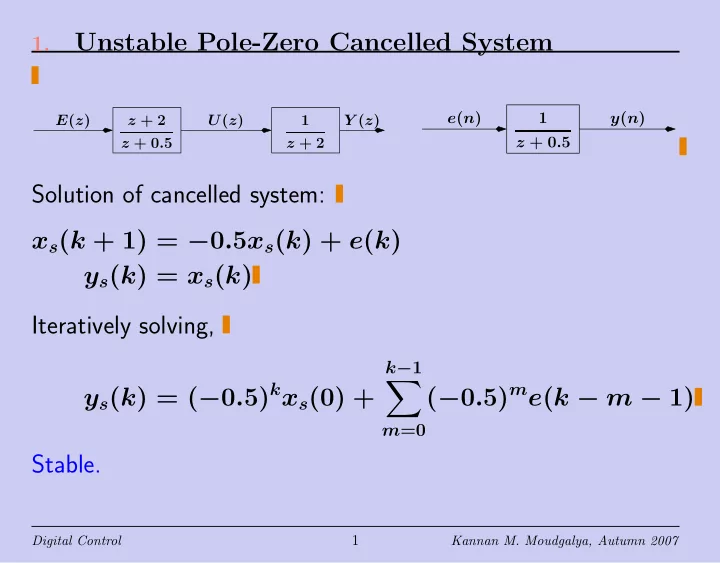
Unstable Pole-Zero Cancelled System 1. e ( n ) 1 y ( n ) E ( z ) z - PowerPoint PPT Presentation

Unstable Pole-Zero Cancelled System 1. e ( n ) 1 y ( n ) E ( z ) z + 2 U ( z ) 1 Y ( z ) z + 0 . 5 z + 0 . 5 z + 2 Solution of cancelled system: x s ( k + 1) = − 0 . 5 x s ( k ) + e ( k ) y s ( k ) = x s ( k ) Iteratively solving, k − 1 � y s ( k ) = ( − 0 . 5) k x s (0) + ( − 0 . 5) m e ( k − m − 1) m =0 Stable. 1 Digital Control Kannan M. Moudgalya, Autumn 2007

System without Pole-Zero Cancellation 2. E ( z ) z + 2 U ( z ) 1 Y ( z ) z + 0 . 5 z + 2 Can verify for first block: First block: x 1 ( k + 1) = − 0 . 5 x 1 ( k ) + 1 . 5 e ( k ) u ( k ) = x 1 ( k ) + e ( k ) Second block: x 2 ( k + 1) = − 2 x 2 ( k ) + u ( k ) y ( k ) = x 2 ( k ) x 2 ( k + 1) = − 2 x 2 ( k ) + x 1 ( k ) + e ( k ) Substituting, � x 1 ( k + 1) � � − 0 . 5 � � x 1 ( k ) � � 1 . 5 � 0 = + e ( k ) x 2 ( k + 1) 1 − 2 x 2 ( k ) 1 � � x 1 ( k ) � � y ( k ) = 0 1 x 2 ( k ) 2 Digital Control Kannan M. Moudgalya, Autumn 2007

System without Pole-Zero Cancellation 3. E ( z ) z + 2 U ( z ) 1 Y ( z ) z + 0 . 5 z + 2 � x 1 ( k + 1) � � − 0 . 5 � � x 1 ( k ) � � 1 . 5 � 0 = + e ( k ) x 2 ( k + 1) 1 − 2 x 2 ( k ) 1 � � x 1 ( k ) � � 0 1 y ( k ) = x 2 ( k ) k − 1 � = CA k x (0) + CA m be ( k − m − 1) m =0 Can show that this is equal to k − 1 3 ( − 0 . 5) k ( − 2) k � � � x 1 (0) � ( − 0 . 5) m e ( k − m − 1) � + − x 1 (0) + 1 . 5 x 2 (0) 2 m =0 x (0) � = 0 , results in y ( k ) being unbounded! Whence − 2 ? 3 Digital Control Kannan M. Moudgalya, Autumn 2007

Diagonalization 4. A : square matrix λ j : j th eigenvalue x j : j th eigenvector Ax 1 = λ 1 x 1 . . . Ax n = λ n x n Stacking these side by side,       | | | | 0 λ 1 ...  = x 1 · · · x n x 1 · · · x n A      | | | | 0 λ n AS = S Λ Assume the eigenvectors to be independent ⇒ S − 1 exists A = S Λ S − 1 4 Digital Control Kannan M. Moudgalya, Autumn 2007

Diagonalization 5. A = S Λ S − 1 A 2 = S Λ S − 1 S Λ S − 1 = S Λ 2 S − 1 A 3 = S Λ 2 S − 1 S Λ S − 1 = S Λ 3 S − 1 . . . A k = S Λ k S − 1 Easy to evaluate RHS: k λ k     λ 1 0 0 1 Λ k = ... ... =     λ k 0 λ n 0 n This approach is used to arrive at the solution. 5 Digital Control Kannan M. Moudgalya, Autumn 2007

Condition for Cancellation of Poles and Zeros 6. E ( z ) z + 2 U ( z ) 1 Y ( z ) z + 0 . 5 z + 2 Solution of system after cancellation: k − 1 � y s ( k ) = ( − 0 . 5) m x s (0) + ( − 0 . 5) m e ( k − m − 1) m =0 Solution of system if there is no cancellation: k − 1 3 ( − 0 . 5) k ( − 2) k � � � x 1 (0) � ( − 0 . 5) m e ( k − m − 1) � + − x 1 (0) + 1 . 5 x 2 (0) 2 m =0 Two solutions are identical only if • x 1 (0) = 1 . 5 x 2 (0) or • x 1 (0) = x 2 (0) = 0 . 6 Digital Control Kannan M. Moudgalya, Autumn 2007

Aryabhatta’s Identity 7. We need to solve the polynomial equation X ( z ) D ( z ) + Y ( z ) N ( z ) = C ( z ) for X and Y , with D , N and C specified. • Has a solution iff the GCD of D ( z ) and N ( z ) divides C ( z ) • There are infinitely many solutions to Aryabhatta’s identity • Unique solution under special conditions. Suppose D and N are coprime with degrees d D > 0 and d N > 0 . If 0 ≤ d C < d D + d N there is a unique least degree solution given by d X ( z ) < d N ( z ) d Y ( z ) < d D ( z ) 7 Digital Control Kannan M. Moudgalya, Autumn 2007

Algorithm for Aryabhatta’s Identity 8. X ( z ) D ( z ) + Y ( z ) N ( z ) = C ( z ) is the same as � � D ( z ) � � X ( z ) Y ( z ) = C ( z ) N ( z ) Solved by comparing the coefficients of powers of z − 1 . Same as V ( z ) F ( z ) = C ( z ) Can be written as [ V 0 + V 1 z − 1 + · · · + V v z − v ][ F 0 + F 1 z − 1 + · · · + F d F z − d F ] = C 0 + C 1 z − 1 + · · · + C d C z − d C v is an unknown. All V i are also unknowns. 8 Digital Control Kannan M. Moudgalya, Autumn 2007

Algorithm for Aryabhatta’s Identity 9. [ V 0 + V 1 z − 1 + · · · + V v z − v ][ F 0 + F 1 z − 1 + · · · + F d F z − d F ] = C 0 + C 1 z − 1 + · · · + C d C z − d C is the same as   F 0 F 1 · · · F d F 0 · · · 0 0 · · · F d F · · · 0 F 0 F 1 [ V 0 V 1 · · · V v ] .   . .   0 · · · 0 · · · F d F F 0 F 1 = [ C 0 C 1 · · · C d C ] with maximal v . Ensures all combination of products are used. Multiplying, first two equations are obtained as V 0 F 0 = C 0 , V 0 F 1 + V 1 F 0 = C 1 Matrix equation is written as V F = C 9 Digital Control Kannan M. Moudgalya, Autumn 2007

Algorithm for Aryabhatta’s Identity 10. Recall from the previous slide: [ V 0 + V 1 z − 1 + · · · + V v z − v ][ F 0 + F 1 z − 1 + · · · + F d F z − d F ] = C 0 + C 1 z − 1 + · · · + C d C z − d C Can be written as, V F = C IF F is right invertible, the solution is, V = C F − 1 • For this, rows of F have to be linearly independent. • Choose v as the largest integer, satisfying this requirement. • From V , V ( z ) and then X ( z ) , Y ( z ) can be determined, � � because, V = X Y 10 Digital Control Kannan M. Moudgalya, Autumn 2007

Summary: Algorithm for Aryabhatta’s Identity 11. X ( z ) D ( z ) + Y ( z ) N ( z ) = C ( z ) � � D ( z ) � � X ( z ) Y ( z ) = C ( z ) N ( z ) V ( z ) F ( z ) = C ( z ) Can be written as   F 0 F 1 · · · F d F 0 · · · 0 0 F 0 F 1 · · · F d F · · · 0   � � V 0 V 1 · · · V v . .   .   0 · · · 0 · · · F d F F 0 F 1 � � C 0 C 1 · · · C d C = Write this as V F = C , solve for V as V = C F − 1 11 Digital Control Kannan M. Moudgalya, Autumn 2007

Example: Algorithm for Aryabhatta’s Identity 12. Solve for X ( z ) and Y ( z ) satisfying X ( z ) D ( z ) + Y ( z ) N ( z ) = C ( z ) with D ( z ) = 1 − 5 z − 1 + 4 z − 2 N ( z ) = z − 1 + z − 2 C ( z ) = 1 − z − 1 + 0 . 5 z − 2 Construct F : � 1 − 5 z − 1 + 4 z − 2 � � 1 � � − 5 � � 4 � z − 1 + z − 2 F ( z ) = = + z − 1 + z − 2 0 1 1 which is of the form F 0 + F 1 z − 1 + F 2 z − 2 . 12 Digital Control Kannan M. Moudgalya, Autumn 2007

Example: Algorithm for Aryabhatta’s Identity 13. Recall � 1 − 5 z − 1 + 4 z − 2 � � 1 � � − 5 � � 4 � z − 1 + z − 2 F ( z ) = = + z − 1 + z − 2 0 1 1 which is of the form F 0 + F 1 z − 1 + F 2 z − 2 . Explore v = 1 : � 1 − 5 4 � � � F = = F 0 F 1 F 2 0 1 1 Although independent, v is not the largest. Explore v = 2 :   1 − 5 4 0 � F 0 F 1 F 2 0 � 0 1 1 0   F = =   0 F 0 F 1 F 2 0 1 − 5 4   0 0 1 1 These rows are independent. Hence v is still not the maximum. 13 Digital Control Kannan M. Moudgalya, Autumn 2007

Example: Algorithm for Aryabhatta’s Identity 14. Explore the possibility of v = 3 :   1 − 5 4 0 0 0 1 1 0 0     F 0 F 1 F 2 0 0   0 1 − 5 4 0  =   F = 0 F 0 F 1 F 2 0    0 0 1 1 0   0 0 F 0 F 1 F 2   0 0 1 − 5 4   0 0 0 1 1 No longer independent. Remove one row and solve: − 1   1 − 5 4 0 0 0 1 1 0 0   � �   1 − 1 0 . 5 0 0 0 1 − 5 4 0 V =     0 0 1 1 0   0 0 1 − 5 4 14 Digital Control Kannan M. Moudgalya, Autumn 2007

Example: Algorithm for Aryabhatta’s Identity 15. • V is a 1 × 5 vector. • To account for the row removed from F , we add a zero to V . We obtain � � V = 1 3 . 25 | 0 . 75 − 3 | 0 0 where we have separated coefficients of powers of z − 1 with vertical lines. � � � � 1 3 . 25 0 . 75 − 3 • We have V 0 = and V 1 = . • We obtain X ( z ) = 1 + 0 . 75 z − 1 Y ( z ) = 3 . 25 − 3 z − 1 These form the solution to Aryabhatta’s identity 15 Digital Control Kannan M. Moudgalya, Autumn 2007