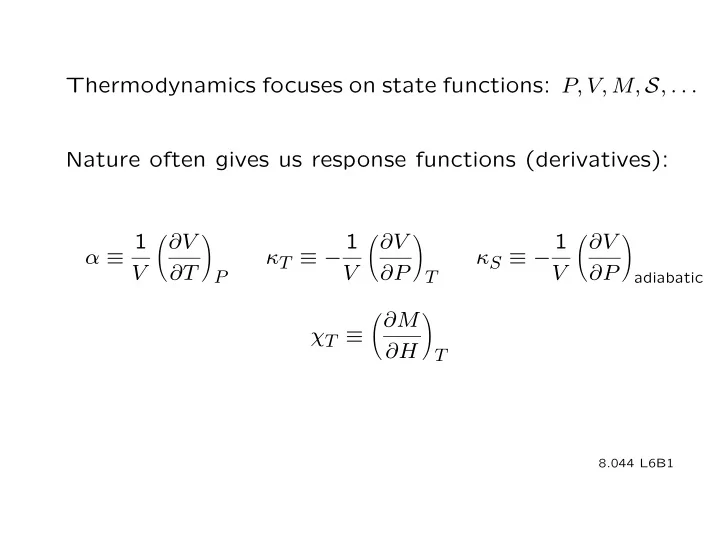
Thermodynamics focuses on state functions: P, V, M, S , . . . Nature - PowerPoint PPT Presentation

Thermodynamics focuses on state functions: P, V, M, S , . . . Nature often gives us response functions (derivatives): 1 1 1 � ∂V � ∂V � ∂V � � � α ≡ V κ T ≡ − κ S ≡ − ∂T P V ∂P T V ∂P adiabatic ∂M � � χ T ≡ ∂H T 8.044 L6B1

Example Non-ideal gas Given • Gas → ideal gas for large T & V ∂P Nk � � = • ∂T V − Nb V 2 aN 2 ∂P NkT � � = − + • ( V − Nb ) 2 V 3 ∂V T Find P 8.044 L6B2

∂P ∂P � � � � dP = dV + dT ∂V T ∂T V ∂P Nk � � � � � � P = dT + f ( V ) = dT + f ( V ) ∂T V V − Nb NkT = ( V − Nb ) + f ( V ) 8.044 L6B3

2 aN 2 ∂P NkT NkT + f I ( V = − = − V ) + ( V − Nb ) 2 ( V − Nb ) 2 V 3 ∂V j ) T j V ) 2 aN 2 aN 2 � f ( V ) = dV = − + c V 3 V 2 aN 2 NkT P = + c − V 2 ( V − Nb ) but c = 0 since P → NkT/V as V → ∞ 8.044 L6B4

Internal Energy U Observational fact initial final isolated ∆ W (adiabatic) Final state is independent of how ∆ W is applied. Final state is independent of which adiabatic path is followed. 8.044 L6B5

⇒ a state function U such that ∆ U = ∆ W adiabatic U = U (independent variables) = U ( T, V ) or U ( T, P ) or U ( P, V ) for a simple fluid 8.044 L6B6

Heat If the path is not adiabatic, dU = d /W d /Q ≡ dU − d /W d /Q is the heat added to the system. It has all the properties expected of heat. 8.044 L6B7

First Law of Thermodynamics dU = d /Q + d /W • U is a state function • Heat is a flow of energy • Energy is conserved 8.044 L6B8

Ordering of temperatures dQ T 1 T 2 When d /W = 0, heat flows from high T to low T. 8.044 L6B9

Example Hydrostatic System: gas, liquid or simple solid Variables (with N fixed): P, V, T, U . Only 2 are independent.     d /Q d /Q C V ≡ C P ≡     dT dT V P Examine these heat capacities. 8.044 L6B10

dU = d /Q + d /W = d /Q − P dV d /Q = dU + P dV d . We have dV . We want dT ∂U ∂U � � � � dU = dT + dV ∂T V ∂V T 8.044 L6B11

∂U ∂U � � �� � � /Q = d dT + + P dV ∂T V ∂V T d /Q ∂U ∂U dV � � �� � � + P = + ⇒ dT ∂T V ∂V T dT ⎛ ⎞ /Q d ∂U � � C V ≡ ⎝ = ⎠ dT ∂T V V 8.044 L6B12

⎛ ⎞ � � �� � � � � /Q d ∂U ∂U ∂V C P ≡ ⎝ + P = + ⎠ dT ∂T V ∂V T ∂T P P " T v " T v C V αV �� � � ∂U C P − C V = + P αV ∂V T The 2 nd law will allow us to simplify this further. � � ∂U Note that C P = ∂T P . 8.044 L6B13

�������������������������������������������� ������� ���������� ���� ���������� ��������� ������ ������� ∆ ��� ∆ ��� ∆ ��� ∆ ��� 8.044 L6B14

∆ ����������������������������������������� ������������������ ρ ��� � � ��������������������������������������������������� �� � ����� ρκ � 8.044 L6B15

������������������������������������������������ ∂U � ������������������������������������� = 0 ∂V T � �������������������� � ���������� ���������� ���������� ������������������� � ��������� � ���� � 8.044 L6B16

No work done so ∆ W = 0 T f = T i ⇒ ∆ Q = 0 together ⇒ ∆ U = 0 ( ∂U/∂V ) T = 0 → , U= Q , U= Q here quasi-static changes • Physics: no interactions, single particle energies only ⇒ ( ∂U/∂V ) T = 0 • Thermo: 2 nd law + ( PV = NkT ) ⇒ ( ∂U/∂V ) T = 0 8.044 L6B17

Consequences ∂U ∂U dU = dT + dV ∂T V ∂V T j V ) j V ) 0 C V T � C V ( T ' ) dT ' + constant U = 0 j V ) set =0 3 Nk . In a monatomic gas one observes C V = 2 3 NkT . Then the above result gives U = C V T = 2 8.044 L6B18

� � � � ∂U ∂V C P − C V = ( + P ) ∂V ∂T T P , p , p 0 ∂ ∂T ( NkT/P ) P = Nk/P = Nk for any ideal gas Applying this to the monatomic gas one finds 3 5 C P = Nk + Nk = Nk 2 2 5 γ ≡ C P /C V = 3 8.044 L6B19

Adiabatic Changes /Q = 0 d Find the equation for the path. Consider a hydrostatic example. � � �� � � ∂U ∂U /Q = d dT + + P dV = 0 ∂T V ∂V T " T v " T v C V ( C P − C V ) /αV ⎛ ⎞ � � ∂T C P − C V ) 1 ( γ − 1) = − = − ⎝ ⎠ ∂V ∆ Q =0 C V αV αV This constraint defines the path. 8.044 L6B20

Apply this relation to an ideal gas. 1 1 ∂ 1 1 V 1 � ∂V � NkT � Nk � � � = = = = α ≡ V ∂T P V ∂T P V P V T T P Path dT T = − ( γ − 1) dV V ⎛ ⎞ dT dV T V = − ( γ − 1) → ln = − ( γ − 1) ln ⎝ ⎠ T V T 0 V 0 ⎞ − ( γ − 1) ⎛ ⎞ ⎛ T V = ⎝ ⎠ ⎝ ⎠ T 0 V 0 8.044 L6B21

Adiabatic Isothermal 1 = c TV γ − � "" PV = c ������� PV γ = c 1 " V − P ∝ dP P = − c) �������� dV V γ = 5 / 3 (monatomic) � 5 / 3 V − P ∝ dP 5 P = − 3 V dV 8.044 L6B22

������������������������� ���������� � ������������������ ������������������� ���������� ∆ ������ ���������� ∆ ������ ���������������� ������������ ∆ ������ ∆ �������������� ∆ ������ ∆ ���������������� � � ������� ���������� � � 8.044 L6B23

Starting with a few known facts, 1 st law, d /W , and state function math, one can find relations between some thermodynamic quantities, a general expression for dU , and the adiabatic constraint. Adding models for the equation of state and the heat capacity allows one to find the internal energy U and the adiabatic path. 8.044 L6B24

MIT OpenCourseWare http://ocw.mit.edu 8.044 Statistical Physics I Spring 2013 For information about citing these materials or our Terms of Use, visit: http://ocw.mit.edu/terms.