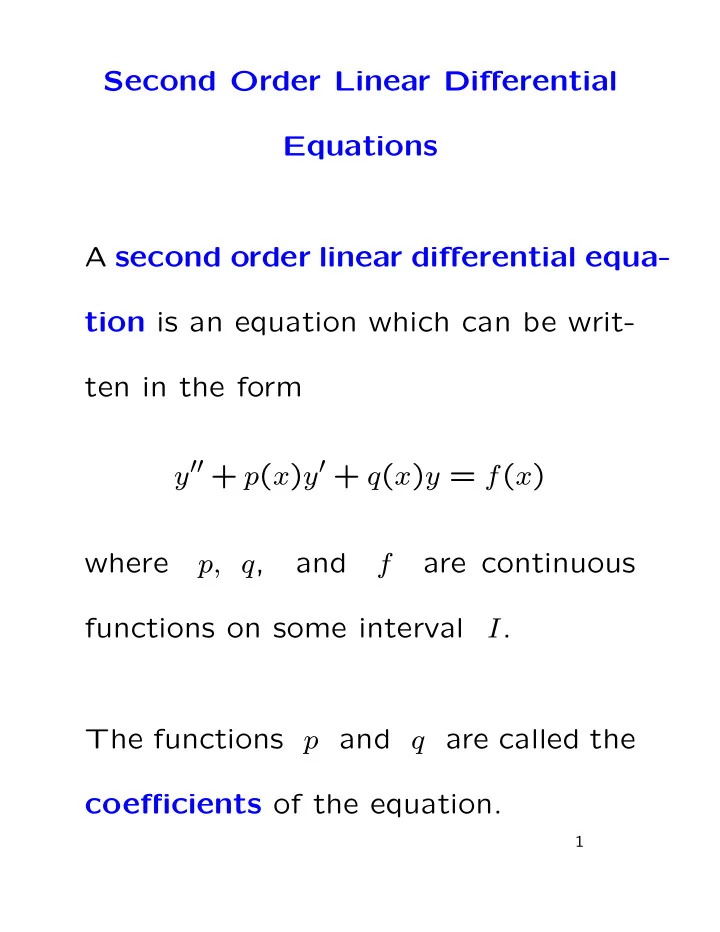
Second Order Linear Differential Equations A second order linear - PDF document

Second Order Linear Differential Equations A second order linear differential equa- tion is an equation which can be writ- ten in the form y ′′ + p ( x ) y ′ + q ( x ) y = f ( x ) where p, q , and are continuous f functions on some interval I . The functions p and q are called the coefficients of the equation. 1

The function is called the forcing f function or the nonhomogeneous term .

“Linear” L [ y ] = y ′′ + p ( x ) y ′ + q ( x ) y . Set Then, for any two twice differentiable functions y 1 ( x ) and y 2 ( x ), L [ y 1 ( x ) + y 2 ( x )] = L [ y 1 ( x )] + L [ y 2 ( x )] and, for any constant c , L [ cy ( x )] = cL [ y ( x )] . That is, is a linear differential L operator . 2

L [ y ] = y ′′ + py ′ + qy L [ y 1 + y 2 ] = ( y 1 + y 2 ) ′′ + p ( y 1 + y 2 ) ′ + q ( y 1 + y 2 ) = y ′′ 1 + y ′′ y ′ 1 + y ′ � � 2 + p + q ( y 1 + y 2 ) 2 = y ′′ 1 + y ′′ 2 + py ′ 1 + py ′ 2 + qy 1 + qy 2 y ′′ 1 + py ′ y ′′ 2 + py ′ � � � � = 1 + qy 1 + 2 + qy 2 = L [ y 1 ] + L [ y 2 ] L [ cy ] = ( cy ) ′′ + p ( cy ) ′ + q ( cy ) = cy ′′ + pcy ′ + qcy = c y ′′ + py ′ + qy � � = cL [ y ] 3

Existence and Uniqueness THEOREM: Given the second order linear equation (1). Let be any a point on the interval I , and let α and be any two real numbers. Then the β initial-value problem y ′′ + p ( x ) y ′ + q ( x ) y = f ( x ) , y ( a ) = α, y ′ ( a ) = β has a unique solution. 4

Homogeneous/Nonhomogeneous Equations The linear differential equation y ′′ + p ( x ) y ′ + q ( x ) y = f ( x ) (1) is homogeneous ∗ if the function f on the right side is 0 for all x ∈ I . That is, y ′′ + p ( x ) y ′ + q ( x ) y = 0 . is a linear homogeneous equation. ∗ There is no relation between the term here and 1st order ”homogeneous” equations in 2.3 5

If is not the zero function on I , f that is, if f ( x ) � = 0 for some x ∈ I , then y ′′ + p ( x ) y ′ + q ( x ) y = f ( x ) is a linear nonhomogeneous equation. 6

Section 3.2. Homogeneous Equa- tions y ′′ + p ( x ) y ′ + q ( x ) y = 0 (H) where and are continuous func- p q tions on some interval I . The zero function, y ( x ) = 0 for all x ∈ I , ( y ≡ 0) is a solution of (H). The zero solution is called the trivial solution . Any other solution is a non- trivial solution. 7

Recall, Example 8, Chap. 1, pg 20: Find a value of r , if possible, such y = x r that is a solution of y ′′ − 1 x y ′ − 3 x 2 y = 0 . y ≡ 0 is a solution (trivial) y 2 = x 3 are solutions y 1 = x − 1 , 8

Basic Theorems THEOREM 1: If y = y 1 ( x ) and y = y 2 ( x ) are any two solutions of (H), then u ( x ) = y 1 ( x ) + y 2 ( x ) is also a solution of (H). The sum of any two solutions of (H) is also a solution of (H) . (Some call this property the superposition prin- ciple ). 9

Proof: y 1 and y 2 are solutions. Therefore, L [ y 1 ] = 0 and L [ y 2 ] = 0 L is linear. Therefore 10

THEOREM 2: If y = y ( x ) is a solution of (H) and if is any real C number, then u ( x ) = Cy ( x ) is also a solution of (H). Proof: y is a solution means L [ y ] = 0. L is linear: Any constant multiple of a solution of (H) is also a solution of (H) . 11

DEFINITION: Let y = y 1 ( x ) and y = y 2 ( x ) be functions defined on some interval I , and let and be C 1 C 2 real numbers. The expression C 1 y 1 ( x ) + C 2 y 2 ( x ) is called a linear combination of y 1 and y 2 . 12

Theorems 1 & 2 can be restated as: THEOREM 3: If y = y 1 ( x ) and y = y 2 ( x ) are any two solutions of (H), and if and are any two C 1 C 2 real numbers, then y ( x ) = C 1 y 1 ( x ) + C 2 y 2 ( x ) is also a solution of (H). Any linear combination of solutions of (H) is also a solution of (H) . 13

NOTE: y ( x ) = C 1 y 1 ( x ) + C 2 y 2 x is a two-parameter family which ”looks like“ the general solution. Is it??? NOTE: A linear equation does not have singular solutions so the general solu- tion represents all solutions. 14

Some Examples from Chapter 1: 1. y 1 = cos 3 x and y 2 = sin 3 x are solutions of y ′′ + 9 y = 0 (Chap 1, p. 47) y = C 1 cos 3 x + C 2 sin 3 x is the general solution. 15

y 1 = e − 2 x y 2 = e 4 x 2. and are solutions of y ′′ − 2 y ′ − 8 y = 0 (Chap 1, p. 55) and y = C 1 e − 2 x + C 2 e 4 x is the general solution. 16

y 2 = x 3 3. y 1 = x and are solutions of y ′′ − 3 xy ′ − 3 x 2 y = 0 (Chap 1 notes, p. 56) and y = C 1 x + C 2 x 3 is the general solution. 17

y ′′ − 1 xy ′ − 15 Example: x 2 y = 0 a. Solutions y 1 ( x ) = x 5 , y 2 ( x ) = 3 x 5 General solution: y = C 1 x 5 + C 2 (3 x 5 ) ?? That is, is EVERY solution a linear combination of and y 2 ? y 1 18

ANSWER: NO!!! y = x − 3 is a solution AND x − 3 � = C 1 x 5 + C 2 (3 x 5 ) C 1 x 5 + C 2 (3 x 5 ) = M x 5 x − 3 is NOT a constant multiple of x 5 . 19

Now consider y 1 ( x ) = x 5 , y 2 ( x ) = x − 3 y = C 1 x 5 + C 2 x − 3 ? General solution: That is, is EVERY solution a linear combination of y 1 and y 2 ?? Let y = y ( x ) be the solution of the equation that satisfies y ′ (1) = y (1) = 20

y = C 1 x 5 + C 2 x − 3 y ′ = 5 C 1 x 4 − 3 C 2 x − 4 At x = 1: C 1 + C 2 = 5 C 1 − 3 C 2 = 21

In general: Let y = C 1 y 1 ( x ) + C 2 y 2 ( x ) be a family of solutions of (H). When is this the general solution of (H)? EASY ANSWER: When and y 1 y 2 ARE NOT CONSTANT MULTIPLES OF EACH OTHER. That is, y 1 and y 2 are independent of each other. 22

Let y = C 1 y 1 ( x ) + C 2 y 2 ( x ) be a two parameter family of solutions of (H). Choose any number a ∈ I and let u be any solution of (H). u ′ ( a ) = β Suppose u ( a ) = α, 23

Does the system of equations C 1 y 1 ( a ) + C 2 y 2 ( a ) = α C 1 y ′ 1 ( a ) + C 2 y ′ 2 ( a ) = β have a unique solution?? 24

DEFINITION: Let y = y 1 ( x ) and y = y 2 ( x ) be solutions of (H). The function defined by W W [ y 1 , y 2 ]( x ) = y 1 ( x ) y ′ 2 ( x ) − y 2 ( x ) y ′ 1 ( x ) is called the Wronskian of y 1 , y 2 . Determinant notation: W ( x ) = y 1 ( x ) y ′ 2 ( x ) − y 2 ( x ) y ′ 1 ( x ) � � y 1 ( x ) y 2 ( x ) � � = � � y ′ 1 ( x ) y ′ � � 2 ( x ) � � � � 25

THEOREM 4: Let y = y 1 ( x ) and y = y 2 ( x ) be solutions of equation (H), and let W ( x ) be their Wronskian. Ex- actly one of the following holds: (i) W ( x ) = 0 for all x ∈ I and is y 1 a constant multiple of y 2 , AND y = C 1 y 1 ( x ) + C 2 y 2 ( x ) IS NOT the general solution of (H) OR 26

(ii) W ( x ) � = 0 for all x ∈ I , AND y = C 1 y 1 ( x ) + C 2 y 2 ( x ) IS the general solution of (H) (Note: W ( x ) is a solution of y ′ + p ( x ) y = 0 . See Section 2.1, Special Case.) The Proof is in the text.

Fundamental Set; Solution basis DEFINITION: A pair of solutions y = y 1 ( x ) , y = y 2 ( x ) of equation (H) forms a fundamental set of solutions (also called a solution basis ) if W [ y 1 , y 2 ]( x ) � = 0 for all x ∈ I. 27

Section 3.3. Homogeneous Equa- tions with Constant Coefficients Fact: In contrast to first order linear equations, there are no general meth- ods for solving y ′′ + p ( x ) y ′ + q ( x ) y = 0 . (H) But, there is a special case of (H) for which there is a solution method, namely 28

y ′′ + ay ′ + by = 0 (1) where and are constants. a b Solutions: (1) has solutions of the form y = e rx 29

y = e rx is a solution of (1) if and only if r 2 + ar + b = 0 (2) Equation (2) is called the character- istic equation of equation (1) See Chap 1 notes, Example 2, p. 18, Example 3 p. 55 30

Note the correspondence: y ′′ + ay ′ + by = 0 Diff. Eqn: r 2 + ar + b = 0 Char. Eqn: y = e rx The solutions of y ′′ + ay ′ + by = 0 are determined by the roots of r 2 + ar + b = 0 . 31

There are three cases: 1. r 2 + ar + b = 0 has two, distinct real roots , r 1 = α, r 2 = β . 2. r 2 + ar + b = 0 has only one real root , r = α . 3. r 2 + ar + b = 0 has complex con- jugate roots , r 1 = α + i β, r 2 = β � = 0. α − i β, 32

Case I: Two, distinct real roots. r 2 + ar + b = 0 has two distinct real roots: r 1 = α, r 2 = β, α � = β. Then y 1 ( x ) = e αx y 2 ( x ) = e βx and y ′′ + ay ′ + by = 0. are solutions of 33

y 1 = e αx y 2 = e βx and are not con- stant multiples of each other, { y 1 , y 2 } is a fundamental set, � � y 1 ( x ) y 2 ( x ) � � W [ y 1 , y 2 ] = y 1 y ′ 2 − y 2 y ′ 1 = � � y ′ 1 ( x ) y ′ � � 2 ( x ) � � � � General solution: y = C 1 e αx + C 2 e βx 34

Example 1: Find the general solution of y ′′ − 3 y ′ − 10 y = 0 . 35

Example 2: Find the general solution of y ′′ − 11 y ′ + 28 y = 0 . 36

Case II: Exactly one real root. r = α ; ( α is a double root ). Then y 1 ( x ) = e αx y ′′ + ay ′ + by = 0. is one solution of We need a second solution which is in- dependent of y 1 . 37

NOTE: In this case, the characteristic equation is ( r − α ) 2 = r 2 − 2 αr + α 2 = 0 so the differential equation is y ′′ − 2 αy ′ + α 2 y = 0 38