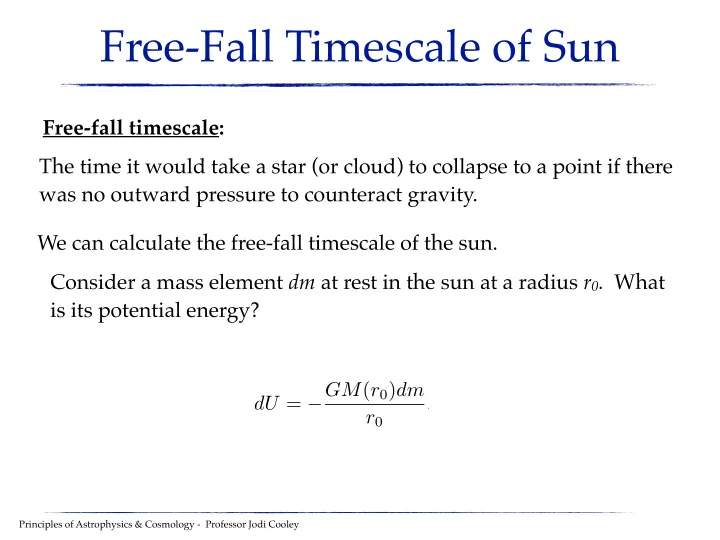
Free-Fall Timescale of Sun Free-fall timescale: The time it would - PowerPoint PPT Presentation

Free-Fall Timescale of Sun Free-fall timescale: The time it would take a star (or cloud) to collapse to a point if there was no outward pressure to counteract gravity. We can calculate the free-fall timescale of the sun. Consider a mass element dm at rest in the sun at a radius r 0 . What is its potential energy? dU = − GM ( r 0 ) dm , r 0 interior to . From conse Principles of Astrophysics & Cosmology - Professor Jodi Cooley

Next, apply the conservation of energy: E i = E f U i + K i = U f + K f +1 − GM ( r 0 ) dm dt ) 2 = − GM ( r 0 ) dm 2 dm ( dr r r 0 Remember, we are looking for free-fall time. Simplify: dt ) 2 = 2[ GM ( r 0 ) − GM ( r 0 ) ( dr ] r 0 r dt ) = [ − 2 GM ( r 0 )(1 r − 1 ( dr 1 )] 2 r 0 Principles of Astrophysics & Cosmology - Professor Jodi Cooley

( dr dt ) = [ − 2 GM ( r 0 )(1 r − 1 1 Continuing to simplify: )] 2 r 0 dt = [ − 2 GM ( r 0 )(1 r − 1 )] − 1 2 dr r 0 To find t ff we integrate: Z τ ff Z 0 ∂∏ − 1 / 2 ∑ µ 1 r − 1 τ ff = dt = − 2 GM ( r 0 ) dr r 0 r 0 0 As 5 points extra credit (due at the beginning of next class), show that from the above equation, you get the free-fall time of µ 3 π ∂ 1 / 2 τ ff = 32 G ¯ ρ Principles of Astrophysics & Cosmology - Professor Jodi Cooley

Let's examine the solution. Does it contain the correct units (use cgs)? µ 3 π ∂ 1 / 2 τ ff = 32 G ¯ ρ G = [erg][cm][g -2 ] ρ = [g][cm -3 ] erg = [g][cm 2 ][s -2 ] p [ g cm 2 s − 2 ][ cm ][ g − 2 ][ g ][ cm − 3 ] units = units = [ s ] Principles of Astrophysics & Cosmology - Professor Jodi Cooley

For the parameters of the Sun, calculate the free-fall timescale. ∂ 1 / 2 µ 3 π τ ff ⊙ = = 1800 s . 32 × 6 . 7 × 10 − 8 cgs × 1 . 4 g cm − 3 hus without pressure support, the Sun would collapse to a point w Observation: Without pressure support, the sun would collapse very quickly! The sun does not collapse because it is in hydrostatic equilibrium . This means that the sun is in a state of balance by which the internal pressure exactly balances the gravitational pressure. Principles of Astrophysics & Cosmology - Professor Jodi Cooley

Hydrostatic Equilibrium Back to Introductory Mechanics! Consider a small cylinder-shaped star mass element of area A and height dr . The pressure difference between the top and bottom of the cylinder is dP . It leads to a net force (due to pressure) F pressure = AdP Equilibrium will exist if there is no net force. Σ F = 0 − GM ( r ) dm − AdP = 0 r 2 Principles of Astrophysics & Cosmology - Professor Jodi Cooley

− GM ( r ) dm The mass element dm is given by the − AdP = 0 r 2 definition of density dm = ρ ( r ) Adr, Combining with our expression for equilibrium, we find AdP = − GM ( r ) ρ ( r ) r 2 Simplifying gives us the Equation of Hydrostatic Equilibrium , the first equation of stellar structure that we will study. dP ( r ) = − GM ( r ) ρ ( r ) Stop and Think: This pressure gradient is . negative. Does that make sense? r 2 dr gradient is negative, because to Principles of Astrophysics & Cosmology - Professor Jodi Cooley

dP ( r ) = − GM ( r ) ρ ( r ) . r 2 dr Now let’s add some thermodynamics (for fun): gradient is negative, because to First, let’s introduce a cleaver 1. Multiply both sides of our equation by 4 π r 3 dr . dr dr = GM ( r ) ρ ( r ) 4 πr 3 dP 4 πr 3 dr r 2 Simplify and integrate from the star’s interior to it’s radius: o , the outer radius of the star: Z r ∗ Z r ∗ GM ( r ) ρ ( r )4 π r 2 dr 4 π r 3 dP dr dr = − . r 0 0 nd side, in the form we have written it, is seen to be This side we will have to This is the gravitational self-potential integrate by parts to solve. energy of the star. It is equal to Egr. Principles of Astrophysics & Cosmology - Professor Jodi Cooley

o , the outer radius of Z r ∗ 4 π r 3 dP Recall integration by parts: dr dr 0 nd side, in the form w Z Z udv = uv − vdu Let dv = dP ( r ) u = 4 πr 3 dr dr Z dP ( r ) du = 3(4 πr 2 ) dr v = dr = P ( r ) dr Putting it together: 0 Z r ∗ Z r ∗ 4 πr 3 dP ( r ) dr = [4 πr 3 P ( r )] r ∗ P ( r )(3)(4) πr 2 dr 0 − dr 0 0 Z r ∗ ¯ P P ( r )4 πr 2 dr = − 3 = − 3 V 0 This is the volume-averaged pressure divided by the volume of the star Principles of Astrophysics & Cosmology - Professor Jodi Cooley

Putting it all together: o , the outer radius of the star: Z r ∗ Z r ∗ GM ( r ) ρ ( r )4 π r 2 dr 4 π r 3 dP dr dr = − . r 0 0 nd side, in the form we have written it, is seen to be ¯ P V = E gr − 3 Which gives the one form of the viral theorem for a gravitationally bound system. P = − 1 E gr ¯ V . 3 in a star equals m This tells us that the pressure inside a star is one third its gravitational energy density. Principles of Astrophysics & Cosmology - Professor Jodi Cooley

Now let’s add some classical thermodynamics. If a star is composed of a classical, non relativistic, mono-atomic ideal gas of N particles, what is the gas equation of stat of the star? …(1) PV = NkT, What is its thermal energy? E th = 3 …(2) 2 NkT Substituting NkT from (2) into (1) we find P = 2 E th V , 3 2/3 the local therm The local pressure is equal to 2/3 the local thermal energy density. Principles of Astrophysics & Cosmology - Professor Jodi Cooley

Multiply by 4 π r 2 and integrating over the volume of the star, we find PV = 2 ¯ 3 E tot th rgy of the star. Subs Recall the our first form of the viral theorem: P = − 1 E gr ¯ 3 V Substituting gives another form of the viral theorem : th = − E gr E tot 2 , The second form of the viral theorem says when a star contacts and losses energy, its self gravity becomes more negative. Principles of Astrophysics & Cosmology - Professor Jodi Cooley

Pressure Inside a Star Let’s examine the following equation again. Z r ∗ GM ( r ) ρ ( r )4 πr 2 dr E gr = − r 0 This is the gravitational self-potential energy of the star. It is equal to Egr. Let’s assume that the density profile is constant, then Z r ∗ Z r ∗ G 4 π 3 r 3 ρ 2 4 πr 2 dr GM ( r ) ρ ( r )4 πr 2 dr E gr = − = − r r 0 0 recall: A little math and we get …. ρ const = M V = M ∗ 4 3 πr 3 ∗ GM 2 E gr = − 3 ∗ r ∗ 5 Principles of Astrophysics & Cosmology - Professor Jodi Cooley

Mean Pressure of the Sun Take a characteristic E gr ~ -GM 2 /r and calculate the mean pressure in the sun. Using the first form of the viral theorem, we get = GM 2 GM 2 ∼ 1 P = 1 E gr ⊙ ⊙ ¯ ≈ 4 π r 4 4 3 π r 3 3 ⊙ r ⊙ V 3 ⊙ a typical temperature perature, which P = (6 . 7 × 10 − 8 erg cm g − 2 )(2 . 0 × 10 33 g ) 2 ¯ = 8 . 9 × 10 14 erg cm − 3 4 π (7 . 0 × 10 10 cm ) 4 P ∼ 8 . 9 × 10 14 erg cm − 3 Note: Your textbook uses units of dyne cm -2 . 1 dyne = 1 erg cm -1 . Principles of Astrophysics & Cosmology - Professor Jodi Cooley

Typical Temperature Viral Temperature is the typical temperature inside a star. To find the viral temperature we start with our second form of the virial theorem. and GM 2 th = − E gr 3 r ∼ 1 E tot ⊙ = 2 , 2 NkT vir 2 r ⊙ ectron is negligi negligibly small, If the particles have a mean mass m we can write: 3 1 GM ⊙ N ¯ m 2 NkT vir ∼ 2 r ⊙ lectron is negligibl , only The sun is comprised of mostly ionized hydrogen gas, consisting of an equal number of protons and electrons. mass , = m H m = m e + m p Note: ¯ m e << m p , thus m H ~ 1/2 m p. 2 2 one-half the mass of the of the proton Principles of Astrophysics & Cosmology - Professor Jodi Cooley

Putting this together, we have 3 1 GM ⊙ N ¯ m 2 NkT vir ∼ 2 r ⊙ lectron is negligibl , only = 6 . 7 × 10 − 8 cgs × 2 × 10 33 g × 1 . 7 × 10 − 24 g kT vir ∼ GM ⊙ m H 6 × 7 × 10 10 cm 6 r ⊙ = 5 . 4 × 10 − 10 erg ith k = 1 . 4 × 10 − 16 erg K − 1 Divide by ure of about . As we w T vir ~ 4 x 10 -6 K Nuclear reactions take place at temperatures of this order of magnitude. So nuclear reactions can take place and thus replenish the thermal energy that a star radiates away. This, temporarily halts the gravitational collapse. Principles of Astrophysics & Cosmology - Professor Jodi Cooley

Mass Continuity For a spherically symmetric star, consider a shell of mass dM r and thickness dr located a distance r from the center. If the shell is thin( dr << r ) the volume of the shell can be approximated as dV = 4 π r 2 dr . If the local density is given by ρ (r), then the shell’s mass is given by dM ( r ) = ρ ( r )4 π r 2 dr, Re-arranging terms yields the Equation of Mass Continuity . dM ( r ) = 4 π r 2 ρ ( r ) . dr Principles of Astrophysics & Cosmology - Professor Jodi Cooley