Curve Sketching Since we have graphing calculators, we can find the - PDF document

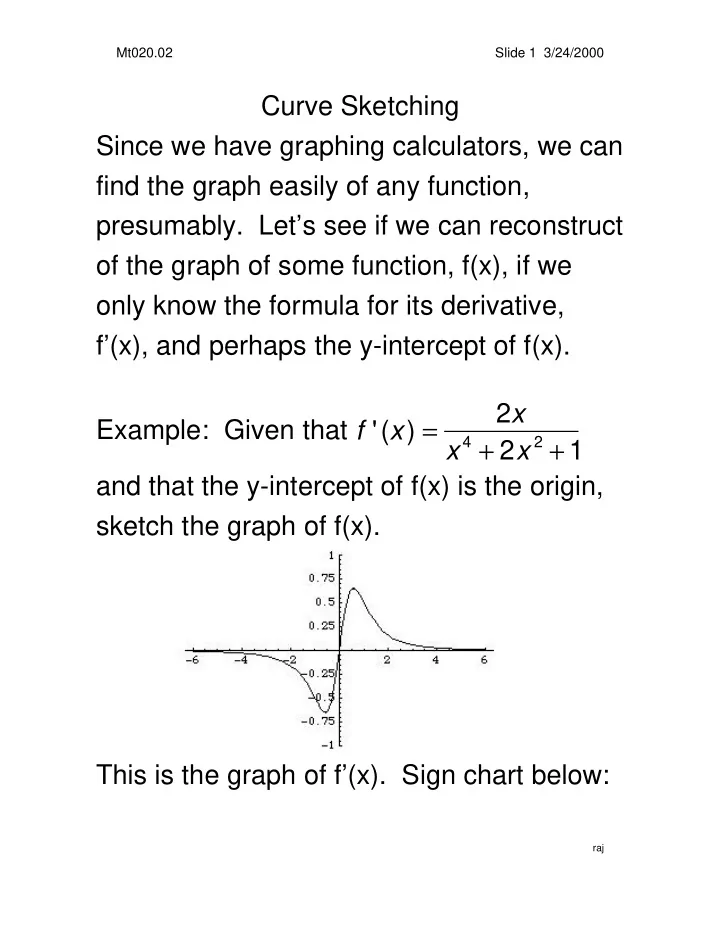
Mt020.02 Slide 1 3/24/2000 Curve Sketching Since we have graphing calculators, we can find the graph easily of any function, presumably. Let’s see if we can reconstruct of the graph of some function, f(x), if we only know the formula for its derivative, f’(x), and perhaps the y-intercept of f(x). 2 x Example: Given that f '( x ) = x 4 + 2 x 2 + 1 and that the y-intercept of f(x) is the origin, sketch the graph of f(x). This is the graph of f’(x). Sign chart below: raj

Mt020.02 Slide 2 3/24/2000 The function f itself will be decreasing until we hit zero and increasing thereafter. Because the derivative changes signs across zero, from – to +, we know that the point (0,f(0)) will turn out to be a relative minimum of f. This is the y-intercept, (0,0). ________________ We differentiate the function f’(x) to find the second derivative f’’(x) which holds the key to the concavity behavior of f(x). f '' ( x ) = 2 − 6 x 2 1 + x 2 ) 3 . The graph of f’’ is ( Sign chart for f’’ below: raj

Mt020.02 Slide 3 3/24/2000 Here is the assembly of our results: f is dec. for x<0 | f is inc. for x>0 x=0 f is ccd | f is ccu | f is ccd -0.577 0.577 Here’s our sketch: raj

Mt020.02 Slide 4 3/24/2000 x 2 And the answer is f ( x ) = 1 + x 2 and its graph ____________________________ Asymptotes are lines to which the graph of a function seems to adhere to as we get further from the origin. In the previous example, our function had a horizontal asymptote y=1, because as we stray further from the origin the graph seems to be attracted by the line y=1. It is also possible to have vertical asymptotes at places where the function seems to want to be infinite. Like this: raj

Mt020.02 Slide 5 3/24/2000 1 f ( x ) = x − 2 This function has a vertical asymptote at x=2 and a horizontal asymptote y=0. Example: Find asymptotes for the function h ( x ) = 4 x 2 − 2 x 2 − 1 . We see that the denominator will be zero for x = ±1 and since those values do not make the numerator zero, we expect vertical asymptotes at x = ±1. And since raj

Mt020.02 Slide 6 3/24/2000 4 x 2 − 2 lim lim x → ∞ h ( x ) = x 2 − 1 = 4 we see x → ∞ that our graph should have horizontal asymptotes at y=4. Here’s a preliminary sketch of the playing field. If we differentiate h(x) we find that − 4 x h ' ( x ) = ) 2 which has this shape: ( x 2 − 1 raj

Mt020.02 Slide 7 3/24/2000 And this sign chart: h’(x) | | | -1 0 1 Note that h (and h’) are not defined at x=±1, which should both appear as critical points where the sign of the derivative could shift. We see h is max at x=0 and that h(0) = 2. Differentiating again we get formula and graphs for h’’: x ) = 4(3 x 2 + 1 ) h ' ' ( ( x 2 − 1 ) 3 raj

Mt020.02 Slide 8 3/24/2000 Sign chart for h’’ h’’ | | -1 1 Here’s our sketch putting all of our information together: raj

Mt020.02 Slide 9 3/24/2000 And here is the real graph of h(x) raj