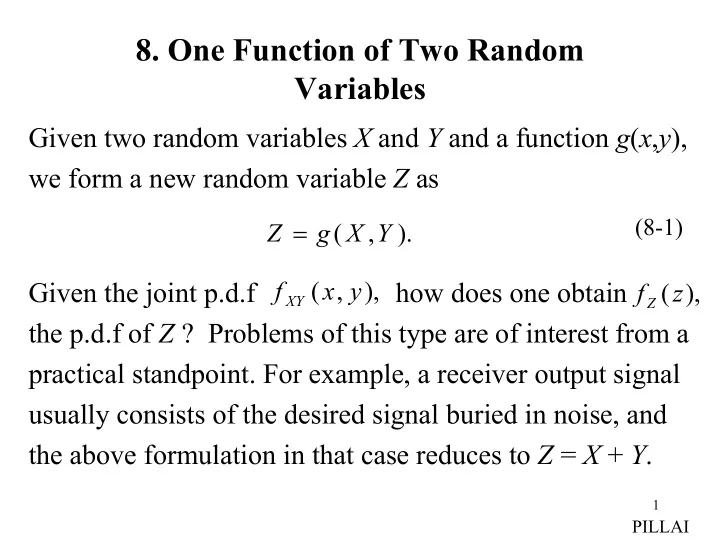
8. One Function of Two Random Variables Given two random variables X - PowerPoint PPT Presentation

8. One Function of Two Random Variables Given two random variables X and Y and a function g ( x , y ), we form a new random variable Z as (8-1) Z = g ( X , Y ). f XY ( x , y ), Given the joint p.d.f how does one obtain f Z ( z ), the p.d.f of Z ? Problems of this type are of interest from a practical standpoint. For example, a receiver output signal usually consists of the desired signal buried in noise, and the above formulation in that case reduces to Z = X + Y . 1 PILLAI

It is important to know the statistics of the incoming signal for proper receiver design. In this context, we shall analyze problems of the following type: X + Y max( X , Y ) X − Y Z = min( X , Y ) g ( X , Y ) XY (8-2) X / Y X + 2 2 Y − tan 1 ( X / Y ) Referring back to (8-1), to start with [ ] ( ) ( ) = ξ ≤ = ≤ = ∈ F ( z ) P Z ( ) z P g ( X , Y ) z P ( X , Y ) D Z z ∫ ∫ = f ( x , y ) dxdy , (8-3) XY ∈ 2 x , y D z PILLAI

where in the XY plane represents the region such D z ≤ that is satisfied. Note that need not be simply g ( x , y ) z D z connected (Fig. 8.1). From (8-3), to determine it is F Z ( z ) enough to find the region for every z , and then evaluate D z the integral there. We shall illustrate this method through various examples. Y D z D z X 3 Fig. 8.1 PILLAI

Example 8.1: Z = X + Y. Find f Z ( z ). Solution: ) ∫ + ∞ − ( z y ∫ (8-4) = + ≤ = F ( z ) P X Y z f ( x , y ) dxdy , Z XY = −∞ = −∞ y x since the region of the xy plane where is the + ≤ x y z D z shaded area in Fig. 8.2 to the left of the line + = x y z . Integrating over the horizontal strip along the x -axis first (inner integral) followed by sliding that strip along the y -axis + ∞ from to (outer integral) we cover the entire shaded − ∞ area. y = − x z y x 4 Fig. 8.2 PILLAI

We can find by differentiating directly. In this f Z ( z ) F Z ( z ) context, it is useful to recall the differentiation rule in (7- 15) - (7-16) due to Leibnitz. Suppose b ( z ) ∫ = H ( z ) h ( x , z ) dx . (8-5) a ( z ) Then ∂ dH ( z ) db ( z ) da ( z ) ) ∫ h ( x , z ) ( ) ( b ( z ) = − + h b ( z ), z h a ( z ), z dx . (8-6) ∂ dz dz dz z a ( z ) Using (8-6) in (8-4) we get ∂ ∂     f ( , ) x y +∞ − +∞ − z y z y ∫ ∫ ∫ ∫ = = − − + f ( ) z f ( , ) x y dx dy f ( z y y , ) 0 XY d y     Z XY XY ∂ ∂  z   z  −∞ −∞ −∞ −∞ +∞ ∫ = − f ( z y y dy , ) . (8-7) XY −∞ Alternatively, the integration in (8-4) can be carried out first along the y -axis followed by the x -axis as in Fig. 8.3. 5 PILLAI

In that case + ∞ − z x y ∫ ∫ = F ( z ) f ( x , y ) dxdy , (8-8) Z XY = −∞ = −∞ x y and differentiation of (8-8) = − y z x gives ∂ dF ( z )   + ∞ − z x ∫ ∫ = = f ( z ) Z  f ( x , y ) dy  dx x Z XY ∂ dz  z  = −∞ = −∞ x y + ∞ ∫ = − (8-9) f ( x , z x ) dx . Fig. 8.3 XY = −∞ x If X and Y are independent, then (8-10) = f ( x , y ) f ( x ) f ( y ) XY X Y and inserting (8-10) into (8-8) and (8-9), we get + ∞ + ∞ ∫ ∫ = − = − (8-11) f ( z ) f ( z y ) f ( y ) dy f ( x ) f ( z x ) dx . Z X Y X Y = −∞ = −∞ y x 6 PILLAI

The above integral is the standard convolution of the functions and expressed two different ways. We f X ( z ) f Y ( z ) thus reach the following conclusion: If two r.vs are independent, then the density of their sum equals the convolution of their density functions. As a special case, suppose that for and = = < f Y ( y ) 0 f X ( x ) 0 x 0 for then we can make use of Fig. 8.4 to determine the < y 0 , new limits for D . z y ( z , 0 ) = − x z y x ( 0 , z ) 7 Fig. 8.4 PILLAI

In that case − z z y ∫ ∫ = F ( z ) f ( x , y ) dxdy Z XY = = y 0 x 0 or  z ∫ ∂    − > f ( z y , y ) dy , z 0 , − z z y ∫ ∫ = = f ( z )  f ( x , y ) dx  dy  XY (8-12) 0 Z XY ∂  z   = = ≤ y 0 x 0 0 , z 0 .  On the other hand, by considering vertical strips first in Fig. 8.4, we get − z z x ∫ ∫ = F ( z ) f ( x , y ) dydx Z XY = = x 0 y 0 or  z ∫  − > f ( x ) f ( z x ) dx , z 0 , z ∫ = − = f ( z ) f ( x , z x ) dx  X Y = (8-13) y 0 Z XY =  x 0 ≤ 0 , z 0 ,  if X and Y are independent random variables. 8 PILLAI

Example 8.2: Suppose X and Y are independent exponential r.vs with common parameter λ , and let Z = X + Y . Determine f Z ( z ). − λ − λ Solution: We have = λ = λ x y (8-14) f ( x ) e U ( x ), f ( y ) e U ( y ), X Y and we can make use of (13) to obtain the p.d.f of Z = X + Y . z z ∫ ∫ (8-15) = λ − λ − λ − = λ − λ = λ − λ 2 x ( z x ) 2 z 2 z f ( z ) e e dx e dx z e U ( z ). Z 0 0 As the next example shows, care should be taken in using the convolution formula for r.vs with finite range. Example 8.3: X and Y are independent uniform r.vs in the common interval (0,1). Determine where Z = X + Y . f Z ( z ), Solution: Clearly, here, and as Fig. 8.5 = + ⇒ < < Z X Y 0 z 2 shows there are two cases of z for which the shaded areas are quite different in shape and they should be considered 9 separately. PILLAI

y y = − x z y = − x z y x x < z < ( b ) 1 2 < z < ( a ) 0 1 Fig. 8.5 For ≤ z < 0 1 , 2 z − z z y z (8-16) ∫ ∫ ∫ = = − = ≤ < F ( z ) 1 dxdy ( z y ) dy , 0 z 1 . Z 2 = = = y 0 x 0 y 0 ≤ z < For notice that it is easy to deal with the unshaded 1 2 , region. In that case ( ) 1 1 ∫ ∫ = − > = − F ( z ) 1 P Z z 1 1 dxdy Z = − = − y z 1 x z y − 2 ( 2 z ) (8-17) 1 ∫ = − − + = − ≤ < 1 ( 1 z y ) dy 1 , 1 z 2 . 10 2 = − y z 1 PILLAI

Using (8-16) - (8-17), we obtain ≤ <  z 0 z 1 , dF ( z ) = = f ( z ) Z (8-18)  Z − ≤ < dz 2 z , 1 z 2 .  By direct convolution of and we obtain the f X ( x ) f Y ( y ), same result as above. In fact, for (Fig. 8.6(a)) ≤ z < 0 1 = ∫ z ∫ − = = f ( z ) f ( z x ) f ( x ) dx 1 dx z . (8-19) Z X Y 0 and for (Fig. 8.6(b)) ≤ z < 1 2 = ∫ − 1 = − (8-20) f ( z ) 1 dx 2 z . Z z 1 Fig 8.6 (c) shows which agrees with the convolution f Z ( z ) of two rectangular waveforms as well. 11 PILLAI

− f ( z x ) f ( x ) − f X ( z x ) f Y ( x ) X Y x x x z z − z 1 1 ≤ z < ( a ) 0 1 − f X ( z x ) − f Y ( x ) f ( z x ) f ( x ) X Y x x x z − z 1 1 − z 1 1 ≤ z < ( b ) 1 2 f Z ( z ) z 2 0 1 Fig. 8.6 ( c ) 12 PILLAI

= − Example 8.3: Let Determine its p.d.f Z X Y . f Z ( z ). Solution: From (8-3) and Fig. 8.7 ) ∫ + ∞ + ( z y ∫ = − ≤ = F ( z ) P X Y z f ( x , y ) dxdy Z XY = −∞ = −∞ y x and hence ∂   dF ( ) z +∞ + +∞ z y ∫ ∫ ∫ = = = + f ( ) z Z f ( , ) x y dx dy f ( y z y dy , ) . (8-21)   Z XY XY ∂ dz  z  =−∞ =−∞ −∞ y x If X and Y are independent, then the above formula reduces to +∞ ∫ (8-22) = + = − ⊗ f ( ) z f ( z y f ) ( ) y dy f ( z ) f ( ), y Z X Y X Y −∞ which represents the convolution of with f X − ( z ) f Y ( z ). y − = x y z y = + x y z x 13 Fig. 8.7 PILLAI

As a special case, suppose = < = < f ( x ) 0 , x 0 , and f ( y ) 0 , y 0 . X Y In this case, Z can be negative as well as positive, and that gives rise to two situations that should be analyzed < ≥ separately, since the region of integration for and z 0 z 0 are quite different. For from Fig. 8.8 (a) ≥ z 0 , y + ∞ + z y ∫ ∫ = F ( z ) f ( x , y ) dxdy = + x z y Z XY = = y 0 x 0 x z and for from Fig 8.8 (b) < z 0 , − z (a) + ∞ + z y ∫ ∫ y = F ( z ) f ( x , y ) dxdy Z XY = − = y z x 0 = + x z y After differentiation, this gives − z  + ∞ ∫ + ≥ f ( z y , y ) dy , z 0 ,  x XY = (8-23) f ( z )  0 + ∞ Z Fig. 8.8 (b) ∫ + <  f ( z y , y ) dy , z 0 .  XY − z 14 PILLAI