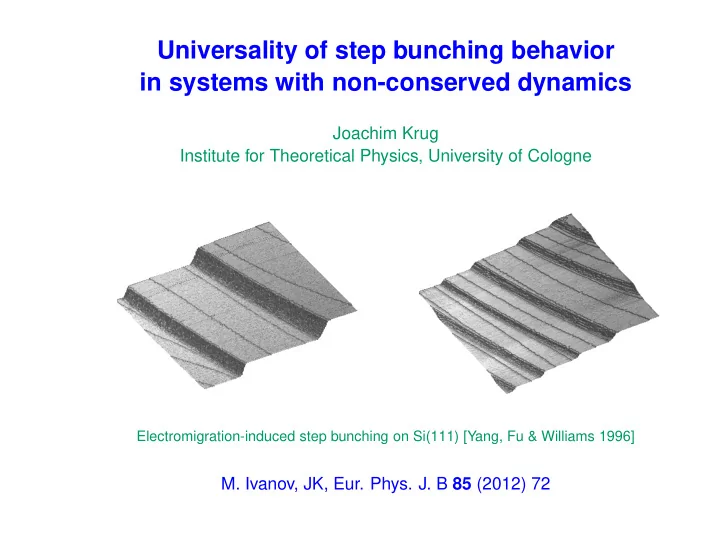
Universality of step bunching behavior in systems with non-conserved - PowerPoint PPT Presentation

Universality of step bunching behavior in systems with non-conserved dynamics Joachim Krug Institute for Theoretical Physics, University of Cologne Electromigration-induced step bunching on Si(111) [Yang, Fu & Williams 1996] M. Ivanov, JK, Eur. Phys. J. B 85 (2012) 72

Electromigration-induced step bunching on Si(111) Courtesy of V. Usov T = 1130 o C

Temperature regimes B.J. Gibbons, S. Schaepe and J.P . Pelz, Surf. Sci. 600 (2006) 2417

Temperature regimes F I 900 C II 1100 C III 1250 C • Regimes I and III: Step bunching for down-step current, consistent with Burton-Cabrera-Frank theory (non-transparent steps) • Regime II: Step bunching for up-step current, requires step transparency or other mechanism

Levels of description D’ • Microscopic (Ehrlich-Schwoebel effect) Ehrlich & Hudda (1966) Energie ∆ E S Schwoebel & Shipsey (1966) 3D discrete ⇓ y j-1 • Mesoscopic (step dynamics) j j+1 Burton, Cabrera & Frank (1951) Stoyanov (1991) 1D discrete x ⇓ h • Macroscopic (continuum theory) Nozières (1987); Pimpinelli et al. (2002) 0D discrete x

BCF-theory with sublimation and surface electromigration • diffusion D and desorption 1 / τ 1/τ D k + k − • asymmetric attachment rates k ± f S. Stoyanov, Jap. J. Appl. Phys. 30 , 1 (1991) electromigration force • stationary diffusion equation for adatom concentration n ( x ) on the terraces: D d 2 n dx 2 − Df dx − n dn D dn dx − Df k B T n | x = x i = ∓ k ± [ n − n eq ] | x = x i τ = 0 b.c.: k B T eq exp [ ∆ µ ( x i ) / k B T ] mit • repulsive step-step interactions: n eq ( x i ) = n 0 �� � 3 � ∆ µ ( x i ) � 3 l l � ≡ g ν i = − g − k B T x i + 1 − x i x i − x i − 1 g : interaction strength l = 1 : mean step spacing

√ D τ diffusion length l D = l ± = D / k ± kinetic lengths • Length scales: ξ = k B T / f electromigration length x Attachment-limited regime: ξ ≫ l D ≫ l ± ≫ l i+1 x i x i−1 dx i � 1 − b ES l i + 1 + b ES � dt = ( 1 + g ν i ) + U ( 2 ν i − ν i + 1 − ν i − 1 )+ R − 1 l i 1 e 2 2 + b el 2 [ { 2 + g ( ν i + ν i + 1 ) } l i −{ 2 + g ( ν i + ν i − 1 ) } l i − 1 ] eq Ω , R e = n 0 , b el = ξ − 1 l 2 with l i = x i + 1 − x i , b ES = l − − l + gl 2 D D , U = τ l − + l + l − + l + l − + l + • Standard approximation: Neglect terms ∼ g ν i because g ∼ | tan θ | 3 ≪ 1

• In the standard approximation the same set of equations describes step bunching by electromigration or ES-effect, in the presence of growth or sublimation Liu & Weeks 1998, JK et al. 2005, Popkov & JK 2005, 2006 dx i � 1 − b l i + 1 + b � + U ( 2 ν i − ν i + 1 − ν i − 1 ) , l i − 1 b = b ES + 2 b el dt = 2 2 • On the level of linear stability analysis, the neglected terms lead to an asymmetry between growth and sublimation Fok et al. 2007; Ivanov et al. 2010 b b 1 1 unstable stable stable 0 0 g g 1 1 6 6 unstable stable − 1 − 1 a ) b ) a) growth: unstable for b < 0 b) sublimation: unstable for b > 6 g • Full equations are conservative for growth but non-conservative for sublimation

Continuum limit of the standard model J.K., V. Tonchev, S. Stoyanov, A. Pimpinelli: Phys. Rev. B 71 , 045412 (2005) dx i dt = 1 − b ( x i + 1 − x i )+ 1 + b x ( x i − x i − 1 ) i+1 x 2 2 i x i−1 + U ( 2 ν i − ν i + 1 − ν i − 1 ) ⇓ h ∂ h ∂ t + ∂ ∂ m ∂ 2 m 2 − b ∂ x + 3 U � � 2 m − 1 = − 1 ∂ x ∂ x 2 6 m 3 2 m m = ∂ h ∂ x > 0 x

Continuum limit of the standard model J.K., V. Tonchev, S. Stoyanov, A. Pimpinelli: Phys. Rev. B 71 , 045412 (2005) dx i dt = 1 − b ( x i + 1 − x i )+ 1 + b x ( x i − x i − 1 ) i+1 x 2 2 i x i−1 + U ( 2 ν i − ν i + 1 − ν i − 1 ) ⇓ h ∂ h ∂ t + ∂ ∂ m ∂ 2 m 2 − b ∂ x + 3 U � � 2 m − 1 = − 1 ∂ x ∂ x 2 6 m 3 2 m m = ∂ h ∂ x > 0 x • destabilizing

Continuum limit of the standard model J.K., V. Tonchev, S. Stoyanov, A. Pimpinelli: Phys. Rev. B 71 , 045412 (2005) dx i dt = 1 − b ( x i + 1 − x i )+ 1 + b x ( x i − x i − 1 ) i+1 x 2 2 i x i−1 + U ( 2 ν i − ν i + 1 − ν i − 1 ) ⇓ h ∂ h ∂ t + ∂ ∂ m ∂ 2 m 2 − b ∂ x + 3 U � � 2 m − 1 = − 1 ∂ x ∂ x 2 6 m 3 2 m m = ∂ h ∂ x > 0 x • destabilizing • stabilizing

Continuum limit of the standard model J.K., V. Tonchev, S. Stoyanov, A. Pimpinelli: Phys. Rev. B 71 , 045412 (2005) dx i dt = 1 − b ( x i + 1 − x i )+ 1 + b x ( x i − x i − 1 ) i+1 x 2 2 i x i−1 + U ( 2 ν i − ν i + 1 − ν i − 1 ) ⇓ h ∂ h ∂ t + ∂ ∂ m ∂ 2 m 2 − b ∂ x + 3 U � � 2 m − 1 = − 1 ∂ x ∂ x 2 6 m 3 2 m m = ∂ h ∂ x > 0 x • destabilizing • stabilizing • symmetry breaking

The shape of step bunches V. Popkov, JK, Europhys. Lett. 72 , 1025 (2005) h • Ansatz for moving step bunches: (S. Stoyanov) Ω h ( x , t ) = φ ( x − Vt ) − Ω t V • sum rule from mass conservation: Ω + V = 1 x ⇒ ODE for surface profile φ ( ξ ) and slope profile m = d φ / d ξ Ω ( ξ + ξ 0 − φ )+ b − m ′ 6 m 3 + 3 U � � 1 − 1 2 m ( m 2 ) ′′ = 0 , ξ = x − Vt m 2 produces solutions with speed V ∼ 1 / N for bunches containing N steps

Comparison to discrete step dynamics 120 80 100 h( ξ ) 40 0 0 20 0 0 40 80 120 ξ • asymmetry between inflow and outflow region

Experimental bunch shapes V. Usov, C.O. Coileain, I.V. Shvets, PRB 83 (2011) 155321 • Electromigration on Si(111) in regime III, T=1270 ◦ C

Scaling laws A. Pimpinelli et al., PRL 88, 206103 (2002) • height and width: L N ∼ W α , α > 1 • minimal terrace size: l min ∼ W / N ∼ N − ( 1 − 1 / α ) N • coarsening: W N ∼ L ∼ t β Results of continuum analysis for moving bunches: α = 3 • W ≈ 4 . 1 × ( UN / b ) 1 / 3 , l min ≈ 2 . 37 × ( U / bN 2 ) 1 / 3 ⇒ • width of the first terrace in the bunch: l 1 ≈ ( 2 U / bN ) 1 / 3 • bunch motion only affects non-dimensional numerical prefactors

Scaling behavior in electromigration experiments V. Usov, C.O. Coileain, I.V. Shvets, PRB 83 (2011) 155321 • Maximal slope y m = 1 / l min ∼ N 2 / 3 , f 1 / 3

Conserved and non-conserved dynamics • Step equations of motion in the standard approximation are conservative, N − 1 ∑ i ˙ x i ≡ R e , but the full equations are not: dx i � 1 − b ES l i + 1 + b ES � dt = ( 1 + g ν i ) + U ( 2 ν i − ν i + 1 − ν i − 1 )+ R − 1 l i − 1 e 2 2 + b el 2 [ { 2 + g ( ν i + ν i + 1 ) } l i −{ 2 + g ( ν i + ν i − 1 ) } l i − 1 ] • Moreover, the non-conserved terms induced by electromigration and ES- effect have different structures. • Does this difference survive on the continuum level? • How does non-conservation affect dynamical features such as coarsening of step bunches?

Continuum equation with non-conserved terms M. Ivanov, JK, Eur. Phys. J. B 85 (2012) 72 • The general form of the continuum equation reads � m ′ m 2 � ′′ m 2 � ′ � � ∂ h ∂ t + ∂ 6 m 3 + 3 U + 1 = 3 g � ′ − 3 gm 2 − m ′ � � − Φ b − J b ∂ x 2 m 6 m 3 2 2 � 1 � ′ J b = 2 b el + b ES Φ b = 3 gb ES ( m 2 ) ′ − 3 gb el m ′ , 2 m 2 m 2 • Analysis of moving bunch solutions in the absence of the red terms suggests upper bounds on bunch slope and bunch wavelength M. Ivanov, V. Popkov, JK, PRE 82 (2010) 011606 • To explore non-linear step dynamics in the general case we turn to numerical simulations of the discrete step equations

Numerical study of non-conserved step equations • Simulation of M = 40 − 80 steps with periodic boundary conditions • Two types of initial conditions: – single large bunch – perturbed regular step train • Parameter ranges: model I: b el = 0 , b ES ∈ [ 0 , 1 ] , U ∈ [ 0 , 1 ] , g ∈ [ 0 , 1 ] Ivanov, Popkov, JK 2010 model II: b ES = 0 , b el ∈ [ 0 , 0 . 5 ] , U ∈ [ 0 , 0 . 5 ] , g ∈ [ 0 , 0 . 1 ] Ivanov, JK 2012 • New phenomena associated with non-conservation: – breakup of large bunches – arrested coarsening – periodic or chaotic switching between different numbers of bunches – dependence of final state on the initial condition

Breakup of step bunches: Step trajectories -60 b) -80 -100 x ˜ -120 -140 -160 -180 11280 11300 11320 11340 11360 11380 11400 11420 11440 11460 time Model II: 80 steps, b el = 0 . 7 , U = g = 0 . 05

Breakup of step bunches: Height profile 160 c) 150 140 130 h 120 110 100 6000 t.u. 11500 t.u. 90 13000 t.u. 15000 t.u. 80 -150 -100 -50 0 50 x ˜ Model II: 80 steps, b el = 0 . 7 , U = g = 0 . 05

Arrested coarsening from random initial conditions: Maximal slope 8 b) 7 6 m max 5 4 3 2 1 0 0 1000 2000 3000 4000 5000 6000 7000 8000 time Model II: 40 steps, b el = 0 . 35 , U = 0 . 2 , g = 0 . 0 , 0 . 01 , 0 . 02 , 0 . 05 , 0 . 09

Comparison of phase diagrams 0.5 a) 0.4 b el 0.3 0.2 lin. stab. 0.1 1 bunch 1 or 2 bunches 2 bunches 0 0 0.01 0.02 0.03 0.04 0.05 0.06 0.07 0.08 0.09 0.1 g Model II: 40 steps, U = 0 . 2 , fluctuating initial conditions

Comparison of phase diagrams 1 0.9 a) 0.8 0.7 0.6 b 0.5 0.4 0.3 lin. stab. 1 bunch 0.2 1/2 bunches 1 or 2 bunches 0.1 2 bunches 0 0 0.01 0.02 0.03 0.04 0.05 0.06 0.07 0.08 0.09 0.1 g Model I: 40 steps, U = 0 . 2 , fluctuating initial conditions