Second order the order of the differential The family of solutions - PowerPoint PPT Presentation

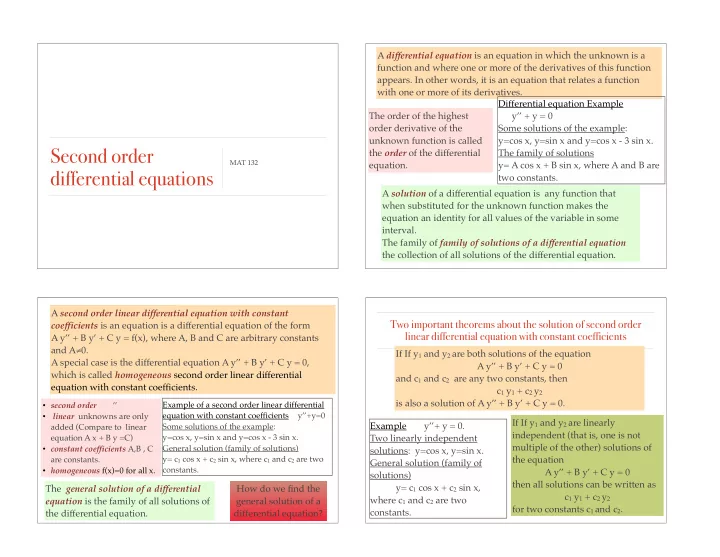
A differential equation is an equation in which the unknown is a function and where one or more of the derivatives of this function appears. In other words, it is an equation that relates a function with one or more of its derivatives. Differential equation Example � The order of the highest � y’’ + y = 0 � order derivative of the Some solutions of the example: � unknown function is called y=cos x, y=sin x and y=cos x - 3 sin x. � Second order the order of the differential The family of solutions � MAT 132 � equation. y= A cos x + B sin x, where A and B are differential equations two constants. A solution of a differential equation is any function that when substituted for the unknown function makes the equation an identity for all values of the variable in some interval. � The family of family of solutions of a differential equation the collection of all solutions of the differential equation. A second order linear differential equation with constant Two important theorems about the solution of second order coefficients is an equation is a differential equation of the form � linear differential equation with constant coefficients A y’’ + B y’ + C y = f(x), where A, B and C are arbitrary constants and A ≠ 0. � If If y 1 and y 2 are both solutions of the equation � A special case is the differential equation A y’’ + B y’ + C y = 0, A y’’ + B y’ + C y = 0 � which is called homogeneous second order linear differential and c 1 and c 2 are any two constants, then � equation with constant coefficients. c 1 y 1 + c 2 y 2 � is also a solution of A y’’ + B y’ + C y = 0. • second order ’’ � Example of a second order linear differential • linear unknowns are only equation with constant coefficients � y’’+y=0 � If If y 1 and y 2 are linearly Example � y’’+ y = 0. � Some solutions of the example: � added (Compare to linear independent (that is, one is not y=cos x, y=sin x and y=cos x - 3 sin x. � Two linearly independent equation A x + B y =C) � � multiple of the other) solutions of • constant coefficients A,B , C General solution (family of solutions) � solutions: y=cos x, y=sin x. � y= c 1 cos x + c 2 sin x, where c 1 and c 2 are two the equation � are constants. � General solution (family of • homogeneous f(x)=0 for all x. constants. A y’’ + B y’ + C y = 0 � solutions) � then all solutions can be written as � y= c 1 cos x + c 2 sin x, � The general solution of a differential How do we find the c 1 y 1 + c 2 y 2 � where c 1 and c 2 are two equation is the family of all solutions of general solution of a for two constants c 1 and c 2 . constants. the differential equation. differential equation?

More about the solution of second order linear differential Case I: Characteristic equation with different (real) roots equation with constant coefficients Example � y’’- 5 y’+ 6y = 0. � The equation � 1. y’’- 5 y’+ 6y = 0. � Find all solutions of the above equation, that can be written as � A x 2 + B x + C = 0 � y= c 1 e rx , � 2.y’’- 4 y’+ 4y = 0. � where r is a real number. is the characteristic equation 3. y’’- 4 y = 0. � associated to the differential If If y 1 and y 2 are linearly independent If the roots r 1 and r 2 of the 4. 9y’’+1=0 � (that is, one is not a multiple of the characteristic equation � equation � 5. y’’+3 y’+ 3 y = 0. � other) solutions of the equation � A x 2 + B x + C = 0 � A y’’ + B y’ + C y = 0 Find the roots of the are real and different the e r 1 x and e r 2 x A y’’ + B y’ + C y = 0 � are linearly independent solutions of then all solutions can be written as � characteristic equation the equation � c 1 y 1 + c 2 y 2 � in each of the above A y’’ + B y’ + C y = 0. for two constants c 1 and c 2 . cases. If r 1 and r 2 are different (real) solutions of the characteristic equation A x 2 + B x + C = 0 then the general solution of A y’’ + B y’ + C y = 0 is y= c 1 e r1x +c 2 e r2x , where c 1 and c 2 are two constants. Case II: Characteristic equation with repeated roots Case I: Characteristic equation with different (real) roots 1. y’’- 5 y’+ 6y = 0. � The equation � Example Find all solutions of the equation � 2.y’’- 4 y’+ 4y = 0. � A x 2 + B x + C = 0 � y’’- 4 y’+ 4y = 0. � 3. y’’- 4 y = 0. � is the characteristic equation 4.9y’’+1=0 � associated to the differential If If y 1 and y 2 are linearly independent If the characteristic equation � 5.y’’+3 y’+ 3 y = 0. � equation � (that is, one is not multiple of the A x 2 + B x + C = 0 � Find all solutions of each other) solutions of the equation � A y’’ + B y’ + C y = 0 has only one real root r then � of the above equations. A y’’ + B y’ + C y = 0 � e r x and x e r x are linearly If r 1 and r 2 are different (real) solutions of the then all solutions can be written as � independent solutions of the c 1 y 1 + c 2 y 2 � equation � characteristic equation � for two constants c 1 and c 2 . A y’’ + B y’ + C y = 0. A x 2 + B x + C = 0 � If the characteristic equation A x 2 + B x + C = 0 has only one then the general solution of � real root then the general solution of A y’’ + B y’ + C y = 0 is � A y’’ + B y’ + C y = 0 � is y= c 1 e rx +c 2 x e rx , where c 1 and c 2 are two constants. is y= c 1 e r1x +c 2 e r2x , where c 1 and c 2 are two constants.

Summary on solving the linear second order homogeneous Case III: Characteristic equation with complex roots differential equation Example 1 Find all the solutions of the equation y’’+3 y’+ 3 y = 0. � Example 2 Find all solutions of the equation 9y’’+1 = 0. roots of the characteristic General solution If the roots of the characteristic If If y 1 and y 2 are linearly independent To find the general solution polynomial equation � (that is, one is not multiple of the of the differential equation A x 2 + B x + C = 0 � other) solutions of the equation � A y’’ + B y’ + C y = 0 we two distinct real are the complex numbers r 1 = α +i 𝛾 and Δ >0 c A y’’ + B y’ + C y = 0 � consider the characteristic roots r r 2 = α - i 𝛾 then e α x cos( 𝛾 x) and e α x then all solutions can be written as � equation: � sin( 𝛾 x) are linearly independent c 1 y 1 + c 2 y 2 � A x 2 + B x + C = 0 � two complex roots e solutions of the equation � Δ <0 for two constants c 1 and c 2 . Set Δ = B 2 - 4 A C. α +i 𝛾 and α - i 𝛾 sin( 𝛾 x)) A y’’ + B y’ + C y = 0. If the roots of the characteristic equation A x 2 + B x + C = 0 are the one double real Δ =0 c complex numbers r 1 = α +i 𝛾 and r 2 = α - i 𝛾 then the general solution of root r A y’’ + B y’ + C y = 0 is y= e α x (c 1 cos( 𝛾 x) + c 2 sin( 𝛾 x)) � is where c 1 and c 2 are two constants. Solving initial value problems 1. Solve the initial-value problem y’’ + 2 y’ + y=0, y(0)=1, y(1)=3. � 2. 2y’’+5y+3y=0, y(0)=3, y’(0)=-4.