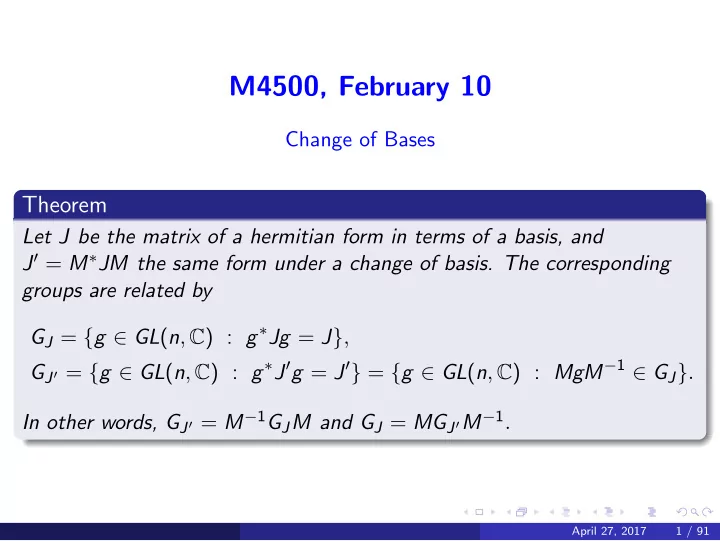
M4500, February 10 Change of Bases Theorem Let J be the matrix of - PowerPoint PPT Presentation

M4500, February 10 Change of Bases Theorem Let J be the matrix of a hermitian form in terms of a basis, and J ′ = M ∗ JM the same form under a change of basis. The corresponding groups are related by G J = { g ∈ GL ( n , C ) : g ∗ Jg = J } , G J ′ = { g ∈ GL ( n , C ) : g ∗ J ′ g = J ′ } = { g ∈ GL ( n , C ) : MgM − 1 ∈ G J } . In other words, G J ′ = M − 1 G J M and G J = MG J ′ M − 1 . April 27, 2017 1 / 91

O (1 , 1) These are the 2 × 2 matrices of determinant 1 satisfying g T J = Jg − 1 : � a � � 1 � � 1 � � d � c 0 0 − b · = · . b d 0 − 1 0 − 1 − c a The resulting equations are a = d , b = c and a 2 − b 2 = 1 . There are four types of matrices in O (1 , 1) , the first and last are in SO (1 , 1) . � cosh t � � − cosh t � � cosh t � � sinh t sinh t − sinht , , , sinh t cosh t sinh t − cosh t − sinh t cosh t So O (1 , 1) has four connected components, SO (1 , 1) has two. The same happens for O ( p , q ) , though somewhat harder to prove. April 27, 2017 2 / 91

Coordinates for SL (2 , R ) The Euler Angles show that SO (3) is connected, and the analogues for SU (2) do the same. The HW problem indicates how this might generalize to SO ( n ) and SU ( n ) . We do this for SL (2 , R ). � 1 � Let e 1 = . Then 0 � � 1 �� x Stab SL (2 , R ) ( e 1 ) = n ( x ) := . 0 1 � a � � 1 � � a � b Let g = ∈ SL (2 , R ) . Then g · = � = 0 . c d 0 c � a � � r cos θ � An arbitrary nonzero vector has polar coordinates = , . We c r sin θ can write � cos θ � � r � � 1 � � 1 � � a � � 1 � − sin θ 0 · · := r ( θ ) · a ( r ) · = = g · . r − 1 sin θ cos θ 0 0 0 c 0 April 27, 2017 3 / 91

So g − 1 · r ( θ ) · a ( r ) ∈ Stab SL (2 , R ) ( e 1 ) , and any g is of the form g − 1 r ( θ ) a ( r ) = n ( x ) ⇐ ⇒ g = n ( x ) a ( r − 1 ) r ( − θ ) . These are the coordinates we need to show SL (2 , R ) is connected. April 27, 2017 4 / 91

O ( n , 1) � A T � � I � � A � � I � C T 0 B 0 · · = B T D T 0 − I C D 0 − I yields the equation B T · B − D T · D = − 1 . In turn this is n = d 2 − 1 . B T B = D T D − I ⇐ ⇒ b 2 1 + · · · + b 2 So either d ≥ 1 or d ≤ − 1 . If a path γ : [0 , 1] ← → O ( n , 1) , � A ( t ) � B ( t ) γ ( t ) = C ( t ) D ( t ) goes from γ (0) = I n +1 × n +1 to some g , D ( t ) must stay ≥ 1 . Any path that contains a matrix with d ≤ − 1 , must stay that way. So there are at least 4 components, det = ± 1 and d ≥ 1 , d ≤ − 1 . Analogues of Euler Angles show that O ( n , 1) has exactly 4 components. April 27, 2017 5 / 91

Exercise 3.17 Compute         cos θ 1 − sin θ 1 0 cosh t 0 sinh t cos θ 2 − sin θ 2 0 0   ·   ·   ·   . sin θ 1 cos θ 1 0 0 1 0 sin θ 2 cos θ 2 0 0 0 0 1 cosh t 0 sinh t 0 0 1 1 April 27, 2017 6 / 91

Exercise 3.18 Compute � cosh t � sinh t r ( θ 1 ) · · r ( θ 2 ) . sinh t cosh t April 27, 2017 7 / 91

February 15 Connected component of the Identity Let G 0 ⊂ G be the set of points that can be connected to I by a continuous path. Say γ ( t ) , µ ( t ) ∈ G such that γ (0) = I , γ (1) = A and µ (0) = I , µ (1) = B . Then look at ρ ( t ) := [ γ ( t )] − 1 . It joins I with A − 1 . � µ ( t ) 0 ≤ t ≤ 1 ρ ( t ) = A − 1 γ ( t − 1) AB 1 ≤ t ≤ 2 joins I with AB ; so does ρ ( t ) = γ ( t ) · µ ( t ) . ρ ( t ) = g γ ( t ) g − 1 joins I with gAg − 1 . You can use these path to show that G 0 is a normal subgroup. The main point is that multiplication and inverses produce continuous paths out of continuous paths. April 27, 2017 8 / 91

Exp and Log . To show that log ◦ exp and exp ◦ log are the identity, it is enough to do it for R . For exp ◦ log you need to substitute one series into the other; then �� � n 1 ≤ m ( − 1) m +1 ( x − 1) m � m collect powers of ( x − 1) in . n ! 0 ≤ n The coefficient of ( x − 1) 0 = 1 is 1. The coefficient of x − 1 is 1. The higher powers have coefficient 0. The answer is 1 + ( x − 1) = x . It is VERY awkward to equate the coefficients. We can argue as follows: Within the radius of convergence, substituting and equating coefficients is justified to get the series for the composition of the functions. The Taylor series for exp and log are the listed ones. We know that exp and log are inverse to each other, therefore e log x = x = 1 + ( x − 1) and log e X = X . These facts imply the answer for the coefficients. The calculation for x and X matrices is formally the same, so the answer follows. April 27, 2017 9 / 91

February 17,2017 Quaternion Algebra Definition Q := { v = x 0 + x 1 i + x 2 j + x 3 k } / { i 2 = j 2 = k 2 = ijk = − 1 } Addition is done coordinate by coordinate, and multiplication is ( x 0 + x 1 i + x 2 j + x 3 k ) · ( y 0 + y 1 i + y 2 j + y 3 k ) = = ( x 0 y 0 − x 1 y 1 − x 2 y 2 − x 3 y 3 ) + ( x 0 y 1 + x 1 y 0 + x 2 y 3 − x 3 y 2 ) i + + ( x 0 y 2 + x 2 y 0 + x 3 y 1 − x 1 y 3 ) j + ( x 0 y 3 + x 3 y 0 + x 1 y 2 − x 2 y 1 ) k , distributive and associative otherwise. The algebra has a conjugation, x 0 + x 1 i + x 2 j + x 3 k = x 0 − x 1 i − x 2 j − x 3 k and a norm | v | 2 := v · v = x 2 0 + x 2 1 + x 2 2 + x 2 3 . April 27, 2017 10 / 91

Exercise. Show that every nonzero element has an inverse. The bracket (as for any associative algebra) is Lie Algebra. [ v 1 , v 2 ] := v 1 v 2 − v 2 v 1 :   i j k   2( x 2 y 3 − y 2 x 3 ) i − 2( x 1 y 3 − y 1 x 3 ) j + 2( x 1 y 2 − y 1 x 2 ) k = det x 1 x 2 x 3 y 1 y 2 y 3 the cross product. So g := { x 1 i + x 2 j + x 3 k } forms a Lie subalgebra, in fact a Lie ideal. Exponential Map. Define � v n exp( v ) := n ! . 0 ≤ n You can ignore the x 0 ; it commutes with everything, and just multiplies the exponential of the remainder by e x 0 . So we may as well restrict attention to g . Since v · v = v · v , 1 = exp(0) = exp( v + v ) = exp( v ) · exp( v ) . Since v = − v for v ∈ g , we can also conclude that exp( g ) consists of elements of norm 1. April 27, 2017 11 / 91

Exercise. What is the image of the exponential map? In the warmup you were asked to show that g ∼ = su (2) and that the elements of norm 1 are equivalent to SU (2) . April 27, 2017 12 / 91

February 22 A two dimensional Lie algebra has a basis { e 1 , e 2 } , and only one relation [ e 1 , e 2 ] = α e 1 + β e 2 . If α = β = 0 , this is the abelian Lie algebra. If not, may as well assume 1 = e 1 + β α � = 0 , by just interchanging the two basis vectors. Then e ′ α e 2 and e ′ 2 = 1 α e 2 are also a basis. The bracket is 2 ] = 1 α [ e 1 + β α e 2 , e 2 ] = 1 α ( α e 1 + β e 2 + 0) = e 1 + β [ e ′ 1 , e ′ α e 2 = e ′ 1 . So there is only one up to equivalence, [ e ′ 1 , e ′ 2 ] = e ′ 1 . Check the 3 − dimensional case, there are more cases, and su (2) as well as sl (2 , R ) have to show up. April 27, 2017 13 / 91

� � 1! X + t 2 2! X 2 + t 3 3! X 3 + t 4 I + t 4! X 4 + . . . · � � 1! Y + t 2 2! Y 2 + t 3 3! Y 3 + t 4 I + t 4! Y 4 + . . . · = � 1 � � 1 � 1! X + 1 2! X 2 1 1!1! XY + 1 + + t 2 2! Y 2 = I + t 1! Y + � 1 � 1 1!2! XY 2 + 1 1 3! X 3 + + t 3 2!1! X 2 Y + 3! Y 3 + . . . April 27, 2017 14 / 91

For a Lie algebra g , you can define a liner map ad X : g − → g , ad X ( Y ) = [ X , Y ] It satisfies ad X ([ Y , Z ]) = [ad X ( Y ) , ad X ( Z )] When g ⊂ M ( n , F ) , is a Lie subalgebra, we have two linear maps, L X : M ( n , F ) − → M ( n , F ) , L X ( Y ) := XY , R X : M ( n , F ) − → M ( n , F ) , R X ( Y ) := YX . Tnen ad X = L X − R X . This has the advantage that L X R Y = R Y L X . Application: e X · Y · e − X = e ad X ( Y ) . April 27, 2017 15 / 91

Differential of Exp For a map F = ( f 1 , . . . , f m ) : R n − → R m , the differential DF is the matrix � ∂ f i � . We can compute it as a linear map at an x 0 ∈ R n : DF := ∂ x j � � DF ( x 0 )( v 0 ) = d � F ( x 0 + tv 0 ) . � dt t =0 We want to do this for F = exp : M ( n , R ) − → M ( n , R ) .   � � � � d 1 e X + tY = �  X k YX n − k − 1  = � dt n ! t =0 n ≥ 0 0 ≤ k ≤ n − 1   � � � L n X − R n 1 1  L k X R n − k − 1  Y = X = Y = X n ! n ! L X − R X n ≥ 0 n ≥ 0 0 ≤ k ≤ n − 1 = e L X − e R X Y = e L X I − e − ad X Y = e X I − e − ad X Y . ad X ad X ad X April 27, 2017 16 / 91

Representations of Lie Algebras and Lie Groups Any linear map into M ( n , R ) = gl ( n , R ) must be of the form φ ( t ) = tA . Two such representations are equivalent if the matrices differ by a change of basis; so this is the same as matrices up to similarity. The Lie group is ( R + , × ) with exponential map t �→ e t . Any representation of the Lie algebra exponentiates Φ( e t ) = e tA . On the other hand, we can consider G = ( S 1 , × ) . There is a dependence on the realization as a matrix group (which disappears if one considers it as a Lie group). � cos θ � � 0 � − sin θ − θ S 1 := { r ( θ ) = } Lie ( G ) = { } . sin θ cos θ θ 0 The Lie algebra is the same as before, realized differently. So the Lie homomorphisms are the same, φ : θ �→ θ A . But for the group, Φ : θ �→ e tA only makes sense if e 2 π A = I . April 27, 2017 17 / 91