Extending a 2 k design The 2 k factorial design can detect main - PowerPoint PPT Presentation

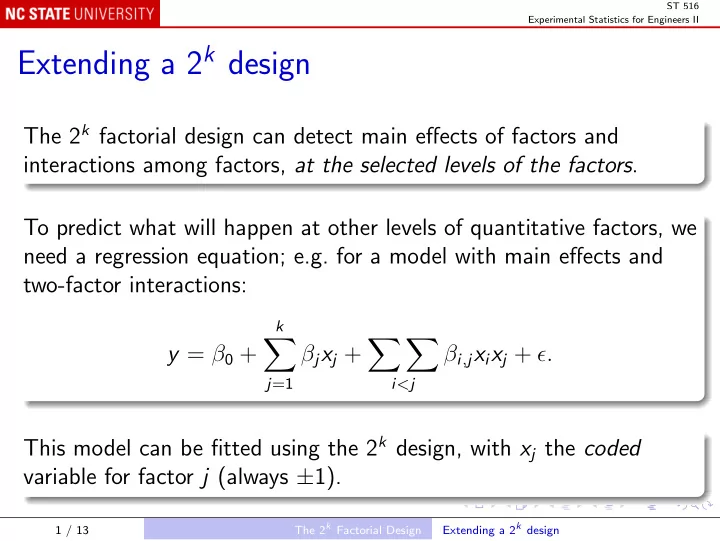
ST 516 Experimental Statistics for Engineers II Extending a 2 k design The 2 k factorial design can detect main effects of factors and interactions among factors, at the selected levels of the factors . To predict what will happen at other levels of quantitative factors, we need a regression equation; e.g. for a model with main effects and two-factor interactions: k � � � y = β 0 + β j x j + β i , j x i x j + ǫ. j =1 i < j This model can be fitted using the 2 k design, with x j the coded variable for factor j (always ± 1). The 2 k Factorial Design Extending a 2 k design 1 / 13

ST 516 Experimental Statistics for Engineers II But: the model is linear in each x j with the other factors held fixed. Cannot detect curvature, e.g. if the true dependence is k k � � � � β j , j x 2 y = β 0 + β j x j + β i , j x i x j + j + ǫ. j =1 i < j j =1 In this case, extra design points are needed. E.g. if all factors are quantitative, n C runs at the center point x 1 = x 2 = · · · = x k = 0. The 2 k Factorial Design Extending a 2 k design 2 / 13

ST 516 Experimental Statistics for Engineers II At the original (2 k factorial) design points, all x 2 j = 1, so � � k k � � � � y = β 0 + + β j x j + β i , j x i x j + ǫ. β j , j j =1 j =1 i < j So ¯ y F , the average y at the original design points, estimates β 0 + � k j =1 β j , j . But clearly ¯ y C , the average y at the center point, estimates β 0 . The 2 k Factorial Design Extending a 2 k design 3 / 13

ST 516 Experimental Statistics for Engineers II y C to test the hypothesis H 0 : � β j , j = 0. So we can use ¯ y F − ¯ This will usually detect quadratic terms (but the test has no power if � β j , j = 0). Test statistic is based on y C ) 2 SS Pure quadratic = n F n C (¯ y F − ¯ , n F + n C where n F is the number of points in the original factorial design. Replicated center values provide n C − 1 degrees of freedom to estimate σ 2 (“pure error”). The 2 k Factorial Design Extending a 2 k design 4 / 13

ST 516 Experimental Statistics for Engineers II Example Filtration rate, with 4 center points (rates 73, 75, 66, 69) R code filt2 <- read.table("data/filtration-with-center.txt", header = TRUE) summary(aov(Rate ~ A * B * C * D + I(A^2) + I(B^2) + I(C^2) + I(D^2), filt2)) summary(aov(Rate ~ A * C + A * D + I(A^2) + I(C^2) + I(D^2), filt2)) The formulas include all squared variables, but, with this design, only one can be estimated. The 2 k Factorial Design Extending a 2 k design 5 / 13

ST 516 Experimental Statistics for Engineers II Full model Df Sum Sq Mean Sq F value Pr(>F) A 1 1870.56 1870.56 115.1115 0.001731 ** B 1 39.06 39.06 2.4038 0.218821 C 1 390.06 390.06 24.0038 0.016273 * D 1 855.56 855.56 52.6500 0.005401 ** I(A^2) 1 1.51 1.51 0.0931 0.780243 A:B 1 0.06 0.06 0.0038 0.954450 A:C 1 1314.06 1314.06 80.8654 0.002903 ** B:C 1 22.56 22.56 1.3885 0.323620 A:D 1 1105.56 1105.56 68.0346 0.003731 ** B:D 1 0.56 0.56 0.0346 0.864273 C:D 1 5.06 5.06 0.3115 0.615686 A:B:C 1 14.06 14.06 0.8654 0.420856 A:B:D 1 68.06 68.06 4.1885 0.133202 A:C:D 1 10.56 10.56 0.6500 0.479099 B:C:D 1 27.56 27.56 1.6962 0.283757 A:B:C:D 1 7.56 7.56 0.4654 0.544069 Residuals 3 48.75 16.25 --- Signif. codes: 0 *** 0.001 ** 0.01 * 0.05 . 0.1 1 The 2 k Factorial Design Extending a 2 k design 6 / 13

ST 516 Experimental Statistics for Engineers II Reduced model Df Sum Sq Mean Sq F value Pr(>F) A 1 1870.56 1870.56 99.7122 1.830e-07 *** C 1 390.06 390.06 20.7927 0.0005354 *** D 1 855.56 855.56 45.6066 1.356e-05 *** I(A^2) 1 1.51 1.51 0.0806 0.7809238 A:C 1 1314.06 1314.06 70.0474 1.359e-06 *** A:D 1 1105.56 1105.56 58.9331 3.502e-06 *** Residuals 13 243.88 18.76 --- Signif. codes: 0 *** 0.001 ** 0.01 * 0.05 . 0.1 1 The 2 k Factorial Design Extending a 2 k design 7 / 13

ST 516 Experimental Statistics for Engineers II The “Residuals” line in the reduced model may be decomposed into “Lack of Fit” and “Pure Error”: summary(aov(Rate ~ A * C + A * D + I(A^2) + I(C^2) + I(D^2) + factor(A):factor(B):factor(C):factor(D), filt2)) Output Df Sum Sq Mean Sq F value Pr(>F) A 1 1870.56 1870.56 115.1115 0.001731 ** C 1 390.06 390.06 24.0038 0.016273 * D 1 855.56 855.56 52.6500 0.005401 ** I(A^2) 1 1.51 1.51 0.0931 0.780243 A:C 1 1314.06 1314.06 80.8654 0.002903 ** A:D 1 1105.56 1105.56 68.0346 0.003731 ** factor(A):factor(B):factor(C):factor(D) 10 195.13 19.51 1.2008 0.494185 Residuals 3 48.75 16.25 --- Signif. codes: 0 *** 0.001 ** 0.01 * 0.05 . 0.1 1 The 2 k Factorial Design Extending a 2 k design 8 / 13

ST 516 Experimental Statistics for Engineers II The line for factor(A):factor(B):factor(C):factor(D) is Lack of Fit, with 10 d.f. pooled from the omitted terms. The residuals line is now Pure Error, with the 3 d.f. from the 4 center point runs. The 2 k Factorial Design Extending a 2 k design 9 / 13

ST 516 Experimental Statistics for Engineers II Central Composite Design Adding center points allows you to detect pure quadratic terms, but not to estimate them. Need to add more points; e.g. axial points: x 1 = ± α, x 2 = · · · = x k = 0, x 1 = 0 , x 2 = ± α, x 3 = · · · = x k = 0, and so on; 2 k extra points when unreplicated (usual case). Choice of α ( n F = number of factorial runs): α = 1 convenient, as it brings in no new levels; √ n F gives desirable property (rotatability). α = 4 The 2 k Factorial Design Extending a 2 k design 10 / 13

ST 516 Experimental Statistics for Engineers II Center Points with Qualitative Factors For a qualitative factor (e.g. equipment from two suppliers), x = 0 is infeasible. Use two center points, one each at the high and low levels of the qualitative factor. Same as axial points with α = 1, but usually replicated. The 2 k Factorial Design Extending a 2 k design 11 / 13

ST 516 Experimental Statistics for Engineers II Coded Variables and Engineering Units A quantitative factor x ∗ has levels x ∗ − and x ∗ + . The coded variable is x = x ∗ − 1 � � x ∗ + + x ∗ 2 − . 1 2 ( x ∗ − ) + − x ∗ Regression equation in coded variables can be converted to engineering units for later use, e.g in predicting the response for arbitrary levels. The 2 k Factorial Design Extending a 2 k design 12 / 13

ST 516 Experimental Statistics for Engineers II The same equation results from fitting directly to the original factor levels. Coded variable form is useful for the experimenter: Gives all effects and interactions; The t -statistics are equivalent to F -statistics in the ANOVA table. Engineering units form is useful for others: Does not depend on experimental levels of factors; Coefficients have a different interpretation: a regression coefficient represents the effect of changing a factor by 1 (engineering) unit, not the effect of changing from low level to high level (actually, one-half that effect). The 2 k Factorial Design Extending a 2 k design 13 / 13