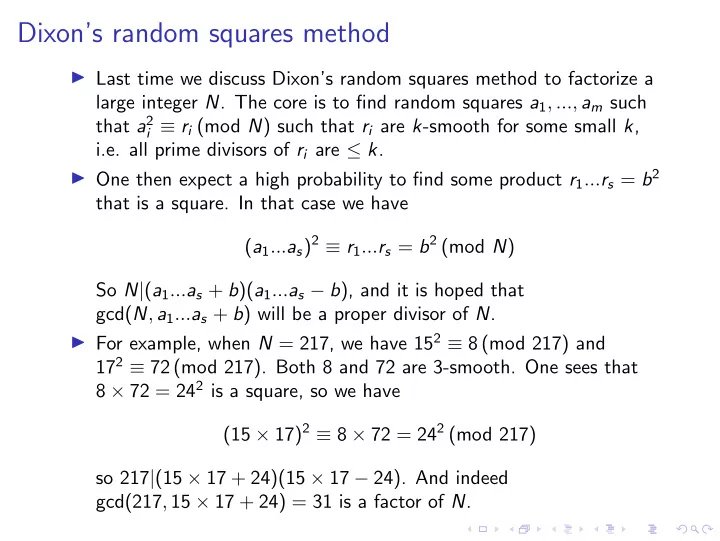

Dixon’s random squares method ◮ Last time we discuss Dixon’s random squares method to factorize a large integer N . The core is to find random squares a 1 , ..., a m such that a 2 i ≡ r i (mod N ) such that r i are k -smooth for some small k , i.e. all prime divisors of r i are ≤ k . ◮ One then expect a high probability to find some product r 1 ... r s = b 2 that is a square. In that case we have ( a 1 ... a s ) 2 ≡ r 1 ... r s = b 2 (mod N ) So N | ( a 1 ... a s + b )( a 1 ... a s − b ), and it is hoped that gcd( N , a 1 ... a s + b ) will be a proper divisor of N . ◮ For example, when N = 217, we have 15 2 ≡ 8 (mod 217) and 17 2 ≡ 72 (mod 217). Both 8 and 72 are 3-smooth. One sees that 8 × 72 = 24 2 is a square, so we have (15 × 17) 2 ≡ 8 × 72 = 24 2 (mod 217) so 217 | (15 × 17 + 24)(15 × 17 − 24). And indeed gcd(217 , 15 × 17 + 24) = 31 is a factor of N .
Products to be squares ◮ Now comes the question: suppose we have a bunch of r 1 , r 2 , ... r m that are all k -smooth, i.e. having only prime factors among p 1 , ..., p n ≤ k . We can write p e 11 1 p e 12 2 ... p e 1 n r 1 = n p e 21 1 p e 22 2 ... p e 2 n r 2 = n ... p e m 1 1 p e m 2 2 ... p e mn r m = n ◮ Alright, so how do we find a product of the subset of them to be a square? ◮ One easily see that it depends on the quantities e ij for 1 ≤ i ≤ m , 1 ≤ j ≤ n . ◮ The key observation, nevertheless, is that this depends only on the parity of e ij , i.e. e ij mod 2!
Products to be squares, II p e 11 1 p e 12 2 ... p e 1 n r 1 = n p e 21 1 p e 22 2 ... p e 2 n r 2 = n ... p e m 1 1 p e m 2 2 ... p e mn = r m n ◮ Suppose we want to check if a subset S of r 1 , ..., r m has product being a square. Let us define a function f S : { 1 , 2 , ..., m } → { 0 , 1 } to be given by f S ( i ) = 1 if r i is chosen (i.e. r i ∈ S ) and f S ( i ) = 0 if r i �∈ S . ◮ Then what is the product of all r i ∈ S ? It can be expressed as f S ( i ) m m n m n n m n � m r f S ( i ) p e ij p e ij f S ( i ) p e ij f S ( i ) i =1 e ij f S ( i ) � � � � � � � � = = = = p i j j j j i =1 i =1 j =1 i =1 j =1 j =1 i =1 j =1 ◮ When is such a product a square? Well something like 2 a 3 b 5 c is a square iff all a , b , c are even. Likewise, the above is a square if m � e ij f S ( i ) is even, for every j = 1 , 2 , ..., n . i =1
Linear algebra ◮ So our situation is: given integers e ij ∈ Z ≥ 0 , we want to find f S ( i ) - let’s just abbreviate it as f i - which can be 0 or 1, such that m � e ij f i ≡ 0 (mod 2), for every j = 1 , 2 , ..., n . i =1 ◮ In other words, the problem becomes to find solutions to a system of linear congruence equations mod 2, with constants e ij and variables f i . ◮ The condition that f i ∈ { 0 , 1 } is no longer a problem at all, since mod 2 we only have two elements, represented by 0 and 1, anyway. ◮ So how do we solve system of linear congruence equations? ◮ High school situation: � 3 f + 2 g = 7 6 f + 5 g = 16
Linear algebra mod 2 � 3 f + 2 g = 7 6 f + 5 g = 16 ◮ We typically subtract a multiple of an equation from another so that some variable is killed (in the difference). For example, it looks tempting to subtract from second equation twice of the first. ◮ In other words, we look at the coefficients for a : they are 3 and 6. We subtract 6 / 3 times the first equation to cancel the a -part of the second. ◮ How do we do this mod 2? In fact, we can do the same mod p , because F p is a field! We can divide things like in the rational or real numbers. And thus all those procedures of solving linear systems works the same mod p . ◮ When p = 2, it’s even better cause ... division in F 2 is extremely simple. The only possible divisor - the non-zero element - is 1, and dividing by it does nothing.
Linear algebra mod 2, II ◮ Let us look at an example, suppose we have r 1 = 30, r 2 = 60, r 3 = 10 and r 4 = 24. We rewrite 2 1 · 3 1 · 5 1 r 1 = 2 2 · 3 1 · 5 1 r 2 = 2 1 · 3 0 · 5 1 r 3 = 2 3 · 3 1 · 5 0 r 4 = ◮ To find r f 1 1 ... r f 4 4 to be a square, that is to solve f 1 + 2 f 2 + f 3 + 3 f 4 ≡ 0 (mod 2) f 1 + f 2 + f 4 ≡ 0 (mod 2) f 1 + f 2 + f 3 ≡ 0 (mod 2) ◮ For those of you familiar with matrices, we are looking at transpose 1 1 1 f 1 1 2 1 3 1 2 1 3 2 1 1 f 2 , solving = 1 1 0 1 1 1 0 1 ≡ 0 (mod 2) 1 0 1 f 3 1 1 1 0 1 1 1 0 3 1 0 f 4
Linear algebra mod 2, III f 1 + 2 f 2 + f 3 + 3 f 4 0 (mod 2) ≡ f 1 + f 2 + f 4 0 (mod 2) ≡ f 1 + f 2 + f 3 0 (mod 2) ≡ ◮ Working mod 2, we can rewrite it mod 2 as f 1 + f 3 + f 4 ≡ 0 (mod 2) f 1 + f 2 + f 4 ≡ 0 (mod 2) f 1 + f 2 + f 3 ≡ 0 (mod 2) ◮ Now suppose we want to cancel the coefficients for f 1 , we can subtract the first equation from the second and third. Noting that 1 − 1 = 0 and 0 − 1 ≡ 1 (mod 2), we have f 1 + f 3 + f 4 ≡ 0 (mod 2) + f 2 + f 3 ≡ 0 (mod 2) + f 2 + f 4 ≡ 0 (mod 2) ◮ Analogously subtract second from the third we havee + + ≡ 0 (mod 2) f 1 f 3 f 4 + + ≡ 0 (mod 2) f 2 f 3 + + ≡ 0 (mod 2) f 3 f 4
Linear algebra mod 2, IV f 1 + f 3 + f 4 0 (mod 2) ≡ + f 2 + f 3 0 (mod 2) ≡ + f 3 + f 4 0 (mod 2) ≡ ◮ Now we can plug in values: say f 4 = 1. Then f 3 ≡ 1 by the third equation. And then f 2 ≡ 1 by the second equation, and finally f 1 ≡ 0 by the first. ◮ Hence for our original r 1 = 30, r 2 = 60, r 3 = 10 and r 4 = 24, we conclude that the product of the last three - excluding r 1 - is a square. ◮ Indeed, 60 × 10 × 24 = 2 6 · 3 2 · 5 2 is a square. ◮ In the scenario above, the leading variables in each equation - f 1 , f 2 and f 3 - are called pivot variables. ◮ They are such that the pivot variable does not appear in latter equations. ◮ The rest non-pivot variables can be arbitrarily assigned values, after which each equation will impose a unique value for its pivot variable, therefore solving the equation. ◮ The same works over any field, in particular F p , just that you need to compute things like 6 / 3 when canceling equations.
Recommend
More recommend