
1 Dense matter QFT with the DS L=24 1e-08 LLR L=24 O( µ) DS L=22 density-of-states DS L=20 DS L=18 DS L=16 LLR L=22 LLR L=20 method LLR L=18 LLR L=16 1e-16 0 0.5 1 1.5 2 µ Kurt Langfeld, Centre of Mathematical Sciences, Plymouth University Sign 2015, Institute for Nuclear Research, Debrecen, 1st October 2015 Nicolas Garron, Plymouth University Biagio Lucini, Swansea University Roberto Pellegrini, Edinburgh University Antonio Rago, Plymouth University
Overview: What do we know about QFTs with a sign problem? Promising algorithmic attempts [Complex Langevin, Lefschetz Thimble, strong coupling methods, ….] Here: density-of-states method, dualisation (bench marking) Foundations of the LLR-approach [the U(1) showcase] Can we solve a strong sign problem with the LLR method? [ yes! Theory & the Z3 showcase]
Overview: Anatomy of a sign problem: Heavy-Dense QCD (HDQCD) Theory : particle-hole duality the Inverse-Silver-Blaze feature [new physics?] Results from re-weighting [regions of strong-sign-problem] Results from the density-of-states approach (LLR method)
Phases of QCD
What did we know in 2003 from 1st principles? D’
What do we know NOW from 1st principles? D’
What do we NOT know from 1st principles? chiral spirals
What is the problem?
How can we quantify the problem? If we drop the imaginary part of the action: Z Z PQ ( µ ) = D φ exp { S R [ φ ] } Define the overlap between full and phase quenched theory Z ( µ ) O ( µ ) = Z PQ ( µ ) = h exp { iµS I } i PQ Standard re-weighting: standard MC h A i = h A exp { iS I } i PQ h exp { iS I } i PQ
Overlap problem: and have different free energy densities Z Z PQ Z ( µ ) ⇒ can be very small O ( µ ) = Z PQ ( µ ) = exp { − ∆ f V } ( ∆ f > 0) re-weighting is inefficient! Silver Blaze Problem: [low temperatures, large volumes] depends on only for: Z ( µ ) µ > m threshold µ not satisfied by Z PQ ( µ ) ! [Tom Cohen, 2003]
…and a simple, but important identity: ∂ ln Z ( µ ) Recall the definition of the density : ρ ( µ ) = T ∂ µ V 3 Z ( µ ) Trivially: Z ( µ ) = Z PQ ( µ ) Z PQ ( µ ) = O ( µ ) Z PQ ( µ ) ∂ ρ ( µ ) = T ∂ µ ln O ( µ ) + ρ PQ ( µ ) V 3 Silver > 0 neglectet 0 Blaze Problem µ < m threshold
Promising attempts to solve QFTs with sign problems: [this is not a complete list!]
LLR Approach Target: density-of-states Z Partition function: Z = dE ρ ( E ) exp { β E } Wang-Landau type algorithm: 1. Divide action range in intervals 2. Generate configurations for each interval 3. Generate action histogram
Wang-Landau type algorithm: 4. Include as re-weighting ρ − 1 factor 5. re-fine until histogram is flat ρ 6. patch together the from ρ intervals to the overall density [Wang, Landau, PRL 86 (2001) 2050] Advantage: solves overlap problems! Ideal for systems with discrete action range (spin systems) Disadvantage: histogram edge effects for continuous systems
LLR approach: For small enough : Poisson δ E distribution 1 Z e − aS [ φ ] D φ θ [ E k , δ E ] ( S [ φ ]) W [ φ ] h h W [ φ ] i i k ( a ) = N k re-weighting standard MC observable restriction to the factor average action range need to find “a” !
LLR approach: Choose: ∆ E = S [ φ ] − E k − δ E/ 2 If “a” is correct, the distribution is flat implying non-linear stochastic h h ∆ E i i k ( a ) = 0 equation Use e.g. Newton Raphson to solve for “a”: a n +1 = a n + 12 δ E 2 h h ∆ E i i ( a n ) [Langfeld, Lucini, Rago, PRL 109 (2012) 111601]
[Langfeld, Lucini, Rago, PRL 109 (2012) 111601] LLR approach: Reconstruct the density-of-states: N − 1 ! Y e a N ( E − E N ) e a k δ E ρ ( E ) = ρ 0 k =1 N ( E ) : E N ≤ E < E N +1 Features: •2nd order accuracy: O ( δ E 2 ) •Exponential error suppression ! [Langfeld, Lucini, Pellegrini, Rago, arXiv:1509.08391]
LLR approach: Indicative result: SU(2) & SU(3) gauge theories E max = 60 , 000
LLR approach: Technical progress: Different versions of the iteration might improve convergence i k ( a ( n ) ) h h ∆ E i a ( n +1) = a ( n ) + σ 2 ( ∆ E ; a ( n ) ) [Gattringer, Toerek, PLB 747 (2015) 545] Solving the stochastic equation: h h ∆ E i i k ( a ) Statistical noise interferes with convergence Robbins-Monro (1951) under-relaxation: a ( n +1) = a ( n ) + 1 12 i k ( a ( n ) ) δ E 2 h h ∆ E i max( n − n th , 1) converges to the true solution “a”! [Pellegrini, Langfeld, Lucini, Rago, PoS LATTICE2014 (2015) 229]
LLR approach: Early objections 1. Is the LLR approach ergodic? Use the Replica Exchange method: Use the LLR estimate for as re-weighting factor ρ D φ ρ − 1 ( S [ φ ) W [ φ ] ρ ( S [ φ ) e β S R Yes! random walk observable in configuration space [Langfeld, Lucini, Pellegrini, Rago, arXiv:1509.08391]
LLR approach: Indicative result for U(1) (more later) 0.67 LLR 0.66 Multicanonical 0.65 0.64 〈 E 〉 0.63 0.62 (credits to RP & AR) 0.61 0.6 1.006 1.008 1.01 1.012 1.014 β [Langfeld, Lucini, Pellegrini, Rago, arXiv:1509.08391]
LLR approach: Early objections 2. You can only calculate observables that are a function of S [ φ ] dE E n ρ ( E ) e β E R Typically: h S n i = R dE ρ ( E ) e β E Progress: arbitrary observables accessible 1 h B [ φ ] i = P i δ E ˜ ρ ( E i ) h h B [ φ ] exp { β S [ φ ] + a i ( S [ φ ] � E i ) } i i Z ( β ) Z ( β ) = P i δ E ˜ ρ ( E i ) h h exp { β S [ φ ] + a i ( S [ φ ] � E i ) } i i 2nd order accuracy: O ( δ E 2 ) [same of for the density-of-states] [Langfeld, Lucini, Pellegrini, Rago, arXiv:1509.08391]
…enough theory. We want results!
LLR approach 4d compact U(1) gauge theory Theory with a (very) weak 1st order phase transition Record: lattice, multicanonical HMC + supercomputer 18 4 [Arnold, Lippert,Neuhaus, Schilling, Nucl.Phys.Proc.Suppl. 94 (2001) 651] LLR: how does a(E) depend on the lattice -1 size? expectation: a i -1.02 L=8 L=10 a = d ln ρ L=12 dE L=14 L=16 -1.04 L=18 [independent of L L=20 for large L] 0.58 0.6 0.62 0.64 0.66 0.68 ε
LLR approach 4d compact U(1) gauge theory How does the LLR results compare with those from the literature? Define critical coupling from the peak position of the β c ( L ) specific heat (credits to RP & AR) record!
LLR approach 4d compact U(1) gauge theory The probability distribution for : ρ ( E ) e β E β = β c ( ∞ ) 120 100 80 P β (E) 60 40 20 L=20 0 0.61 0.62 0.63 0.64 0.65 0.66 0.67 E/6V
LLR approach 4d compact U(1) gauge theory Do we achieve the predicted precision ? O ( δ E 2 ) 6.0e-04 The specific heat: c V ( β c ( L )) L=8 5.0e-04 L=10 L=12 L=14 L=16 C V ( β c (L)) 4.0e-04 3.0e-04 yes! 2.0e-04 0 0.02 0.04 0.06 0.08 δ E /V
The LLR algorithm is designed to solve overlap problems, but can it solve Sign problems? Recall: theory with complex action Define the generalised density-of-states: Partition function emerges from a FT:
LLR approach for complex systems: Need to calculate the overlap density, etc R ds exp { iµs } P β ( s ) Z ( µ ) O ( µ ) = Z PQ ( µ ) = R ds P β ( s ) overall normalisation drops out Testbed: Z3 Polyakov line model (3d spin model, discrete) η = κ e µ , η = κ e − µ z ∈ Z 3 τ : temperature, ¯ Solvable: dual theory is real, efficient flux algorithm [Mercado, Evertz, Gattringer, PRL 106 (2011) 222001]
Z3 spin model Dual solutions show: Strong sign problem snake algorithm
What is the challenge Z3 spin model [without dualisation]? Indicative result: action volume statistical errors exponentially small Need exponential error LLR approach: suppression over the whole action range
LLR approach: Numerical findings Z3 spin model 1 histogram density-of-states 1e-15 P β ( s ) 1e-30 1e-45 needed for FT 1e-60 -1000 0 1000 2000 3000 4000 5000 6000 N + - N - s = [Langfeld, Lucini, PRD 90 (2014) 094502]
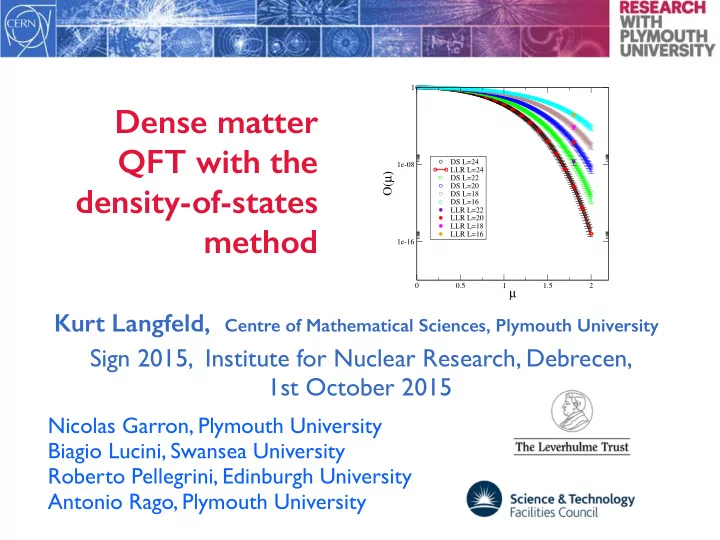
LLR approach: Numerical findings Z3 spin model We haven’t talked about FT (see later), but our findings are: 1 DS L=24 1e-08 LLR L=24 O( µ) DS L=22 DS L=20 DS L=18 First “head on” DS L=16 LLR L=22 solution of a sign LLR L=20 LLR L=18 problem! LLR L=16 1e-16 0 0.5 1 1.5 2 µ [Langfeld, Lucini, PRD 90 (2014) 094502]
Z3 spin model Silver Blaze Problem in the Z3 model: ∂ ρ ( µ ) = T Recall: ∂ µ ln O ( µ ) + ρ PQ ( µ ) V 3 always negative The phase quenched theory always overestimates the true density
….let’s discuss new physics!
Anatomy of a sign problem: Heavy-Dense QCD (HDQCD) Z Starting point Z ( µ ) = D U µ exp { β S YM [ U ] } Det M ( µ ) QCD: SU(3) gauge theory quark determinant Limit quark mass , large, µ/m → finite m µ [Bender, Hashimoto, Karsch, Linke, Nakamura, Plewnia, Nucl. Phys. Proc. Suppl. 26 (1992) 323] ⌘ 2 ⌘ 2 ⇣ ⇣ 1 + e ( µ − m ) /T P ( ~ 1 + e − ( µ + m ) /T P † ( ~ Y Det M ( µ ) = det x ) det x ) x ~ neglect anti-quark contribution Low temperatures, : µ > 0 ⌘ 2 ⇣ 1 + e ( µ − m ) /T P ( ~ Y Det M ( µ ) = det x ) ~ x
Recommend
More recommend