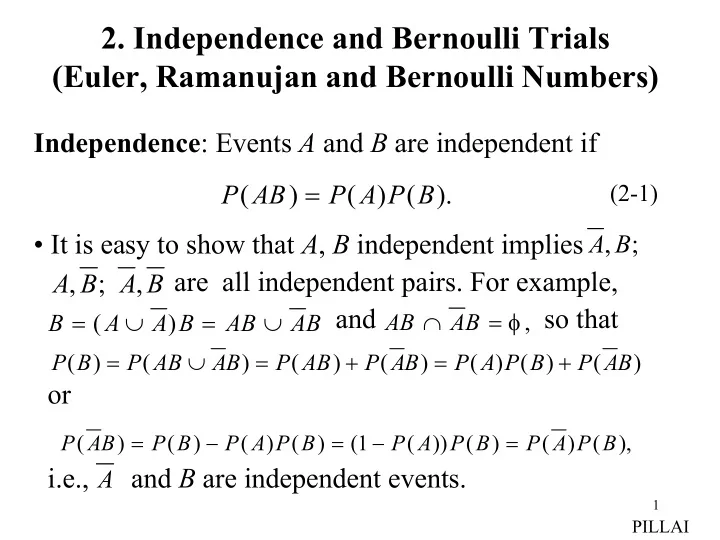

2. Independence and Bernoulli Trials (Euler, Ramanujan and Bernoulli Numbers) Independence : Events A and B are independent if = P ( AB ) P ( A ) P ( B ). (2-1) • It is easy to show that A , B independent implies A , B ; are all independent pairs. For example, A , B ; A , B and so that = ∪ = ∪ ∩ = φ AB A B , B ( A A ) B AB A B = ∪ = + = + P ( B ) P ( AB A B ) P ( AB ) P ( A B ) P ( A ) P ( B ) P ( A B ) or = − = − = P ( A B ) P ( B ) P ( A ) P ( B ) ( 1 P ( A )) P ( B ) P ( A ) P ( B ), i.e., and B are independent events. A 1 PILLAI
As an application, let A p and A q represent the events = A "the prime divides the number " p N p and = A "the prime divides the number ". q N q Then from (1-4) 1 1 = = P A { } , P A { } p q p q Also 1 ∩ = = = P A { A } P {" pq divides N "} P A { } { P A } p q p q pq (2-2) Hence it follows that A p and A q are independent events! 2 PILLAI
AB ⊂ • If P ( A ) = 0, then since the event always, we have A ≤ = ⇒ = P ( AB ) P ( A ) 0 P ( AB ) 0 , and (2-1) is always satisfied. Thus the event of zero probability is independent of every other event! • Independent events obviously cannot be mutually > > exclusive, since and A , B independent P ( A ) 0 , P ( B ) 0 > implies Thus if A and B are independent, P ( AB ) 0 . the event AB cannot be the null set. { } • More generally, a family of events are said to be A i independent, if for every finite sub collection we have A , A , � , A , i i i 1 2 n n n ∏ ∩ = P A P ( A ). (2-3) i i k k = = k 1 3 k 1 PILLAI
• Let = ∪ ∪ ∪ ∪ (2-4) A A A A � A , 1 2 3 n a union of n independent events. Then by De-Morgan’s law = A A A � A 1 2 n and using their independence n n ∏ ∏ = = = − P ( A ) P ( A A � A ) P ( A ) ( 1 P ( A )) . (2-5) 1 2 n i i = = i 1 i 1 Thus for any A as in (2-4) n ∏ = − = − − P ( A ) 1 P ( A ) 1 ( 1 P ( A )) , (2-6) i = i 1 a useful result. We can use these results to solve an interesting number theory problem. 4 PILLAI
Example 2.1 Two integers M and N are chosen at random. What is the probability that they are relatively prime to each other? Solution: Since M and N are chosen at random, whether p divides M or not does not depend on the other number N . Thus we have P {" divides both p M and "} N 1 = = P {" divides p M "} {" divides P p N "} 2 p where we have used (1-4). Also from (1-10) P {" does p not divede both M and N "} 1 = − = − 1 P {" divides both p M and N "} 1 2 p Observe that “ M and N are relatively prime” if and only if 5 there exists no prime p that divides both M and N. PILLAI
Hence = ∩ ∩ ∩ � " M and N are relatively prime" X X X 2 3 5 where X p represents the event = X " divides both p M and N ". p Hence using (2-2) and (2-5) ∏ = P {" M and N are relatively prime"} P X ( ) p p prime 1 1 6 ∏ = − = = = = 1 (1 ) 0.6079, ∞ 2 p π π 2 2 /6 ∑ 2 p prime 1/ k = k 1 where we have used the Euler’s identity 1 6 1 See Appendix for a proof of Euler’s identity by Ramanujan. PILLAI
∞ ∑ ∏ − 1 = − s 1 1/ k (1 ) . p s = k 1 p prime The same argument can be used to compute the probability that an integer chosen at random is “square free”. Since the event "An integer chosen at random is square free" = ∩ 2 {" p does not divide N "}, p prime using (2-5) we have P {"An integer chosen at random is square free"} ∏ ∏ = = − 2 1 P p { does not divide } N (1 ) 2 p p prime p prime 1 1 6 . = = = ∞ π π 2 2 /6 ∑ 2 1/ k 7 = k 1 PILLAI
Note : To add an interesting twist to the ‘square free’ number problem, Ramanujan has shown through elementary but clever arguments that the inverses of the n th powers of all ‘square free’ numbers add to where (see (2-E)) S / S , n 2 n ∞ = ∑ n S 1/ k . n = k 1 Thus the sum of the inverses of the squares of ‘square free’ numbers is given by 1 1 1 1 1 1 1 1 1 S + + + + + + + + + = � 2 2 2 2 2 2 2 2 2 2 2 3 5 6 7 10 11 13 14 S 4 π 2 /6 15 = = = 1.51198. π π 4 2 /90 8 PILLAI
Example 2.2: Three switches connected in parallel operate independently. Each switch remains closed with probability p . (a) Find the probability of receiving an input signal at the output. (b) Find the probability that switch S 1 is open given that an input signal is received at the output. s 1 s 2 Input Output s 3 Fig.2.1 i = Solution: a. Let A i = “Switch S i is closed”. Then P ( A ) p , = → Since switches operate independently, we have i 1 3 . = = P ( A A ) P ( A ) P ( A ); P ( A A A ) P ( A ) P ( A ) P ( A ). i j i j 1 2 3 1 2 3 9 PILLAI
Let R = “input signal is received at the output”. For the event R to occur either switch 1 or switch 2 or switch 3 must remain closed, i.e., (2-7) = ∪ ∪ R A A A . 1 2 3 Using (2-3) - (2-6), = ∪ ∪ = − − = − + 3 2 3 P ( R ) P ( A A A ) 1 ( 1 p ) 3 p 3 p p . (2-8) 1 2 3 We can also derive (2-8) in a different manner. Since any event and its compliment form a trivial partition, we can always write = + (2-9) P ( R ) P ( R | A ) P ( A ) P ( R | A ) P ( A ). 1 1 1 1 1 = But and = ∪ = − 2 P ( R | A ) 1 , P ( R | A ) P ( A A ) 2 p p 1 2 3 and using these in (2-9) we obtain = + − − = − + 2 2 3 P ( R ) p ( 2 p p )( 1 p ) 3 p 3 p p , (2-10) which agrees with (2-8). 10 PILLAI
Note that the events A 1 , A 2 , A 3 do not form a partition, since they are not mutually exclusive. Obviously any two or all three switches can be closed (or open) simultaneously. Moreover, + + ≠ P ( A ) P ( A ) P ( A ) 1 . 1 2 3 b. We need From Bayes’ theorem P ( A 1 R | ). − − − + 2 2 P ( R | A ) P ( A ) ( 2 p p )( 1 p ) 2 2 p p (2-11) 1 1 = = = P ( A | R ) . 1 − + − + 2 3 2 3 P ( R ) 3 p 3 p p 3 p 3 p p Because of the symmetry of the switches, we also have = = P ( A | R ) P ( A | R ) P ( A | R ). 1 2 3 11 PILLAI
Repeated Trials Consider two independent experiments with associated probability models ( Ω 1 , F 1 , P 1 ) and ( Ω 2 , F 2 , P 2 ). Let ξ ∈Ω 1 , η ∈Ω 2 represent elementary events. A joint performance of the two experiments produces an elementary events ω = ( ξ , η ). How to characterize an appropriate probability to this “combined event” ? Towards this, consider the Cartesian product space Ω = Ω 1 × Ω 2 generated from Ω 1 and Ω 2 such that if ξ ∈ Ω 1 and η ∈ Ω 2 , then every ω in Ω is an ordered pair of the form ω = ( ξ , η ). To arrive at a probability model we need to define the combined trio ( Ω , F , P ). 12 PILLAI
Suppose A ∈ F 1 and B ∈ F 2 . Then A × B is the set of all pairs ( ξ , η ), where ξ ∈ A and η ∈ B. Any such subset of Ω appears to be a legitimate event for the combined experiment. Let F denote the field composed of all such subsets A × B together with their unions and compliments. In this combined experiment, the probabilities of the events A × Ω 2 and Ω 1 × B are such that × Ω = Ω × = P ( A ) P ( A ), P ( B ) P ( B ). (2-12) 2 1 1 2 Moreover, the events A × Ω 2 and Ω 1 × B are independent for any A ∈ F 1 and B ∈ F 2 . Since (2-13) × Ω ∩ Ω × = × ( A ) ( B ) A B , 2 1 we conclude using (2-12) that 13 PILLAI
(2-14) × = × Ω ⋅ Ω × = P ( A B ) P ( A ) P ( B ) P ( A ) P ( B ) 2 1 1 2 for all A ∈ F 1 and B ∈ F 2 . The assignment in (2-14) extends to a unique probability measure on the sets in F ≡ × P ( P P ) 1 2 and defines the combined trio ( Ω , F , P ). Generalization : Given n experiments and Ω Ω Ω � , , , , 1 2 n their associated let = → F and P , i 1 n , i i Ω = Ω × Ω × × Ω (2-15) � 1 2 n represent their Cartesian product whose elementary events ξ ∈ Ω are the ordered n -tuples where Events ξ ξ ξ . , , � , , i i 1 2 n in this combined space are of the form × × × � (2-16) A A A 1 2 n where and their unions an intersections. A ∈ F , 14 i i PILLAI
Recommend
More recommend