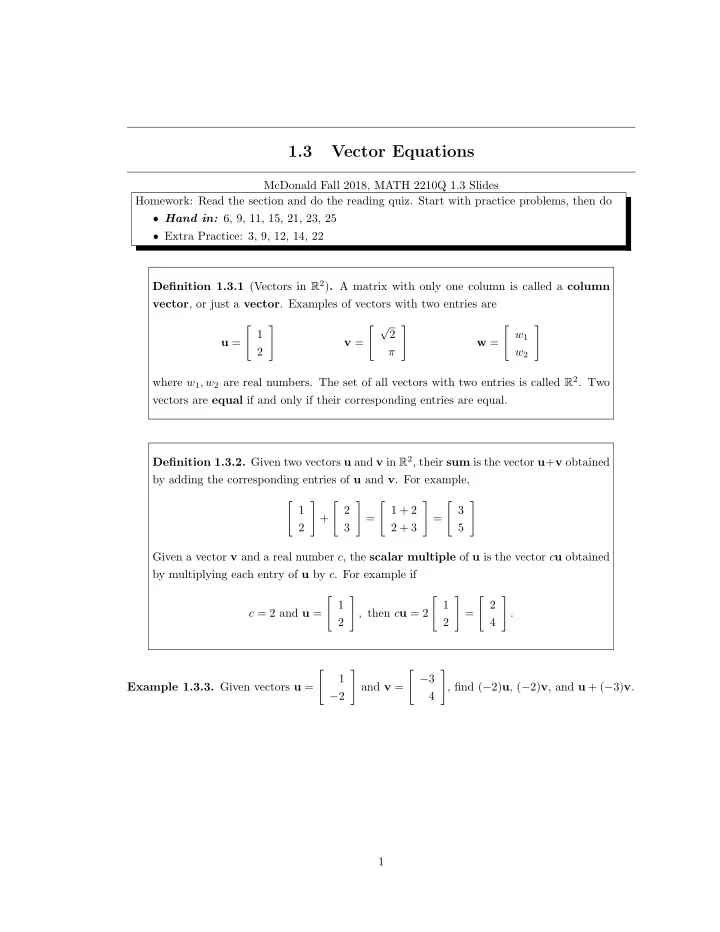

1.3 Vector Equations McDonald Fall 2018, MATH 2210Q 1.3 Slides Homework: Read the section and do the reading quiz. Start with practice problems, then do ❼ Hand in: 6, 9, 11, 15, 21, 23, 25 ❼ Extra Practice: 3, 9, 12, 14, 22 Definition 1.3.1 (Vectors in R 2 ) . A matrix with only one column is called a column vector , or just a vector . Examples of vectors with two entries are � √ � � � � � 1 2 w 1 u = v = w = 2 π w 2 where w 1 , w 2 are real numbers. The set of all vectors with two entries is called R 2 . Two vectors are equal if and only if their corresponding entries are equal. Definition 1.3.2. Given two vectors u and v in R 2 , their sum is the vector u + v obtained by adding the corresponding entries of u and v . For example, � � � � � � � � 1 2 1 + 2 3 + = = 2 3 2 + 3 5 Given a vector v and a real number c , the scalar multiple of u is the vector c u obtained by multiplying each entry of u by c . For example if � � � � � � 1 1 2 c = 2 and u = , then c u = 2 = . 2 2 4 � � � � 1 − 3 Example 1.3.3. Given vectors u = and v = , find ( − 2) u , ( − 2) v , and u + ( − 3) v . − 2 4 1
� a Observation 1.3.4 (Vectors in R 2 ) . We can identify the column vector � with the b point ( a, b ) in the plain, so we can consider R 2 as the set of all points in the plain. We usually visualize a vector by including an arrow from the origin. � � � � 2 − 6 Example 1.3.5. Let u = and v = . Graph u , v and u + v on the plane. 2 1 Proposition 1.3.6 (Parallelogram Rule) . If u and v in R 2 are represented in the plain, then u + v corresponds to the last vertex of the parallelogram with vertices are u , v and 0 . � � 1 Example 1.3.7. Let u = . Graph u , ( − 2) u , and 3 u . What’s special about c u for any c ? − 1 Observation 1.3.8 (Vectors in R 3 ) . Vectors in R 3 are 3 × 1 matrices. Like above, we can represent them geometrically in three-dimensional coordinate space. For example, 2 a = 3 4 2
Definition 1.3.9 (Vectors in R n ) . If n is a positive integer, R n denotes the collection of ordered n -tuples of n real numbers, usually written as n × 1 column matrices, such as a 1 a 2 a = , . . . a n we we again, sometimes denote ( a 1 , a 2 , . . . , a n ). The zero vector , denoted 0 is the vector whose entries are all zero. We also denote ( − 1) u = − u . Proposition 1.3.10 (Algebraic Properties of R n ) . For u , v , w in R n , and scalars c , d : (i) u + v = v + u (v) c ( u + v ) = c u + c v (ii) ( u + v ) + w = u + ( v + w ) (vi) ( c + d ) u = c u + d u (iii) u + 0 = 0 + u = u (vii) c ( d u ) = ( cd ) u (iv) u + ( − u ) = − u + u = 0 (viii) 1 u = u a 1 a 2 Remark 1.3.11. Sometimes, for ease of notation, we denote as ( a 1 , a 2 , . . . , a n ). . . . a n Example 1.3.12. Prove properties (i) and (v) of the Algebraic Properties above. 3
Definition 1.3.13 (Linear Combinations) . Given vectors v 1 , v 2 , . . . , v m in R n , and scalars c 1 , c 2 , . . . , c m . The vector c 1 v 1 + c 2 v 2 + · · · + c m v m is called a linear combination of the v 1 , . . . v m with weights c 1 , . . . , c m . � 2 � − 1 � � Example 1.3.14. The figure below shows linear combinations of v 1 = and v 2 = where 1 1 with integer weights. Estimate the linear combinations of v 1 and v 2 that produce u and w . 1 2 7 Example 1.3.15. Let a 1 = − 2 , a 2 = 5 , b = 4 . Is b a linear combination of a 1 and a 2 ? − 5 6 − 3 4
Remark 1.3.16. In the previous example, the vectors a 1 , a 2 and b became the columns of the augmented matrix that we reduced: 1 2 7 − 2 5 4 − 5 6 − 3 � � For brevity, we will write this matrix, using vectors, as . This suggests the following. a 1 a 2 b Procedure 1.3.17. A vector equation x 1 a 1 + · · · + x n a n = b , has the same solution set as the linear system whose augmented matrix is � � a 1 · · · a n b In particular, b can be represented as a linear combination of a 1 , . . . , a n if and only if there is a solution to the linear system corresponding to this matrix. Definition 1.3.18. If v 1 , . . . , v m are in R n , then the set of all linear combinations of is denoted by Span { v 1 , . . . , v m } and is called the subset of R n spanned by v 1 , . . . , v m . In other words, Span { v 1 , . . . , v m } is the collection of all vectors of the form c 1 v 1 + c 2 v 2 + · · · + c m v m , with c 1 , . . . , c m scalars. � � � � − 1 2 Example 1.3.19. Let v 1 = and v 2 = . Prove that v 1 and v 2 span all of R 2 . 1 1 5
Remark 1.3.20. Actually, for any u and v (which are not multiples) in R 3 , Span { u , v } is a plane! Observation 1.3.21 (Geometric Descriptions of Span { u } and Span { u , v } ) . Let u and v be nonzero vectors in R 3 , with u not a multiple of v . Then Span { v } is the set of points on the line in R 3 through 0 and v , and Span { u , v } is the plane in R 3 containing 0 , u and v , that is, it contains the line in R 3 through u and the line through 0 and v and 0 . 1 5 Example 1.3.22. If a 1 = , a 2 = . Is ( − 3 , 8 , 1) in the plane spanned by a 1 and a 2 ? − 2 − 13 3 − 3 6
Recommend
More recommend