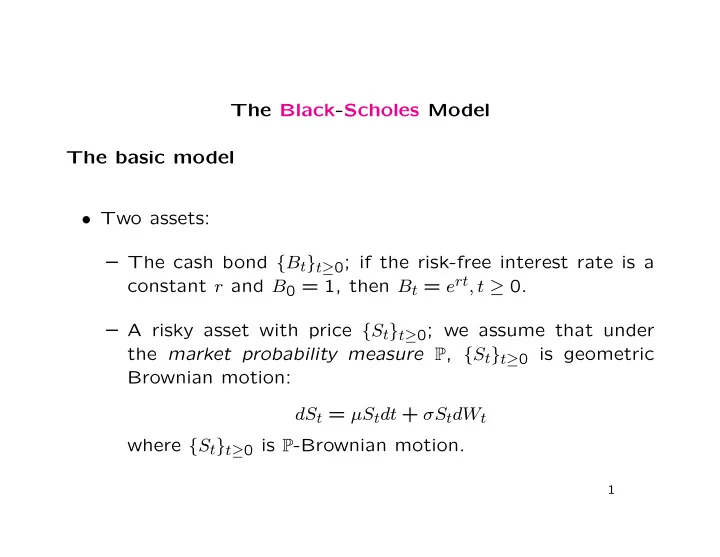
The Black-Scholes Model The basic model Two assets: The cash bond - PowerPoint PPT Presentation

The Black-Scholes Model The basic model • Two assets: – The cash bond { B t } t ≥ 0 ; if the risk-free interest rate is a constant r and B 0 = 1, then B t = e rt , t ≥ 0. – A risky asset with price { S t } t ≥ 0 ; we assume that under the market probability measure P , { S t } t ≥ 0 is geometric Brownian motion: dS t = µS t dt + σS t dW t where { S t } t ≥ 0 is P -Brownian motion. 1

Self-financing strategy • We want the no-arbitrage price at time t of a claim C T at time T that depends on the path up to time T . • If we can replicate the claim with a self-financing portfolio of cash and stock, the no-arbitrage price must be the value of that portfolio. • Consider the portfolio ( ψ, φ ) consisting at time t of ψ t bonds and φ t shares; { ψ t } 0 ≤ t ≤ T and { φ t } 0 ≤ t ≤ T must be predictable . 2

• To be self-financing, the portfolio value V t ( ψ, φ ) = ψ t B t + φ t S t satisfies dV t ( ψ, φ ) = ψ t dB t + φ t dS t . • In integrated form, V t ( ψ, φ ) − V 0 ( ψ, φ ) = ψ t B t + φ t S t − ψ 0 B 0 − φ 0 S 0 � t � t = 0 ψ u dB u + 0 φ u dS u . • That is, � t � t ψ t B t + φ t S t = ψ 0 B 0 + φ 0 S 0 + 0 ψ u dB u + 0 φ u dS u . 3

• We assume that � T � T 0 | φ t | 2 dt < ∞ : 0 | ψ t | dt + – ensures that the integrals are well defined; – also excludes strategies, like “doubling”, that require un- bounded borrowing. • If { ˜ V t ( ψ, φ ) } t ≥ 0 and { ˜ S t } t ≥ 0 are the corresponding discounted values, then d ˜ V t ( ψ, φ ) = φ t d ˜ S t , or � t V t ( ψ, φ ) = ˜ ˜ 0 φ u d ˜ V 0 ( ψ, φ ) + S u . 4

Approach to pricing the claim • Suppose that we can find a predictable process { φ t } 0 ≤ t ≤ T and a constant φ ∗ such that the discounted claim △ = B − 1 ˜ C T T C T satisfies � T C T = φ ∗ + ˜ 0 φ u d ˜ S u . • Note that we do not assume that φ ∗ = φ 0 . 5

• Let � t ψ t = φ ∗ + 0 φ u d ˜ S u − φ t ˜ S t . • If { ˜ V t ( ψ, φ ) } 0 ≤ t ≤ T is the value of the portfolio ( ψ, φ ), then � t S t = φ ∗ + V t ( ψ, φ ) = ψ t + φ t ˜ ˜ 0 φ u d ˜ S u . • So d ˜ V t ( ψ, φ ) = φ t d ˜ S t , and the portfolio is self-financing. 6

• Also � T V T ( ψ, φ ) = φ ∗ + ˜ 0 φ u d ˜ S u = ˜ C T , so the portfolio replicates the claim. • So the no-arbitrage price of the claim is V 0 ( ψ, φ ) = φ ∗ . ˜ • Problem: how to find { φ t } 0 ≤ t ≤ T and φ ∗ , or at least φ ∗ . 7

find a measure Q such that { ˜ • Solution: S t } 0 ≤ t ≤ T is a Q - martingale. • Then so is { ˜ V t ( ψ, φ ) } 0 ≤ t ≤ T . • So φ ∗ = ˜ V 0 ( ψ, φ ) = E Q � � ˜ V T ( ψ, φ ) = E Q � � ˜ C T . 8

The equivalent martingale measure • Recall that dS t = µS t dt + σS t dW t so d ˜ S t = ( µ − r )˜ S t dt + σ ˜ S t dW t . • Write θ = ( µ − r ) /σ and X t = W t + θt . 9

• Girsanov’s Theorem states that if Q is defined by − θW t − 1 d Q � � � 2 θ 2 t � = exp � d P � F t then { X t } t ≥ 0 is a Q -Brownian motion. • Also d ˜ S t = ˜ S t σdX t , so { ˜ S t } t ≥ 0 is a Q -martingale, and σX t − 1 � � 2 σ 2 t S t = ˜ ˜ S 0 exp . 10

The replicating portfolio • We still need to find the predictable processes { ψ t } 0 ≤ t ≤ T and { φ t } 0 ≤ t ≤ T defining the replicating portfolio ( ψ, φ ). • Let △ � = E Q � � ˜ M t C T � F t . � • Then { M t } 0 ≤ t ≤ T is a Q -martingale, and by the martingale representation theorem there exists a predictable process { θ t } 0 ≤ t ≤ T such that � t M t = M 0 + 0 θ s dX s . 11

• So if φ t = θ t / ( σ ˜ S t ), then � t 0 φ u σ ˜ M t = M 0 + S u dX u � t 0 φ u d ˜ = M 0 + S u . • So � T ˜ 0 φ u d ˜ C T = M T = M 0 + S u , as required. • We therefore know that { φ t } 0 ≤ t ≤ T exists , but we have not constructed it. When C T = f ( S T ), we can use the Feynman- Kac representation to construct { φ t } 0 ≤ t ≤ T . 12