Standard form of the maximum principle The controlled system of - PDF document

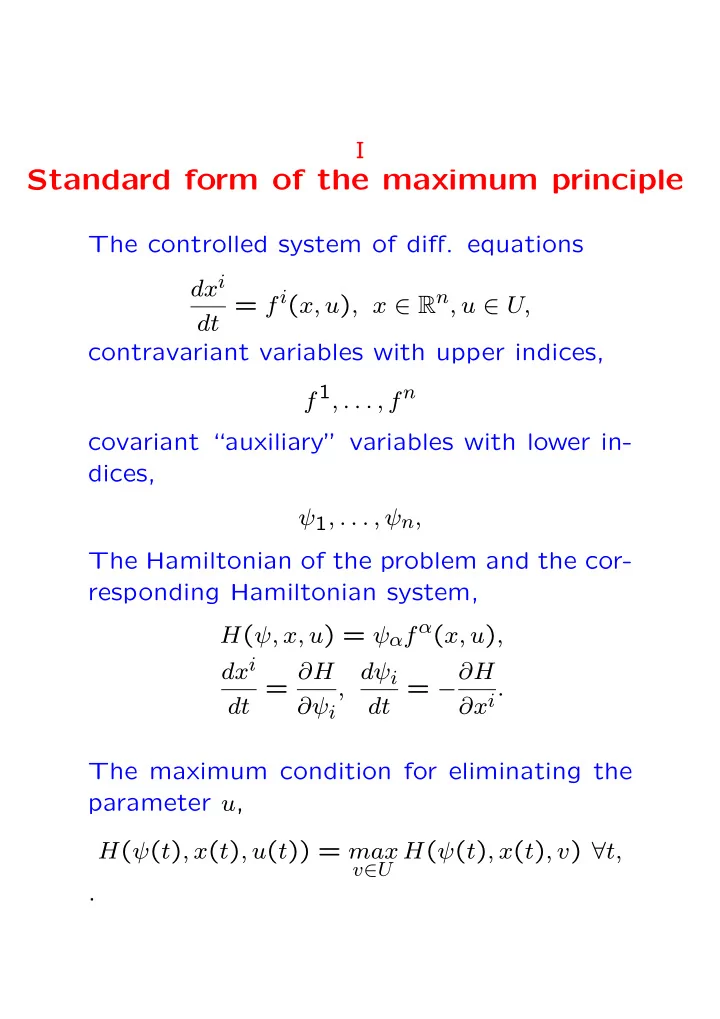
I Standard form of the maximum principle The controlled system of diff. equations dx i dt = f i ( x, u ) , x ∈ R n , u ∈ U, contravariant variables with upper indices, f 1 , . . . , f n covariant “auxiliary” variables with lower in- dices, ψ 1 , . . . , ψ n , The Hamiltonian of the problem and the cor- responding Hamiltonian system, H ( ψ, x, u ) = ψ α f α ( x, u ) , dx i dt = ∂H , dψ i dt = − ∂H ∂x i . ∂ψ i The maximum condition for eliminating the parameter u , H ( ψ ( t ) , x ( t ) , u ( t )) = max v ∈ U H ( ψ ( t ) , x ( t ) , v ) ∀ t, .

II The Pontryagin derivative P X Extremals of the problem � t 1 I = L ( q, ˙ q ) dt = min, t 0 q = ( q 1 , . . . , q n ) , ˙ q 1 , . . . , ˙ q n ) , q = ( ˙ are solutions of the Euler-Lagrange equation ∂ 2 L q + ∂ 2 L q − ∂ L q 2 ¨ q ˙ ∂q = 0 . ∂ ˙ ∂q ∂ ˙ The Euler-Lagrange derivative: q �→ ∂ 2 L q + ∂ 2 L q − ∂ L q 2 ¨ q ˙ ∂q . ∂ ˙ ∂q ∂ ˙ Invariant formulation of the time-optimal pr- oblem: X = X ( x, u ) ∈ T x M ⊂ TM, x ∈ M, u ∈ U. The fiberwise linear Hamiltonian H X ( ψ, u ) of the problem and the Pontryagin derivative P X : def = < ψ, X ( πψ, u ) >, ψ ∈ T ∗ H X ( ψ, u ) πψ M, i P X ω = dH X

Computing H X and P X in canonical coordi- nates q = ( q 1 , . . . , q n ) , p = ( p 1 , . . . , p n ):  ω = dq α ∧ dp α , X = X α ∂ ∂x α , ψ = p α dx α   H X = < ψ, X > = p α X α = pX.    P X = Q ∂ ∂q + P ∂ ∂p = Q α ∂ ∂q α + P ∂    ∂p α      � �  < dq, P X >< dp, P X >  � �  i P X dq ∧ dp = det � =  � �  dq dp  � �   �   Q dp − P dq = dH X = p∂X ∂q dq + X dp = ⇒       Q = X, P = − p∂X   ∂q dq,         ⇓   P X = X ∂ ∂q − p∂X ∂ ∂q ∂p ⇓ dq dt = X = ∂H X ∂p , dp dt = − p∂X ∂q = − ∂H X ∂q

III Identification of P X Pontryagin derivative P X is the unique ex- tension as a vector field on T ∗ M of the Lie bracket operator ad X Y = [ X, Y ] , initially de- fined as a derivation on the C ∞ ( M ) -module V ect M . The C ∞ ( T ∗ M )-module M = M 0 ⊕ M 1 ⊂ C ∞ ( T ∗ M ) , M 0 = π ∗ C ∞ ( M ) , M 1 = V ect M and unique extension to V ect T ∗ M of deriva- tions on M , which preserve both submodules M 0 , M 1 . The importance of M in the geometry of T ∗ M : ∀ A ∈ C ∞ ( T ∗ M ) , A = F ( q, p ) � � ∀ X , Y ∈ V ect T ∗ M. � � = Y = ⇒ X = Y X � � � M � M

P X = X ∂ ∂q − p∂X ∂ ∂q ∂p ⇓ I ) P X · π ∗ a = π ∗ · Xa, II ) P X H Y = H ad X Y , ⇓ P X is a Hamiltonian lift over X, i.e. � ∀ ψ ∈ T ∗ M ; � = X πψ π ∗ · P X � � ψ II ) P X H Y = H ad X Y ⇓ If the flow G t is generated by P X t , X t = X ( x, u ( t )) , then G t is bundle preserving over generated by X t : the flow g t � G t : T ∗ → T ∗ � x M − g t x M ∀ x ∈ M. � � T ∗ x M

IV P X (= ad X ) through the Lie Expression of X , derivative L X over e t L X = e tX : TM − → TM. ∗ The conjugate to the bundle-preserving flow e t L X over the flow e tX : ∗   � ∗ � � def � e t L X  e t L X � � = : � �  � T ∗ x M � T e − tXx M T ∗ − T ∗ e − tX x M ← x M ∀ x ∈ M The natural duality � ∗− 1 = � ∗ � � e t ad X = e t L X e − t L X e t L X , e t ad X are consid- The same duality, if ered as corresponding pullback flows of auto- morphisms and restricted to Λ 1 ( M ) , V ect M : e tX < θ, Y > = < e t L X θ, e t ad X Y > ⇓ X < θ, Y > = < L X θ, Y > + < θ, ad X Y >

Pontryagin derivative P X is the unique ex- tension as a vector field on T ∗ M of the Lie bracket operator ad X Y = [ X, Y ] , initially de- fined as a derivation on the C ∞ ( M ) -module V ect M . P X preserves the submodules M 0 , M 1 of fiber- wise constant and fiberwise linear functions on T ∗ M , hence every flow on T ∗ M generated by a nonstationary vector field, P X t , X t = X ( x, u ( t )) , is bundle-preserving. There exists a natural duality between the Lie and Pontryagin derivatives expressed by the relation � ∗− 1 � � ∗ � � � e t P X = e t L X e − t L X = or, in the infinitesimal form , X < θ, Y > = < L X , Y > + < θ, P X > . Note. The operator X �→ P X is not a con- nection since applied to aX, a ∈ C ∞ ( M ) , it differentiates a .