Pythagoras Theorem in Noncommutative Geometry Francesco DAndrea A - PowerPoint PPT Presentation

Pythagoras Theorem in Noncommutative Geometry Francesco D’Andrea A c b B a C Workshop on Noncommutative Geometry and Optimal Transport Besanc ¸on, 27/11/2014

Introduction ◮ The line element in nc geometry “is” the inverse of the Dirac operator: M 2 “ ds ∼ D − 1 ” ds M 1 ds 2 ◮ For a product of Riemannian manifolds M = M 1 × M 2 , with product metric: ds 1 ds 2 = ds 2 1 + ds 2 2 is an “infinitesimal” version of Pythagoras equality. D 2 = D 2 ◮ For a product of noncommutative manifolds (spectral triples): 1 ⊗ 1 + 1 ⊗ D 2 2 which is a sort of “inverse Pythagoras equality”: 1 1 1 ds 2 = + ( ⋆ ) ds 2 ds 2 1 2 See e.g.: A. Connes, Variations sur le th` eme spectral (2007), available on-line. ◮ Can we “integrate” ( ⋆ ) to get some (in)equalities for the distance in ncg? 1 / 19

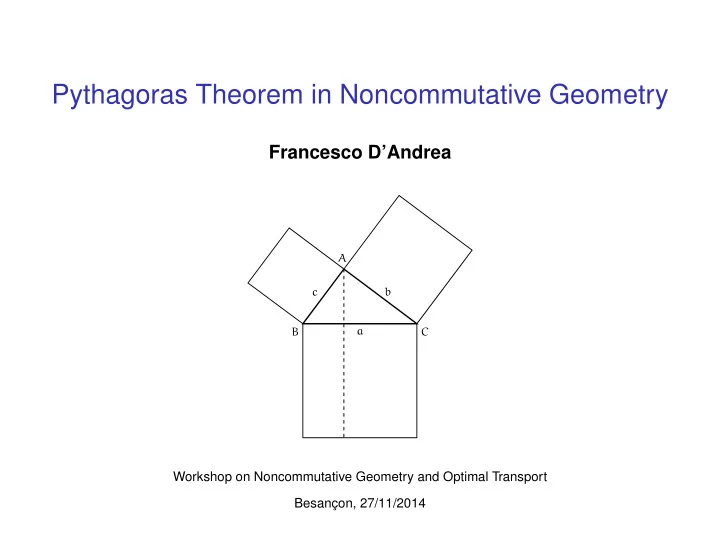
Pythagoras A a 2 = b 2 + c 2 c b or d ( B , C ) 2 = d ( A , B ) 2 + d ( A , C ) 2 ( † ) B a C Generalization to nc geometry: • what are points A , B , C , . . . ? • what is a right-angle triangle? • what is d ( A , B ) ? • is ( † ) still valid? Reference: FD & P . Martinetti, On Pythagoras Theorem for Products of Spectral Triples , Lett. Math. Phys. 103 (2013), 469–492. 2 / 19

What are points? • Any topological space X is the spectrum of a commutative C ∗ -algebra A = C 0 ( X ) . • Points are linear maps δ x : C 0 ( X ) → C , δ x ( f ) := f ( x ) . Recall that: A state on a C ∗ -algebra A is a linear map ϕ : A → C which is positive, Definition. i.e. ϕ ( a ∗ a ) � 0 ∀ a ∈ A , and normalized: � ϕ � := sup a � = 0 | ϕ ( a ) | / � a � = 1 . � � • The set S ( A ) := states of A is a convex space. Extreme points are called pure states. � � � � � � • A = C 0 ( X ) ⇒ S ( A ) = δ x : x ∈ X probability measures on X ∧ pure states = . • Quantum mechanical interpretation: � a ∈ A : a = a ∗ � A s.a. := ← → physical observables outcome of a measure ← → eigenvalue of a (or residual spectrum) ϕ ∈ S ( A ) associates to any observable a its expectation value 3 / 19

What is a right-angle triangle? Sp ( A 2 ) Pythagoras revised (here C = ( 0, 0 ) ): d ( δ b ⊗ δ c , δ 0 ⊗ δ 0 ) 2 = ( 0, c ) B ( b , c ) = d ( δ b , δ 0 ) 2 + d ( δ c , δ 0 ) 2 a c C b A ( b , 0 ) Sp ( A 1 ) Dictionary � Point A ( b , 0 ) pure state δ b of A 1 = C 0 ( R ) � Point ( 0, c ) pure state δ c of a second copy A 2 = C 0 ( R ) � Point B ( b , c ) product δ b ⊗ δ c : state of A 1 ⊗ A 2 ≃ C 0 ( R × R ) Given two separable states ϕ = ϕ 1 ⊗ ϕ 2 and ψ = ψ 1 ⊗ ψ 2 on A = A 1 ⊗ A 2 , is d ( ϕ , ψ ) 2 = d ( ϕ 1 , ψ 1 ) 2 + d ( ϕ 2 , ψ 2 ) 2 ? 4 / 19

Spectral triples Example: the Hodge-Dirac operator M = oriented Riemannian manifold. Definition ◮ A = C ∞ A spectral triple is given by: 0 ( M ) ◮ H = Ω • ( M ) = L 2 -diff. forms ◮ a complex separable Hilbert space H ; ◮ D = d + d ∗ γ = (− 1 ) degree ◮ a ∗ -algebra A of bounded operators on H ; It is unital ⇐ ⇒ M is compact. ◮ a (unbounded) selfadjoint operator D on H s.t. [ D , a ] is bounded and a ( D + i ) − 1 is a Example: the generator of K 0 ( C ) compact operator for all a ∈ A . � � a � � 0 ◮ A = : a ∈ C It is called: 0 0 ◮ unital if 1 B ( H ) ∈ A ; ◮ H = C 2 � 0 � � 1 � ◮ even if ∃ a grading γ on H s.t. A is even and 1 0 ◮ D = γ = D is odd; 1 0 0 − 1 Note that this is a non-unital spectral ◮ non-degenerate if triple (because 1 M 2 ( C ) / ∈ A ), even if � � Span av : a ∈ A , v ∈ H ≡ H . A ≃ C is a unital algebra. 5 / 19

What is d ( ϕ , ψ ) ? A spectral triple induces a distance on S ( A ) : � � d A , D ( ϕ , ψ ) := sup a ∈ A s.a. ϕ ( a ) − ψ ( a ) : � [ D , a ] � � 1 ∀ ϕ , ψ ∈ S ( A ) . , Monge d´ eblais et remblais problem: � Villani, Optimal transport, old and new c dµ 1 = distribution of material in a mine ( d´ eblais ) Same total mass: � � dµ 2 = distribution in the construction site ( remblais ) dµ 1 = dµ 2 = 1 (in suitable units) Moving a unit material from x to y = T ( x ) (transport plan) costs d geo ( x , y ) . The total cost is � � c T ( µ 1 , µ 2 ) := d geo ( x , T ( x )) dµ 1 ( x ) . For ϕ i ( f ) = f ( x ) dµ i ( x ) , the Wasserstein dist. is: W ( ϕ 1 , ϕ 2 ) := inf c T ( µ 1 , µ 2 ) ( † ) T : T ∗ ( µ 1 )= µ 2 ◮ On a complete Riem. manifold, ( † ) is the spectral distance of the Hodge-Dirac operator! 6 / 19

Example: the SNCF spectral triple Let X ⊂ R n be a finite ∗ subset and x 0 a basepoint. Take x 0 = 0 to simplify the notations. � � f : X → C s.t. f ( 0 ) = 0 Let A := , e x be the indicator function of x ∈ X and { e ± } the canonical basis of C 2 , H := A ⊗ C 2 with orthonormal basis { e x ⊗ e ± } x ∈ X . The representation π of A on H and D are � f � � � x � − 1 e x ⊕ e ∓ if x � = 0 0 π ( f ) = D ( e x ⊕ e ± ) = 0 0 0 if x = 0 Then | f ( x ) | � [ D , π ( f )] � = sup � x � � 1 | f ( x ) − f ( y ) | � � x � + � y � ∀ x , y ∈ X (including 0 ) ⇒ x � = 0 The sup is attained on the function f x , y = � x � e x − � y � e y . Thus: � � x � + � y � if x � = y d A , D ( δ x , δ y ) ≡ d SNCF ( x , y ) = 0 if x = y ∗ For the compact resolvent condition, which could be dropped if only interested in the metric aspect. 7 / 19

Optimal public-transport d geo ( Lyon , Besanc ¸on ) = 188 km d SNCF ( Lyon , Besanc ¸on ) = 720 km 8 / 19

Optimal control Other “non-Riemannian” examples arise in minimization problems with constraints. Soft Moon landing. Falling cat. thrust = - k m ' H t L total mass = m H t L m g height = h H t L c c � 2012 Wolfram Media, Inc. � R. Montgomery, Commun. Math. Phys. 1990 Goal: minimize the amount of fuel. Goal: turn of 180 degrees while falling. Constraint: at height h = 0 the velocity must Constraint: while falling, keep the total angular momentum � J = 0. ∗ be � v = 0 (avoid crashing). ∗ Motion constrained on a distribution H ⊂ TM , with M the configuration space. R. Montgomery (1993): Gauge theory of the falling cat . 9 / 19

The Carnot-Carath´ eodory distance A sub-Riemannian geometry on a manifold M consists of a vector sub-bundle H ⊂ TM with a fiber inner-product g ( · , · ) on it. A curve γ : [ 0, 1 ] → M is horizontal if ˙ γ ( t ) ∈ H ∀ t . The Carnot-Carath´ eodory distance is defined as: � 1 2 dt , d CC ( x , y ) := inf g ( ˙ γ ( t ) , ˙ γ ( t )) where the inf is on all horizontal curves from x to y . A contact manifold ( M , H ) is a 2 n + 1 -dimensional manifold M equipped with a codimension 1 vector sub-bundle H ⊂ TM which is completely non-integrable (that is, sections of H generate Vect ( M ) as a Lie algebra). Locally, H = ker τ where τ ∈ Ω 1 M satisfies τ ( dτ ) n � = 0 (contact 1 -form). Fact: there is a natural sub-Riemannian metric on any contact manifold. 10 / 19

The Rumin complex Let τ be a contact for on a 2 n + 1 -dim. manifold M , and: I • = � τ , dτ � — differential ideal generated by τ . • J • = ker ( τ ∧ ) ∩ ker ( dτ ∧ ) — annihilator. • Theorem (Rumin, 1994) ∃ a 2nd order diff. op. D such that the following is a cochain complex: d d d D d d d → Ω n / I n → J n → J n + 1 → J 2 n + 1 Ω 0 / I 0 → Ω 1 / I 1 − − → . . . − − − − − − − → . . . − Its cohomology is the de Rham cohomology of M . Observations. For f ∈ C ∞ ( M ) : • D is not 1st order, so [ D , f ] is not bounded = ⇒ no spectral triple; 1 • [ D , f ] is bounded relatively to | D | 2 = ⇒ bounded Fredholm module; • [[ D , f ] , f ] is bounded, and [R. Yuncken, Bonn 2014] � � d CC ( x , y ) = | f ( x ) − f ( y ) | : � [[ D , f ] , f ] � � 2 sup . f ∈ C ∞ ( M ) 11 / 19

Back to Pythagoras. . . Given two separable states ϕ = ϕ 1 ⊗ ϕ 2 and ψ = ψ 1 ⊗ ψ 2 on A = A 1 ⊗ A 2 , is d ( ϕ , ψ ) 2 = d ( ϕ 1 , ψ 1 ) 2 + d ( ϕ 2 , ψ 2 ) 2 satisfied by the spectral distance? S ( A 2 ) ϕ ϕ 2 d ( ϕ 2 , ψ 2 ) d ( ϕ , ψ ) ψ ψ 2 d ( ϕ 1 , ψ 1 ) ϕ 1 ψ 1 S ( A 1 ) A last ingredient is missing: on A = A 1 ⊗ A 2 we want a product metric. 12 / 19

Products of spectral triples In nc geom., the Cartesian product of spaces is replaced by the product of spectral triples. Given two spectral triples ( A 1 , H 1 , D 1 , γ 1 ) and ( A 2 , H 2 , D 2 ) , their product ( A , H , D ) is A = A 1 ⊗ A 2 , H = H 1 ⊗ H 2 , D = D 1 ⊗ 1 + γ 1 ⊗ D 2 . ( † ) Here A 1 ⊗ A 2 is the algebraic tensor product. One can also consider the case when both spectral triples are odd, however note that: � 0 ( M ) , Ω • ( M ) , D = d + d ∗ , γ = (− 1 ) degree � ◮ In the Hodge-Dirac example C ∞ the spectral triple is even whatever is the dimension of M ! ◮ In the latter case, ( † ) corresponds to equipping M = M 1 × M 2 with the product metric. 13 / 19