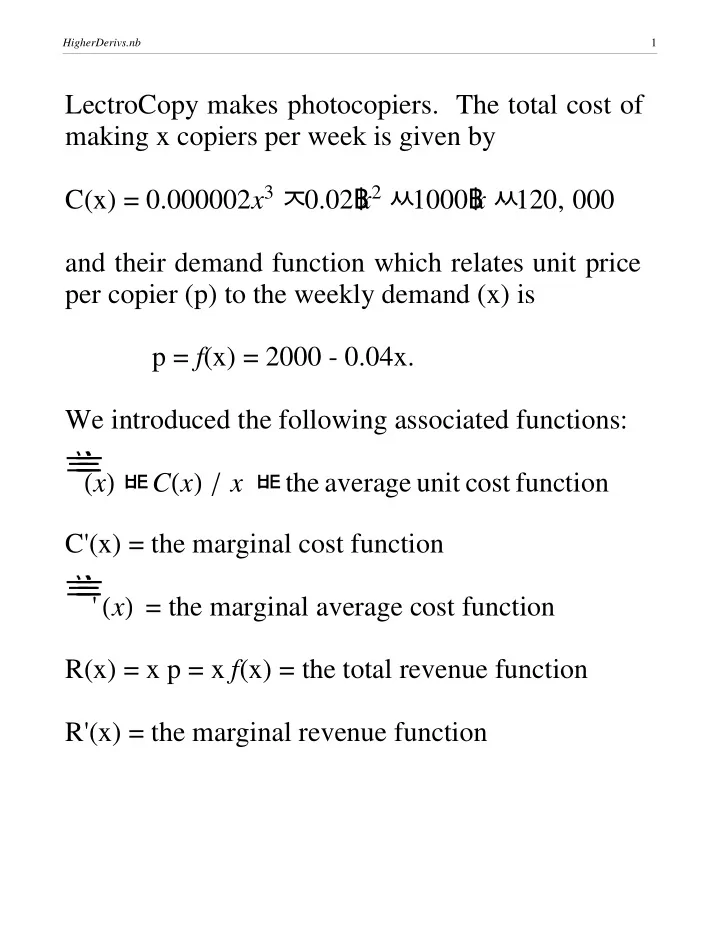

HigherDerivs.nb 1 LectroCopy makes photocopiers. The total cost of making x copiers per week is given by C(x) = 0.000002 x 3 - x 2 + 0.02 1000 x + 120, 000 and their demand function which relates unit price per copier (p) to the weekly demand (x) is p = f (x) = 2000 - 0.04x. We introduced the following associated functions: H x L = C H x L ê x = the average unit cost function ê ê ê C'(x) = the marginal cost function ' H x L = the marginal average cost function ê ê ê R(x) = x p = x f (x) = the total revenue function R'(x) = the marginal revenue function
HigherDerivs.nb 2 When you are in business, it is most important that you make a profit. Profit is what is left behind from your revenues once you've paid your costs. If costs exceed revenues then you are running at a loss and your profits are negative. We introduce the total profit function, P(x) = R(x) - C(x) = x f (x) - C(x) as revenues minus costs. P(x) provides the total dol- lar profit connected with making and selling x units of production. In our LectroCopy photocopier example it would be profit for x copiers per week: P(x) = -0.000002 x 3 - 0.02 x 2 +1000 x - 120,000 (after the math is done)
HigherDerivs.nb 3 You can check with your calculator that for x ≤ 120 we have P(x) < 0 but for x > 120 then P(x) >0. It seems that 120 copiers per week is the break even equilibrium point. Making fewer than 120 per week insures a loss, whereas making more than 120 per week guarantees a profit. Equilibrium, break-even point is when P(x) = 0. The marginal profit function, P '(x), will provide us with the additional profit generated by making and selling just one additional copier. Check that P '(3000) = $826.
HigherDerivs.nb 4 Higher Derivatives Recall that when f(x) is a function, then the associ- ated derivative function, f '(x), provides the 'rate of change of the function "f" at the point x'. Again, the derivative informs us of the rate of change of the original function. Example: C'(x), the marginal cost function, tells us how fast the total cost, C, is changing. It provides the amount by which the total cost changes per "additional" unit manufactured. The units here are important. The units of C' are dol- lars per unit, so the units for a derivative should always be: derivative units = units of function / unit of argument If C'(3000) = 934, then, when x = 3000, we know that costs change at a rate of $934 per unit.
HigherDerivs.nb 5 Standard Example: Suppose that when a rocket is launched, its height, in feet, t seconds after launch is given by s(t) = 8t + ( 3 /6) . The rate at which s changes, s'(t), is just the veloc- ity of the rising rocket, measured in feet per second. So we say the velocity of the rocket at time t sec- onds after launch is v(t) = s'(t) = 8 + ( 2 /2). The velocity function, v(t), is the derivative of the height function, s(t). Now comes the fun part. How fast is v(t) changing?
HigherDerivs.nb 6 Simply take the derivative of v(t) to measure how fast it is changing. The units in which v changes will be (units of v) / (units of t) which is units of v' = (feet per second)/(second) or feet / second 2 ("feet per second squared") This is acceleration, the rate at which velocity changes. The acceleration function, a(t) is just a(t) = v'(t) = (s'(t))' = s''(t). We call this the second derivative of s(t). Since in our rocket example we have a(t) = v'(t) = (8 + ( 2 /2))' = t, then a(3), the acceleration of the rocket at t = 3 sec- onds is 3 feet per second squared.
HigherDerivs.nb 7 Examples: Find f '''(1) if f(x) = 3 x 5 -4 x 2 +10. Suppose g'(x) = H 3 1 L 2 , find g'''(-1). x 2 +
Recommend
More recommend