Hlder spaces R n open, bounded u C 0 ( ) [0,1] | u ( x ) u - PowerPoint PPT Presentation

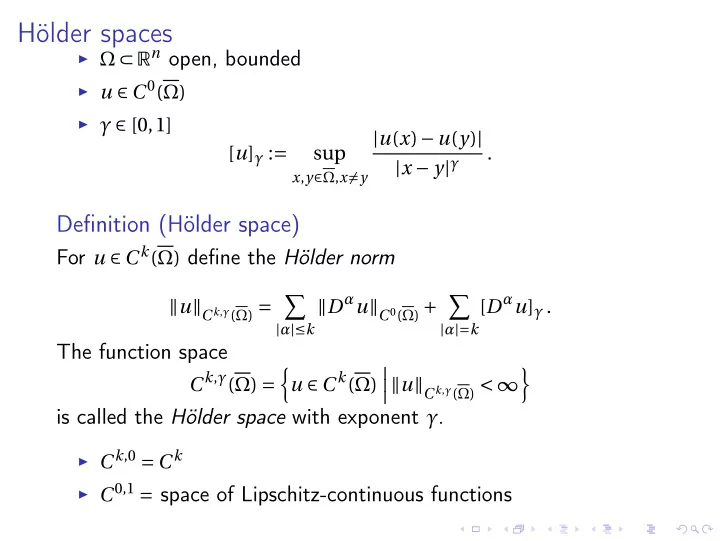
Hölder spaces � Ω ⊂ R n open, bounded � u ∈ C 0 ( Ω ) � γ ∈ [0,1] | u ( x ) − u ( y ) | [ u ] γ : = sup . | x − y | γ x , y ∈ Ω , x �= y Definition (Hölder space) For u ∈ C k ( Ω ) define the Hölder norm � � � D α u � C 0 ( Ω ) + [ D α u ] γ . � u � C k , γ ( Ω ) = | α |≤ k | α |= k The function space � � � � C k , γ ( Ω ) = u ∈ C k ( Ω ) � � u � C k , γ ( Ω ) < ∞ is called the Hölder space with exponent γ . � C k ,0 = C k � C 0,1 = space of Lipschitz-continuous functions

Theorem (Hölder space) The Hölder space with the Hölder norm is a Banach space, i. e. � C k , γ ( Ω ) is a vector space, � �·� C k , γ ( Ω ) is a norm, � any Cauchy sequence in the Hölder space converges. Proof. Homework!

Weak derivative � u , v ∈ L 1 loc ( Ω ) � α a multiindex � C ∞ c ( Ω ) = infinitely smooth functions with compact support in Ω Definition v is called the α th weak derivative of u , D α u = v , if � � uD α ψ d x = ( − 1) | α | (1) v ψ d x Ω Ω for all test functions ψ ∈ C ∞ c ( Ω ) . Remark � (1) � = k times integration by parts � u smooth ⇒ v = D α u is classical derivative

Weak derivative Example (on Ω = (0,2) ) � x if 0 < x ≤ 1 1. � u ( x ) = 1 if 1 < x < 2 � 1 if 0 < x ≤ 1 � v ( x ) = 0 if 1 < x < 2 v = Du , since for any ψ ∈ C ∞ c ( Ω ) � 2 � 2 u ψ ′ d x = ... = − v ψ d x , 0 0 � x if 0 < x ≤ 1 2. � u ( x ) = 2 if 1 < x < 2 u does not have a weak derivative, since � 2 � 1 − v ψ d x = ... = − ψ d x − ψ (1) 0 0 cannot be fulfilled for all ψ ∈ C ∞ c ( Ω ) by any v ∈ L 1 loc ( Ω )

Lebesgue spaces Definition (Lebesgue space) Let p ∈ [1, ∞ ] . ��� � 1/ p Ω | u | p d x ( p < ∞ ) � u � L p ( Ω ) = esssup Ω | u | ( p = ∞ ) The Lebesgue space with exponent p is � � � L p ( Ω ) = � u measurable with � u � L p ( Ω ) < ∞ u : Ω → R . Theorem (Lebesgue space) L p ( Ω ) is a Banach space.

Sobolve spaces Definition (Sobolev space) Let p ∈ [1, ∞ ] , k ∈ N 0 . The space � � � W k , p ( Ω ) = u ∈ L 1 � weak derivative D α u ∈ L p ( Ω ) for all | α | ≤ k loc ( Ω ) ��� � � 1/ p Ω | D α u | p d x 1 ≤ p < ∞ | α |≤ k with � u � W k , p ( Ω ) = � | α |≤ k esssup Ω | D α u | p = ∞ is called a Sobolev space . Theorem (Sobolev space) W k , p ( Ω ) is a Banach space. Remark � W 0, p ( Ω ) ≡ L p ( Ω ) � W k , p c ( Ω ) in W k , p ( Ω ) ( Ω ) = closure of C ∞ 0 � H k ( Ω ) ≡ W k ,2 ( Ω ) are Hilbert spaces (what is inner product?)

Properties of Lebesgue and Sobolev functions Theorem (Hölder’s inequality) � � p , p ∗ ∈ [1, ∞ ] with 1 � p + 1 p ∗ = 1 ⇒ | f g | d x ≤ � f � L p ( Ω ) � g � L p ∗ ( Ω ) � f ∈ L p , g ∈ L p ∗ Ω Theorem (Trace theorem) Let Ω ⊂ R n bounded, ∂ Ω Lipschitz. There exists a continuous linear operator T : W 1, p ( Ω ) → L p ( ∂ Ω ) , the trace, with (i) Tu = u | ∂ Ω if u ∈ W 1, p ( Ω ) ∩ C 0 ( Ω ) , (ii) � Tu � L p ( ∂ Ω ) ≤ C � u � W 1, p ( Ω ) , u ∈ W 1, p (iii) Tu = 0 ( Ω ) . ⇔ 0 Theorem (Poincaré’s inequality) Ω ⊂ R n bounded, open, connected, ∂ Ω Lipschitz. ∃ C = C ( n , p , Ω ) � ∀ u ∈ W 1, p ( Ω ) � u − Ω u d x � L p ( Ω ) ≤ C �∇ u � L p ( Ω ) ∀ u ∈ W 1, p � u � L p ( Ω ) ≤ C �∇ u � L p ( Ω ) ( Ω ) 0

Embedding theorems Theorem (Sobolev embedding) Ω ⊂ R n open, bounded, ∂ Ω Lipschitz, m 1 , m 2 ∈ N 0 , p 1 , p 2 ∈ [1, ∞ ) . If m 1 ≥ m 2 and m 1 − n p 1 ≥ m 2 − n p 2 then W m 1 , p 1 ( Ω ) ⊂ W m 2 , p 2 ( Ω ) and there is a constant C > 0 s. t. � u � W m 1, p 1 ( Ω ) ≤ C � u � W m 2, p 2 ( Ω ) ∀ u . If the inequalities are strict, W m 1 , p 1 ( Ω ) � → W m 2 , p 2 ( Ω ) compactly. Theorem (Hölder embedding) Ω ⊂ R n open, bounded, ∂ Ω Lipschitz, m , k ∈ N 0 , p ∈ [1, ∞ ) , α ∈ [0,1] . If m − n p ≥ k + α and α �= 0,1 then W m , p ( Ω ) ⊂ C k , α ( Ω ) and there is a constant C > 0 s. t. � u � W m , p ( Ω ) ≤ C � u � C k , α ( Ω ) ∀ u . If m − n p < k + α , W m , p ( Ω ) � → C k , α ( Ω ) compactly.