dU = T dS P dV + dN Different phases gas to liquid to solid - PowerPoint PPT Presentation

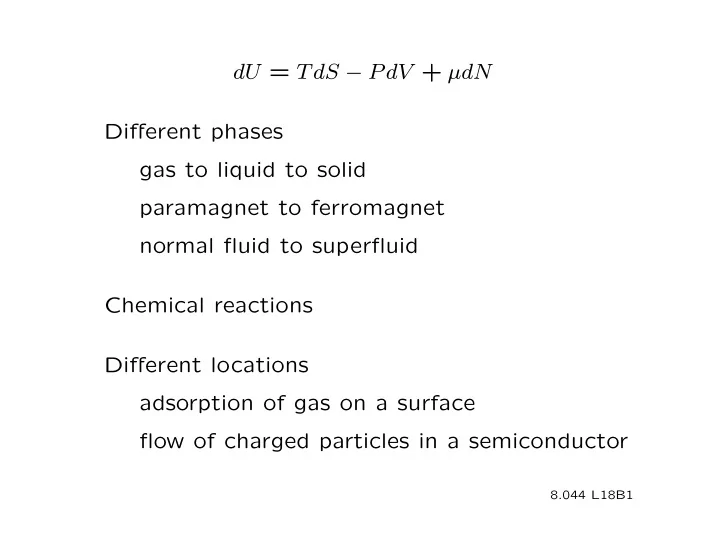
dU = T dS − P dV + µdN Different phases gas to liquid to solid paramagnet to ferromagnet normal fluid to superfluid Chemical reactions Different locations adsorption of gas on a surface flow of charged particles in a semiconductor 8.044 L18B1

Note that ∂U � � µ = ∂N S,V ↑ This is often a source of miss-understanding. However F ≡ U − TS ⇒ dF = dU − T dS − SdT dF = − SdT − P dV + µdN So ∂F � � µ = ∂N T,V 8.044 L18B2

�� �� � � ��� � ��� � � � ��� � ��� � dS = 1 T dU + P T dV − µ T dN = 1 + 1 ( − dU 2 ) − µ 1 dU 2 − µ 2 ( − dN 2 ) dN 2 T 1 T 1 T 2 T 2      1 − 1  µ 1 − µ 2 =  dU 2 +  dN 2 ≥ 0 T 2 T 1 T 1 T 2 8.044 L18B3

If T 1 > T 2 , energy flows to the right. If T 1 = T 2 there is no energy flow. If the two sides are at the same temperature and µ 1 > µ 2 particles flow to the right. If T 1 = T 2 and µ 1 = µ 2 there is neither energy flow nor particle flow and one has an equilibrium situation. 8.044 L18B4

Example: Adsorption ε ���� ε ����� � ε � ��������������� ������������ ������������������������ ��������� 8.044 L18B5

3D gas V 2 2 2 dp x dp y dp z /h 3 = λ 3 ( T ) � − ( p x + p y + p ) / 2 mk B T Z 1 = V e z 1 N Z = Z 1 N ! F = − k B T ln Z = − k B T ( N ln Z 1 − N ln N + N ) ∂F � � µ = = − k B T (ln Z 1 − N/N − ln N + 1) ∂N V,T V 1 N � � � � λ 3 ( T ) = − k B T ln = k B T ln N λ 3 V 8.044 L18B6

2D gas on surface with binding energy E 0 2 2 � E 0 /k B T − ( p x + p y ) / 2 mk B T dp x dp y /h 2 Z 1 = A e e A E 0 /k B T = e λ 2 ( T ) ⎛ ⎞ Z 1 E 0 /k B T A 1 � � µ = − k B T ln = − k B T ln ⎝ e ⎠ N λ 2 ( T ) N N � � λ 2 ( T ) = − E 0 + k B T ln A 8.044 L18B7

Define the number density in the bulk as n ≡ N/V and on the surface as σ ≡ N/A . In equilibrium µ surface = µ bulk − � 0 + k B T ln σ λ 2 ( T ) = k B T ln n λ 3 ( T ) � � � � ln σ λ 2 ( T ) = � 0 /k B T + ln n λ 3 ( T ) � � � � σ λ 2 ( T ) = e � 0 /k B T n λ 3 ( T ) � 0 /k B T σ = λ ( T ) e n 8.044 L18B8

h � 0 /k B T σ = √ e n 2 πmk B T 8.044 L18B9

Ensembles • Microcanonical: E and N fixed Starting point for all of statistical mechanics Difficult to obtain results for specific systems • Canonical: N fixed, T specified; E varies Workhorse of statistical mechanics • Grand Canonical: T and µ specified; E and N vary Used when the the particle number is not fixed 8.044 L18B1 0

� � �������������������������������� � ������������������������������������������������ � ����������������������������������������������� � ������������������������������������������������� ������������������������ �������� 8 � 11

For the entire system (microcanonical) one has volume of accessible phase space consistent with X p ( system in state X ) = Ω( E ) In particular, for our case p ( { p 1 , q 1 , N 1 } ) ≡ p ( subsystem at { p 1 , q 1 , N 1 } ; remainder undetermined ) Ω 1 ( { p 1 , q 1 , N 1 } ) Ω 2 ( E − E 1 , N − N 1 ) = Ω( E, N ) 8.044 L18B 12

k ln p ( { p 1 , q 1 , N 1 } ) = k ln Ω 1 − k ln Ω( E, N ) f ( ) f ( ) k ln 1 = 0 S ( E, N ) + k ln Ω 2 ( E − E 1 , N − N 1 ) f ( ) S 2 ( E − E 1 , N − N 1 ) 8.044 L18B 13

⎛ ⎞ ∂S 2 S 2 ( E − E 1 , N − N 1 ) ≈ S 2 ( E, N ) − E 1 ⎝ ⎠ ∂E 2 N 2 , 2 1 /T ⎛ ⎞ ∂S 2 − ⎝ N 1 ⎠ ∂N 2 E 2 , 2 − µ/T = S 2 ( E, N ) − H 1 ( { p 1 , q 1 , N 1 } /T + µN 1 /T 8.044 L18B 14

H 1 ( { p 1 , q 1 , N 1 } ) µN 1 k ln p ( { p 1 , q 1 , N 1 } ) = − + T T + S 2 ( E, N ) − S ( E, N ) The first line on the right depends on the specific state of the subsystem. The second line on the right depends on the reser- voir and the average properties of the subsystem. 8.044 L18B 15

¯ 1 , N ¯ 1 ) + S 2 ( E ¯ 2 , N ¯ 2 ) S ( E, N ) = S 1 ( E S 2 ( E, N ) − S ( E, N ) [ S 2 ( E, N ) − S 2 ( ¯ E 2 , ¯ N 2 )] − S 1 ( ¯ E 1 , ¯ = N 1 )      ∂S 2  ∂S 2 ¯ N 1 ] − S 1 ( ¯ ¯ E 1 , ¯ = [ E 1 + N 1 )   ∂E 2 ∂N 2 N 2 E 2 [ ¯ E 1 /T − µ ¯ N 1 /T ] − S 1 ( ¯ E 1 , ¯ = N 1 ) = ( ¯ E 1 − µ ¯ N 1 − T S 1 ) /T = ( F 1 − µ ¯ N 1 ) /T 8.044 L18B 16

H 1 ( { p 1 , q 1 , N 1 } ) + µN 1 k ln p ( { p 1 , q 1 , N 1 } ) = − T T +( F 1 − µ ¯ N 1 ) /T exp[ β ( µN 1 − H )] exp[ β ( F 1 − µ ¯ p ( { p 1 , q 1 , N 1 } ) = N 1 ) exp[ β ( µN − H )] exp[ β ( F − µ ¯ p ( { p, q, N } ) = N )] exp[ β ( µN − H )] / exp[ − β ( F − µ ¯ = N )] 8.044 L18B 17

∞ e p ( { p, q, N } ) { dp, dq } = 1 N =1 exp[ β ( µN − H )] p ( { p, q, N } ) = Z ∞ Z ( T, V, µ ) = e exp[ β ( µN − H )] { dp, dq } N =1 ∞ e ( e βµ ) N Z ( T, V, N ) = N =1 ¯)] = exp[ − β ( F − µN 8.044 L18B 18

∞ ⎛ ⎞ ∂ Z e = exp[ β ( µN − H )] { dp, dq } βN ⎝ ⎠ ∂µ N =1 T,V ∞ ⎛ ⎞ ⎛ ⎞ 1 ∂ Z exp[ β ( µN − H )] = e ⎠ { dp, dq } N ⎝ ⎠ ⎝ β Z ∂µ Z N =1 T,V ∞ ⎛ ⎞ 1 ∂ Z e = p ( { p, q } , N ) { dp, dq } N ⎝ ⎠ β Z ∂µ T,V N =1 ⎛ ⎞ 1 ∂ ln Z = < N > ⎝ ⎠ β ∂µ T,V 8.044 L18B 19

Define a new thermodynamic potential, the ”Grand potential”, Φ G . ¯ = U − TS − µN ¯ Φ G ≡ F − µN ¯ − Ndµ ¯ d Φ G = dF − µ d N ¯ = − SdT − P dV − Ndµ 8.044 L18B 20

Then the connection between statistical mechan- ics and thermodynamics in the Grand Canonical Ensemble is through the Grand potential ∂ Φ G � � S = − ∂T V, µ ∂ Φ G � � P = − ∂V T, µ ⎛ ⎞ ∂ Φ G ¯ N = − ⎝ ⎠ ∂µ T,V 8.044 L18B 21

Specification of a symmetrically allowed many body state. Indicate which single particle states, α, β, γ, · · · , are used and how many times. { n α , n β , n γ , · · ·} An ∞ # of entries, each ranging from 0 to N for Bosons and 0 to 1 for Fermions, but with the restriction that α n α = N � 8.044 L18B 22

| 1 , 0 , 1 , 1 , 0 , 0 , · · ·) Fermi-Dirac | 2 , 0 , 1 , 3 , 6 , 1 , · · ·) Bose-Einstein ' E α n α = E � � Prime indicates n α = N α α 8.044 L18B 23

Statistical Mechanics Try Canonical Ensemble − E ( state ) /kT Z ( N, V, T ) = � e states ↔ − E ( { n α } ) /kT � = e { n α } � � ↔ − E α n α /kT � � = e α { n α } This can not be carried out. One can not interchange the � over occupation numbers and the � over states because the occupation numbers are not independent ( n α = N ). � 8.044 L 18 B 24

Statistical Mechanics Grand Canonical Ensemble [ µN − E ( state )] /kT Z ( T, V, µ ) = e states [ µN − E ( { n α } )] /kT = e { n α } � � e ( µ − E α ) n α /kT = α { n α } ⎛ ⎞ ( µ − E α ) n α /kT ⎟ = e ⎜ ⎝ ⎠ α { n α } 8.044 L18B 25

For Fermions n α = 0 , 1 ( µ − E α ) n α /kT ( µ − E α ) β = 1 + e e { n α } � � ( µ − E α ) β ln Z = ln 1 + e α For Bosons n α = 0 , 1 , 2 , · · · � − 1 � ( µ − E α ) β ] n α ( µ − E α ) β [ e = 1 − e { n α } � � ( µ − E α ) β ln Z = − ln 1 − e α 8.044 L18B 26

< n α > < N > = α ⎛ ⎞ = 1 ⎝ ∂ ln Z ⎠ β ∂µ T,V ( µ − E α ) β e = { + F-D , − B-E } ( µ − E α ) β α 1 ± e 1 < n α > = e ( E α − µ ) β ± 1 8.044 L18B27

MIT OpenCourseWare http://ocw.mit.edu 8.044 Statistical Physics I Spring 2013 For information about citing these materials or our Terms of Use, visit: http://ocw.mit.edu/terms.