dt < | ( ) | h t (this has to do with system stability - PDF document

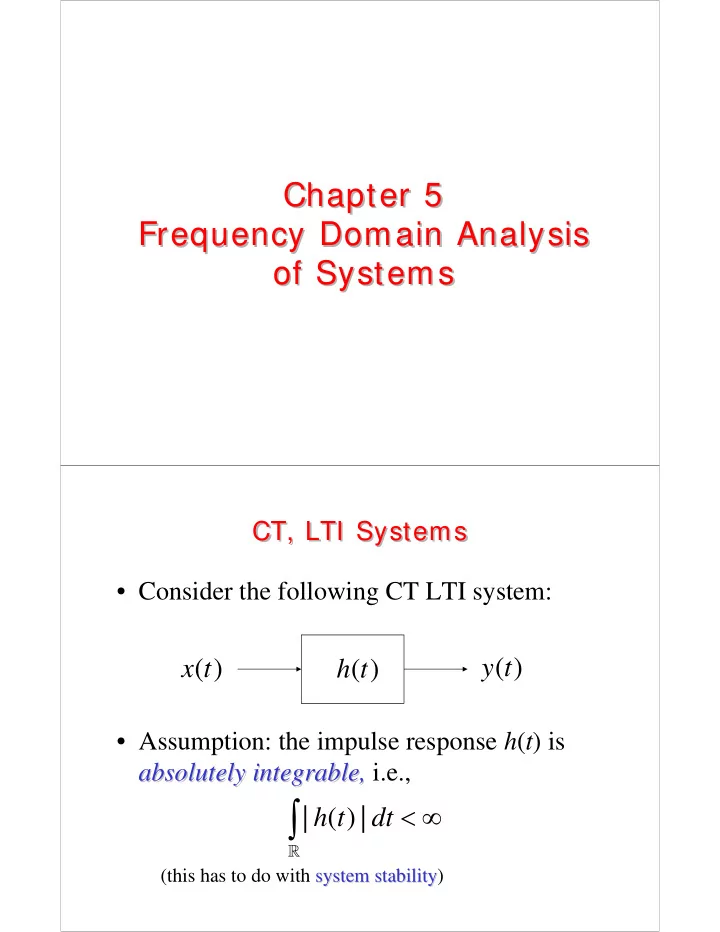
Chapter 5 Chapter 5 Frequency Domain Analysis Frequency Domain Analysis of Systems of Systems CT, LTI Systems CT, LTI Systems • Consider the following CT LTI system: ( ) ( ) ( ) y t x t h t • Assumption: the impulse response h ( t ) is , i.e., absolutely integrable integrable, absolutely ∫ dt < ∞ | ( ) | h t � (this has to do with system stability system stability)

Response of a CT, LTI System to a Response of a CT, LTI System to a Sinusoidal Input Sinusoidal Input • What’s the response y ( t ) of this system to the input signal = ω + θ ∈ � ( ) cos( ), ? x t t t 0 • We start by looking for the response y c ( t ) of the same system to ω = ∈ � j t ( ) x t e t 0 c Response of a CT, LTI System to a Response of a CT, LTI System to a Complex Exponential Input Complex Exponential Input • The output is obtained through convolution as ∫ = ∗ = τ − τ τ = ( ) ( ) ( ) ( ) ( ) y t h t x t h x t d c c c � ∫ ω − τ = τ τ = ( ) j t ( ) h e d 0 � ∫ ω − ω τ = τ τ = j t j ( ) e h e d � 0 0 x ( ) t � c ∫ − ω τ = τ τ j ( ) ( ) x t h e d 0 c �

The Frequency Response of a CT, The Frequency Response of a CT, LTI System LTI System • By defining H ω is the frequency ( ) = ∫ − ωτ ω τ τ response of the CT, j ( ) ( ) H h e d LTI system = Fourier � transform of h(t ) it is = ω = ( ) ( ) ( ) y t H x t 0 c c ω = ω ∈ � j t ( ) , H e t 0 0 • Therefore, the response of the LTI system to a complex exponential is another complex ω exponential with the same frequency 0 Analyzing the Output Signal y c ( t ) Analyzing the Output Signal y c ( t ) H ω ( ) • Since is in general a complex 0 quantity, we can write ω = ω = j t ( ) ( ) y t H e 0 0 c ω ω = ω = arg ( ) j H j t | ( ) | H e e 0 0 0 ω + ω = � ω � � � � � ( arg ( )) j t H | ( ) | H e 0 0 � �� � 0 output signal’s output signal’s phase magnitude

Response of a CT, LTI System to a Response of a CT, LTI System to a Sinusoidal Input Sinusoidal Input • With Euler’s formulas we can express x(t) as = ω + θ ( ) cos( ) x t t 0 ω + θ − ω + θ = + ( ) ( ) j t j t 1 ( ) e e 0 0 2 ω − ω θ − θ = + j j t j j t 1 1 e e e e 0 0 2 2 Using the previous result, the response is θ ω − θ − ω = ω + − ω j j t j j t 1 1 ( ) ( ) ( ) y t e H e e H e 0 0 0 0 2 2 Response of a CT, LTI System to a Response of a CT, LTI System to a Sinusoidal Input – Cont’d Sinusoidal Input – Cont’d − ω = ω • If h(t) is real, then and * ( ) ( ) H H ω = ω ω arg ( ) j H ( ) | ( ) | H H e 0 0 0 − ω − ω = ω j arg H ( ) ( ) | ( ) | H H e 0 0 0 • Thus we can write y(t) as 1 1 = ω ω + + θ ω + ω − ω + + θ ω ( arg ( )) ( arg ( )) j t H j t H ( ) | ( ) | | ( ) | y t H e H e 0 0 0 0 0 0 2 2 = ω ω + θ + ω | ( ) | cos( arg ( )) H t H 0 0 0

Response of a CT, LTI System to a Response of a CT, LTI System to a Sinusoidal Input – Cont’d Sinusoidal Input – Cont’d • Thus, the response to = ω + θ ( ) cos( ) x t A t 0 is ( ) = ω ω + θ + ω ( ) | ( ) | cos arg ( ) y t A H t H 0 0 0 which is also a sinusoid with the same ω frequency but with the amplitude amplitude scaled by scaled by 0 H ω | ( ) | the factor and with the phase shifted the factor 0 H ω arg ( ) by amount 0 Example: Response of a CT, LTI Example: Response of a CT, LTI System to Sinusoidal Inputs System to Sinusoidal Inputs • Suppose that the frequency response of a CT, LTI system is defined by the following specs: H ω ≤ ω ≤ | ( ) | ⎧ 1.5, 0 20, 1.5 ω = ⎨ | ( ) | H ω > ⎩ 0, 20, 0 ω 20 H ω arg ( ) H ω = − ∀ ω � ω arg ( ) 60 , − � 60

Example: Response of a CT, LTI Example: Response of a CT, LTI System to Sinusoidal Inputs – System to Sinusoidal Inputs – Cont’d Cont’d • If the input to the system is = + + + � � ( ) 2cos(10 90 ) 5cos(25 120 ) x t t t • Then the output is = + � + + ( ) 2 | (10) | cos(10 90 arg (10) ) y t H t H + + + = � 5 | (2 5) | cos(25 120 arg (25) ) H t H = + � 3cos (10 30 ) t Example: Frequency Analysis of an Example: Frequency Analysis of an RC Circuit RC Circuit • Consider the RC circuit shown in figure

Example: Frequency Analysis of an Example: Frequency Analysis of an RC Circuit – Cont’d RC Circuit – Cont’d • From EEE2032F, we know that: 1. The complex impedance complex impedance of the capacitor is ω 1/ j C equal to e ω = j t ( ) 2. If the input voltage is , then the x t c output signal is given by ω 1/ 1/ j C RC ω ω = = j t j t ( ) y t e e + ω ω + c 1/ 1/ R j C j RC Example: Frequency Analysis of an Example: Frequency Analysis of an RC Circuit – Cont’d RC Circuit – Cont’d ω = ω • Setting , it is 0 1/ RC ω = e ω = j t j t ( ) y t e 0 ( ) x t 0 and and ω + c c 1/ j RC 0 whence we can write = ω ( ) ( ) ( ) y t H x t 0 c c where 1/ RC ω = ( ) H ω + 1/ j RC

Example: Frequency Analysis of an Example: Frequency Analysis of an RC Circuit – Cont’d RC Circuit – Cont’d 1/ RC ω = | ( ) | H ω + 2 2 (1/ ) RC ( ) ω = − ω arg ( ) arctan H RC RC = 1/ 1000 Example: Frequency Analysis of an Example: Frequency Analysis of an RC Circuit – Cont’d RC Circuit – Cont’d • The knowledge of the frequency response H ω ( ) allows us to compute the response y ( t ) of the system to any sinusoidal input signal = ω + θ ( ) cos( ) x t A t 0 since ( ) = ω ω + θ + ω ( ) | ( ) | cos arg ( ) y t A H t H 0 0 0

Example: Frequency Analysis of an Example: Frequency Analysis of an RC Circuit – Cont’d RC Circuit – Cont’d RC = 1/ 1000 • Suppose that and that = + ( ) cos(100 ) cos(3000 ) x t t t • Then, the output signal is = + + ( ) | (100) | cos(100 arg (100) ) y t H t H + + = | (30 00) | cos(3000 arg (3000 ) ) H t H = − + − � � 0.9950cos(10 0 5.71 ) 0.3162cos(3 000 7 1.56 ) t t Example: Frequency Analysis of an Example: Frequency Analysis of an RC Circuit – Cont’d RC Circuit – Cont’d ( ) x t ( ) y t

Example: Frequency Analysis of an Example: Frequency Analysis of an RC Circuit – Cont’d RC Circuit – Cont’d • Suppose now that = + ( ) cos(100 ) cos(50,000 ) x t t t •Then, the output signal is = + + ( ) | (100) | cos(100 arg (100) ) y t H t H + + = | (50,000) | cos(50,000 arg (50,000) ) H t H = − + − � � 0.9950cos(10 0 5.71 ) 0.0200cos(50,000 88.85 ) t t Example: Frequency Analysis of an Example: Frequency Analysis of an RC Circuit – Cont’d RC Circuit – Cont’d ( ) ( ) x t y t The RC circuit behaves as a lowpass lowpass filter filter, by letting low- frequency sinusoidal signals pass with little attenuation and by significantly attenuating high-frequency sinusoidal signals

Response of a CT, LTI System to Response of a CT, LTI System to Periodic Inputs Periodic Inputs • Suppose that the input to the CT, LTI system is a periodic signal periodic signal x ( t ) having period T • This signal can be represented through its Fourier series as Fourier series ∞ ∑ ω = ∈ � x jk t ( ) , x t c e t 0 k =−∞ k + where t T 0 1 ∫ − ω = ∈ jk t � x ( ) , c x t e dt k 0 k T t 0 Response of a CT, LTI System to Response of a CT, LTI System to Periodic Inputs – Cont’d Periodic Inputs – Cont’d • By exploiting the previous results and the linearity of the system, the output of the system is ∞ ∑ ω = ω jk t x ( ) ( ) y t H k c e 0 0 k =−∞ k ∞ ∑ ω + �� + �� � ω = ω x = ( ar g( ) arg ( ) ) j k t c H k x | ( ) | | | H k c e 0 k 0 �� � ��� � 0 k y arg =−∞ c k k y | | c k ∞ ∞ ∑ ∑ ω + ω y = = ∈ ( arg( ) ) j k t c � y y jk t | | , c e c e t 0 0 k k k =−∞ =−∞ k k

Example: Response of an RC Circuit Example: Response of an RC Circuit to a Rectangular Pulse Train to a Rectangular Pulse Train • Consider the RC circuit ∑ = − with input ( ) rect( 2 ) x t t n ∈ � n Example: Response of an RC Circuit to Example: Response of an RC Circuit to a Rectangular Pulse Train – Cont’d a Rectangular Pulse Train – Cont’d ∑ = − ( ) rect( 2 ) x t t n ∈ � n • We have found its Fourier series to be ∑ π = ∈ � x jk t ( ) , x t c e t k ∈ � k with ⎛ ⎞ 1 sinc k = x ⎜ ⎟ c k ⎝ ⎠ 2 2