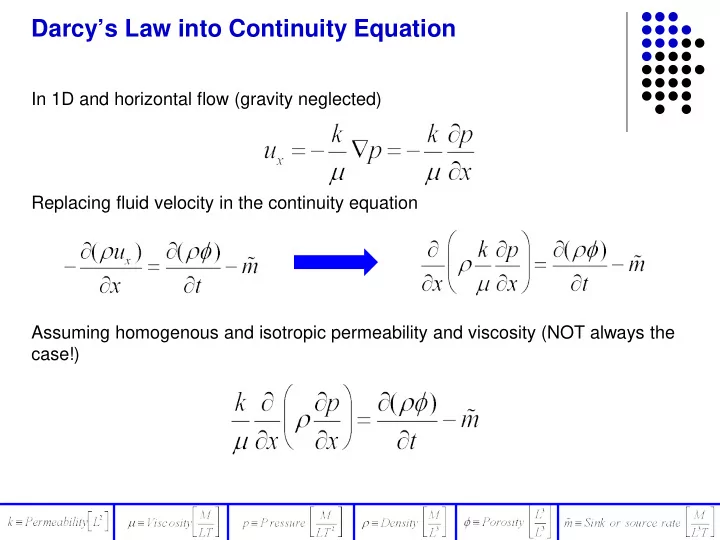
Darcys Law into Continuity Equation In 1D and horizontal flow - PowerPoint PPT Presentation

Darcy’s Law into Continuity Equation In 1D and horizontal flow (gravity neglected) Replacing fluid velocity in the continuity equation Assuming homogenous and isotropic permeability and viscosity (NOT always the case!)

Formation Volume Factor (B w ) We measure volume at surface but do the mass balance at the reservoir Surface/Standard Conditions = = = ρ P 14.7 psi; T 60 °F; V V sc ρ = SC RC depth B w Reservoir Conditions = = = P P ; T T ; V V R R R Formation volume factor and ρ depend on both pressure and temperature • B o = formation volume factor for oil (> 1.0) • B g = formation volume factor for gas (<< 1.0) • B w = formation volume factor for water (~1.0)

Formation Volume Factor—Continuity Equation At reservoir conditions, density is: Replacing density in continuity equation and divide through by ρ SC (a constant)     ∂ ∂ ∂ φ  k 1 p m = −     µ ∂ ∂ ∂ ρ     x B x t B w w sc Using the product rule on the left-hand side of the equation       ∂ ∂ ∂ ∂ φ  2 k 1 p 1 p m + = −       µ ∂ ∂ ∂ ∂ ρ 2      B x x B x  t B w w w sc …And chain rule on the left hand side,       ∂ ∂ ∂ 2 ∂ φ    2 k 1 p 1 p m + = −         µ ∂ ∂ ∂ ∂ ρ   2       B x p B x t B   w w w sc

Expansion of the Time Derivative Chain and product rule on time derivative (right hand side)         ∂ ∂ ∂ 2 ∂ ∂ φ ∂    2 k 1 p 1 p 1 1 p m + = φ + −           µ ∂ ∂ ∂ ∂ ∂ ∂ ρ   2       B x p B x  p B B p  t   w w w w sc A few definitions: ∂ ∂ φ 1 V 1 = = P c (rock compressibility) ∂ φ ∂ r V p p P   ∂ ∂ ρ ∂ − ∂ 1 V 1 1 1 B = − = = =   w c B (fluid compressibility) ∂ ρ ∂ ∂ ∂ f w   V p p p B B p w w T T = + c c c (total compressibility) t r f With some manipulation:             ∂ ∂ ∂ 2 φ ∂ ∂ φ ∂    2 k 1 p 1 1 P 1 1 p m     + = + −       B B µ ∂ ∂ ∂ ∂ φ ∂ ∂ ρ     w   w 2     B x B p B x B p B p t    w w w w w sc         c c c r f f     2 2 LT LT ≡ ≡   c rock compressibility   c fluid compressibility r f     M M

1D Diffusivity Equation ≈ 0 slightly compressible fluid   ∂ ∂ 2 φ ∂    2 c k 1 p p c p m + = − f   t   µ ∂ ∂ ∂ ρ 2     B x B x B t   w w w sc If the fluid is “slightly compressible” (liquid), the compressibility is small (< 10 -5 ) and constant and terms involving can be ignored. 1D diffusivity (with homogenous fluid and reservoir properties) can be written: k ≡ α = Diffusivity constant µφ c t k ≡ λ = Mobility µ B w  m ≡ =  Source q ρ SC SC If no sources or sinks (wells) are present, we get the “heat equation” ∂ ∂ 2 p 1 p = ∂ α ∂ 2 x t

Generalized Diffusivity (Heat) Equation In 2D (x-y plane) In 3D and potential Φ accounting for gravity

Slightly Compressible Fluids: Liquids P1 P2 More Pressure V1 V2 Recall fluid compressibility factor (c f ) at constant temperature   ∂ ∂ ρ ∂ − ∂ B 1 V 1 1 1 = − = = =   w c B ∂ ρ ∂ ∂ ∂ f w   V p p p B B p w w T T Integrating from a reference point (p 0 , ρ 0 ), to any other point ρ 1 ∫ p ∫ = ρ p c dp d ρ f ρ 0 0

Taylor Series Expansion 1 1 ′ ′′ + ∆ = + ∆ + ∆ + ∆ + 2 3 f x ( x ) f x ( ) f ( ) x x f ( ) x x f "'( ) x x ... 2! 3! Using Taylor series to expand density around a reference density, ∂ ρ 1 1 ρ + ∆ = ρ + ∆ + ρ ∆ + ρ ∆ + 0 0 0 0 2 0 3 ( p p ) ( p ) ( p ) p "( p ) p "( p ) p ... ∂ p 2! 3! ∂ ρ ( ) − 0 0 = ρ = ρ c ( p p ) 0 0 0 p c e f c Differentiate exponential equation for density: ∂ f f p For slightly compressible (c f < 10 -5 psi -1 ) liquids, higher order terms are small: Negligible for small c f   1 1 ρ + ∆ = ρ + ρ ∆ + ρ ∆ + ρ ∆ + 0 0 0 2 0 2 3 0 3 ( p p ) c p c p c p ...   f f f   2! 3! Therefore, 1 B ≈ + − ρ   ρ ≈ + − 0 0 0 1 c ( p p ) 1 c ( p p ) 0 =1 (assume reference is   B w f f standard conditions) w

Simple 1D Problem: Core Flooding P B1 P B2 “Heat” Equation p=p init ∂ ∂ 2 p 1 p = ∂ α ∂ 2 x t x=L x=0 ( ) = = p x ,0 p 0.0 psi init ( ) = = p 0, t p 1000.0 psi B 1 ( ) = = p L t , p 0.0 psi B 2 Analytical Solution to PDE α + π n 2 − + π ∞ (2 n 1) t n 4 p ( 1) (2 n 1) x ∑ ( ) = − 2 init p x t , p e 4 L cos π + B 1 2 n 1 2 L = n 0

Analytical Solution α + π n 2 − + π ∞ (2 n 1) t n 4 p ( 1) (2 n 1) x ∑ ( ) = − 2 init p x t , p e 4 L cos π + B 1 2 n 1 2 L = n 0 Steady state solution Time increasing

Real Reservoirs That was the easy solution… Real reservoirs have: Spatially varying permeability, porosity, etc. • Time-varying viscosity, formation volume factor • Geometries that are 2D or 3D and are not on a regular grid • Sources and sinks (called wells) spaced irregularly throughout the reservoir • Complex boundary conditions •   φ ∂ c k p ( ) ∇ ∇ + ρ ∇ = −    t p g z q µ ∂ SC   B B t w w (and this is just for single phase flow…)

Solving “ugly” PDE So how do we solve this complex, nonhomogeneous, 3D PDE? 1. Break the reservoir into manageable blocks that have contain reservoir and fluid properties 2. Write algebraic equations for each block by “discretizing” PDE − = 3 TP TP Q 1 2 1 − + − = TP 2 TP TP Q 1 2 3 2 − + − = TP 2 TP TP Q 2 3 4 3  − + = TP TP Q − N 1 N N 3. Solve system of linear equations   B B + + + = + n 1 n 1   T P P Q ∆ ∆   t t