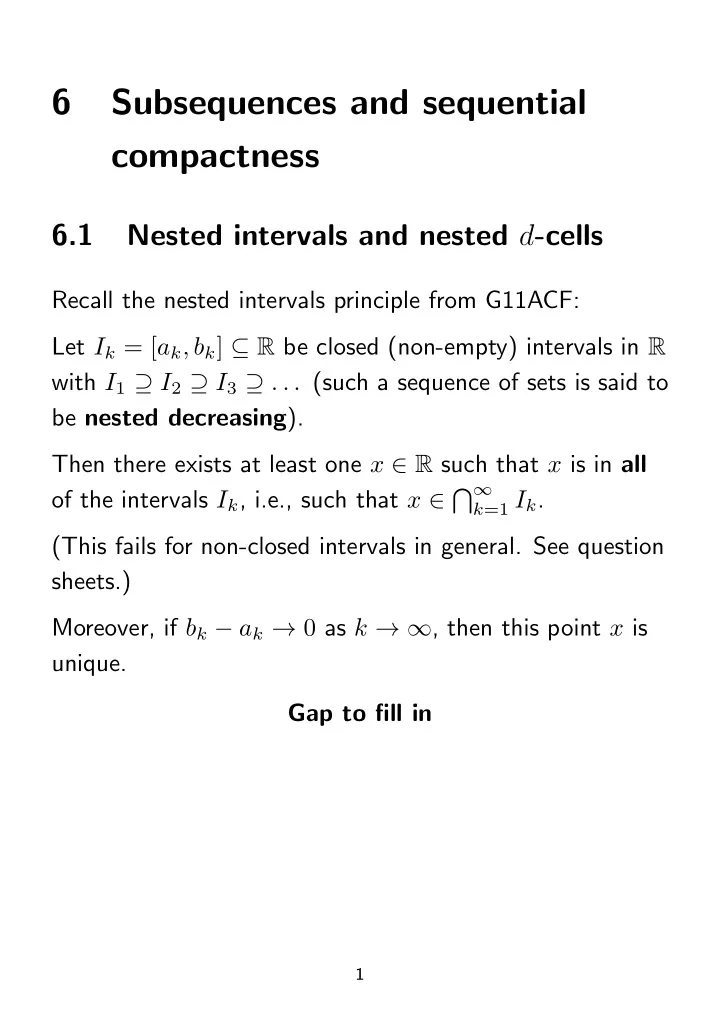

6 Subsequences and sequential compactness 6.1 Nested intervals and nested d -cells Recall the nested intervals principle from G11ACF: Let I k = [ a k , b k ] ⊆ R be closed (non-empty) intervals in R with I 1 ⊇ I 2 ⊇ I 3 ⊇ . . . (such a sequence of sets is said to be nested decreasing ). Then there exists at least one x ∈ R such that x is in all of the intervals I k , i.e., such that x ∈ � ∞ k =1 I k . (This fails for non-closed intervals in general. See question sheets.) Moreover, if b k − a k → 0 as k → ∞ , then this point x is unique. Gap to fill in 1
Before discussing the d -dimensional analogue of this result, let us first look at the condition that b k − a k → 0 as k → ∞ . Note that b k − a k = max {| x − y | : x, y ∈ [ a k , b k ] } . In general, for a non-empty, bounded subset A of R d , we define the diameter of A , diam ( A ) , by diam ( A ) = sup {� x − y � : x , y ∈ A } the supremum of the possible distances between pairs of points of A . From earlier results we know that 0 ≤ diam ( A ) < ∞ for non-empty bounded sets. For (non-empty, bounded) closed d -cells , this supremum is actually a maximum , and this maximum is achieved by considering any pair of diametrically opposite corners of the d -cell. Gap to fill in 2
Traditionally, when authors discuss ‘closed intervals’ in R , they mean intervals of the form [ a, b ] where a and b are real numbers with a ≤ b , and not unbounded intervals such as [0 , ∞ [ . For the rest of this chapter, whenever we discuss ‘closed intervals’ (or ‘closed d -cells’), we will follow this traditional terminology, so that we will mean non-empty, closed and bounded intervals (or d -cells). We now come to the d -dimensional analogue of the nested intervals principle. Theorem 6.1.1 (Nested d -cells principle) Let d ∈ N , and let I k be a nested decreasing sequence of closed d -cells (so I 1 ⊇ I 2 ⊇ I 3 ⊇ . . . ). Then there exists at least one point x ∈ R d such that x is in all of the d -cells I k , i.e., such that x ∈ � ∞ k =1 I k . Moreover, if diam ( I k ) → 0 as k → ∞ then this point x is unique. The statement and applications of this this d -dimensional principle are examinable, but the proof is an NEB exercise . It is, in fact, fairly easy to deduce the result from the nested intervals principle in R . 3
6.2 The Bolzano–Weierstrass Theorem Let x 1 , x 2 , x 3 , x 4 , x 5 , . . . be a sequence in R d . We can form a new sequence by omitting some of the sequence members, e.g. (i) x 1 , x 3 , x 5 , x 7 , . . . (ii) x 2 , x 4 , x 6 , x 8 , . . . (iii) x 2 , x 32 , x 532 , x 7532 , . . . (All three examples are meant to continue indefinitely.) In (i) we have formed the new sequence ( y n ) , where y n = x 2 n − 1 . In (ii) we have formed ( z n ) , where z n = x 2 n . In (iii) it is perhaps less clear what the pattern is but in any case we have chosen a strictly increasing sequence of positive integers k 1 = 2 < k 2 = 32 < k 3 = 532 < k 4 = 7532 < . . . and formed a new sequence ( p n ) given by p n = x k n . 4
Definition 6.2.1 A subsequence of a sequence ( x n ) in R d is a sequence of the form ( y n ) = ( x k n ) , where k 1 < k 2 < k 3 < . . . is an increasing sequence of positive integers. This means : when a subsequence is formed the order of the sequence members is kept and repetitions of the index are excluded. There may be repeated values , but only if there were repeated values in the original sequence. Why is this notion important? It happens often that divergent (i.e. non-convergent) sequences have convergent subsequences. For example, x n = ( − 1) n defines a divergent sequence in R but ( x 2 n ) is convergent. What about the sequence x n = sin( n ) in R ? (Here we are working in radians, as usual.) The Bolzano–Weierstrass Theorem (below) will show, in particular, that this sequence also has at least one convergent subsequence. 5
We say that a sequence ( x n ) in R d is bounded if the set { x n | n ∈ N } ⊆ R d of all sequence members is bounded, i.e. there exists M > 0 such that, for all n ∈ N , we have || x n || ≤ M . Now we can formulate the famous Theorem 6.2.2 (Bolzano–Weierstrass Theorem) Every bounded sequence ( x n ) in R d has at least one convergent subsequence. [ So, in particular, (sin( n )) ⊆ R has a convergent subsequence!] The statement of the Bolzano–Weierstrass Theorem and its applications are examinable. The proof is NEB . See books for full details, if interested. Most books give a fairly straightforward proof based on the nested d -cells principle, or some similar result. 6
6.3 Sequential compactness The notion of sequential compactness and other related notions of compactness are extremely important in analysis and mathematics in general and have far-reaching applications. Definition 6.3.1 A subset E ⊆ R d is said to be sequentially compact if every sequence ( x n ) ⊆ E has at least one subsequence which converges to a point of E . We can characterize sequentially compact subsets of R d as follows. Theorem 6.3.2 (Heine-Borel Theorem, sequential compactness version) Let E ⊆ R d be any subset. Then the following conditions are equivalent: (i) E is sequentially compact; (ii) E is closed and bounded. Gap to fill in 7
Gap to fill in 8
Gap to fill in 9
Gap to fill in 10
Gap to fill in 11
Example 6.3.3 1. With the traditional terminology, closed intervals in R and closed d -cells and closed balls in R d are sequentially compact. 2. The set { 0 } ∪ { 1 n | n ∈ N } is sequentially compact. Gap to fill in 12
Sequentially compact sets are ‘nice’ sets in many respects, as we will see. It also turns out that, for subsets of R d , sequential compactness is equivalent to a topological condition involving coverings by open sets. This related condition, which is called simply compactness , is of great importance throughout mathematics. See books and/or the module G13MTS if interested. 13
Recommend
More recommend