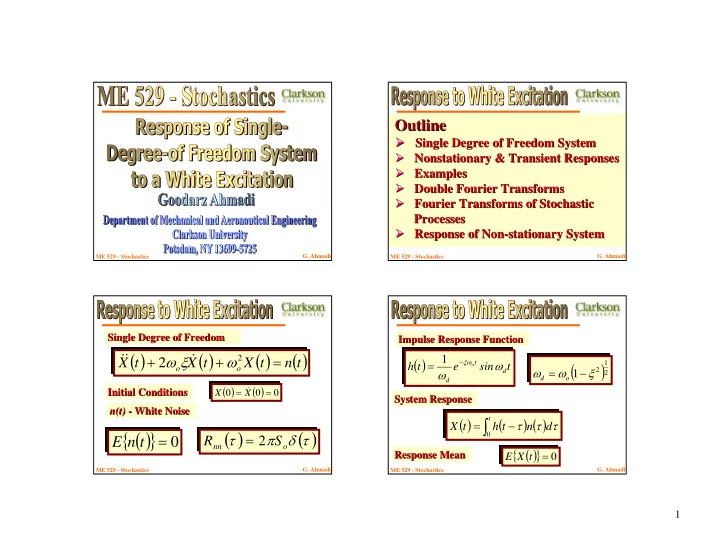
t x t h t n d 0 r 2 s e
play

t = X t h t n d { ( ) } ( ) ( ) = = - PowerPoint PPT Presentation

Mar 07, 2024 •312 likes •351 views

Outline Outline Single Degree of Freedom System Single Degree of Freedom System Nonstationary Nonstationary & Transient Responses & Transient Responses Examples Examples Double Fourier Transforms

  1. Outline Outline � � Single Degree of Freedom System Single Degree of Freedom System � Nonstationary � Nonstationary & Transient Responses & Transient Responses � Examples � Examples � Double Fourier Transforms � Double Fourier Transforms � Fourier Transforms of Stochastic � Fourier Transforms of Stochastic Processes Processes � � Response of Non Response of Non- -stationary System stationary System ME 529 - Stochastics G. Ahmadi ME 529 - Stochastics G. Ahmadi Single Degree of Freedom Single Degree of Freedom Impulse Response Function Impulse Response Function ( ) ( ) ( ) ( ) & & & + ω ξ + ω = ( ) 2 = 1 X t 2 X t X t n t − ξω ω ( ) 2 t 1 h t e sin t o o o ω = ω 1 ξ − 2 ω d d o d ( ) ( ) & = X = Initial Conditions Initial Conditions X 0 0 0 System Response System Response n(t) ) - - White Noise White Noise n(t ( ) ( ) ( ) ∫ t = − τ τ τ X t h t n d { ( ) } ( ) ( ) = τ = π δ τ 0 R 2 S E n t 0 nn o { ( ) } = Response Mean Response Mean E X t 0 ME 529 - Stochastics G. Ahmadi ME 529 - Stochastics G. Ahmadi 1

  2. Mean- -Square Response Square Response Mean- -Square Response Square Response Mean Mean { } ( ) ( ) ( ) ( ) ∫ ∫ t t ( ) ( ) ( ) 1 ∞ ( ) ( ) = − τ − τ τ − τ τ τ ∫ ∫ t t ∫ σ = − τ − τ ω ω τ − τ ω τ τ 2 E X t h t h t R d d 2 t h t h t S cos d d d 1 2 nn 1 2 1 2 x 1 2 π nn 1 2 1 2 0 0 0 0 0 For white excitation, integrating over delta function For white excitation, integrating over delta function Approximate Solution for Small Damping, Caughey Approximate Solution for Small Damping, Caughey and and Stumpf Stumpf (1963) (1963) { } π ( ) ( ) ( ) 2 S ∫ t = π τ τ ∫ t σ = − ξω τ ω τ τ 2 2 2 2 2 E X t 2 S h d o t e sin d ( ) o [ ] ⎧ ⎫ ω o x ω d 2 ( ) S 1 ( ) 0 0 σ ≈ − − ξω ω + ξω ω + ω ω ξ ω 2 ⎨ 2 t 2 2 2 ⎬ nn o d t 1 e o 2 sin t sin 2 t x ξω ω d o d o d d 3 2 4 ⎩ ⎭ o d [ ] ⎧ ⎫ π ( ) 2 S 1 ( ) − ξω . . σ = − ω + ξω ω + ω ω ξ ω 2 ⎨ 2 t 2 2 2 ⎬ o t 1 e o 2 sin t sin 2 t ( ) [ ξω ω ω x 3 2 d o d o d d ⎩ ⎭ ] 2 ( ) S For ξ ξ = 0 σ = ω − ω = 0 o d For 2 nn o t 2 t sin 2 t ω x 2 o o 4 o ME 529 - Stochastics G. Ahmadi ME 529 - Stochastics G. Ahmadi ME 529 - Stochastics G. Ahmadi ME 529 - Stochastics G. Ahmadi 2

  3. ( ) +∞ ( ) ( ) ( ) +∞ +∞ ( ) Linear System ∫ ( ) Linear System ∫ ∫ = − τ τ τ Γ ω ω = − ω − ω Y t X t h d i t t , R t , t e dt dt 1 1 2 2 − ∞ 1 2 1 2 1 2 − ∞ − ∞ = ∫ ( ) +∞ ( ) ( ) ( ) ( ) η η − τ τ τ = η Expected Value Expected Value t t h d t * h t ( ) 1 + ∞ + ∞ ( ) ( ) Y X X ∫ ∫ − ∞ = Γ ω ω ω − ω ω ω i t t Inverse Inverse R t , t , e d d 1 1 2 2 ( ) 1 2 π 1 2 1 2 2 − ∞ − ∞ 2 Autocorrelation Autocorrelation ( ) ( ) ( ) ( ) Γ ω ω = Γ ω ω Γ − ω − ω = Γ ω ω Properties * = ∫ Properties , , * ( ) +∞ ( ) ( ) ( ) ( ) , , − τ τ τ = 1 2 2 1 1 2 1 2 R t , t R t , t h d R t , t * h t XY 1 2 XX 1 2 XX 1 2 2 − ∞ Linear System Response Linear System Response = ∫ ( ) +∞ ( ) ( ) ( ) ( ) − τ τ τ = R t , t R t , t h d R t , t * h t ( ) ( ) ( ) ( ) YY 1 2 XY 1 2 XY 1 2 1 − ∞ . . Γ ω ω = Γ ω ω ω ω * , , H H ( ) ( ) ( ) ( ) YY 1 2 XX 1 2 1 2 = R t , t R t , t * h t * h t ( ) ( ) ( ) YY 1 2 XX 1 2 2 1 X(t) stationary X(t ) stationary Γ ω ω = π ω δ ω − ω , 2 S 1 2 1 2 1 ME 529 - Stochastics G. Ahmadi ME 529 - Stochastics G. Ahmadi ( ) +∞ ( ) +∞ ( ) For random input, X(t X(t) ) ∫ ∫ For random input, ω = ω − i ω 2 < ∞ Exists iff iff t Exists X X e dt X t dt − ∞ − ∞ ( ) ( ) ( ) ( ) ( ) ( ) = ω = ω ω Y t h t * X t Y H X ∴ FT of stationary processes does not exist ∴ FT of stationary processes does not exist ( ) + ∞ ( ) 1 ∫ ω d Inverse Inverse = ω ω i t Noting Noting X t X e ( ) ( ) ( ) } π − ∞ * 2 Γ ω ω = ω ω { , E Y Y YY 1 2 1 2 { } Mean of Mean of ( ) +∞ { ( ) } +∞ ( ) ∫ ∫ ω = − ω = η − ω i t i t E X E X t e dt t e dt X( ω ω ) X( ) X − ∞ − ∞ . ( ) ( . ) ( ) ( ) { } Γ ω ω = ω ω Γ ω ω ( ) ( ) ( ) * , H H , ω ω = Γ ω , ω * Theorem Theorem E X X YY 1 2 1 2 XX 1 2 1 2 1 2 ME 529 - Stochastics G. Ahmadi ME 529 - Stochastics G. Ahmadi 3

Recommend

More recommend