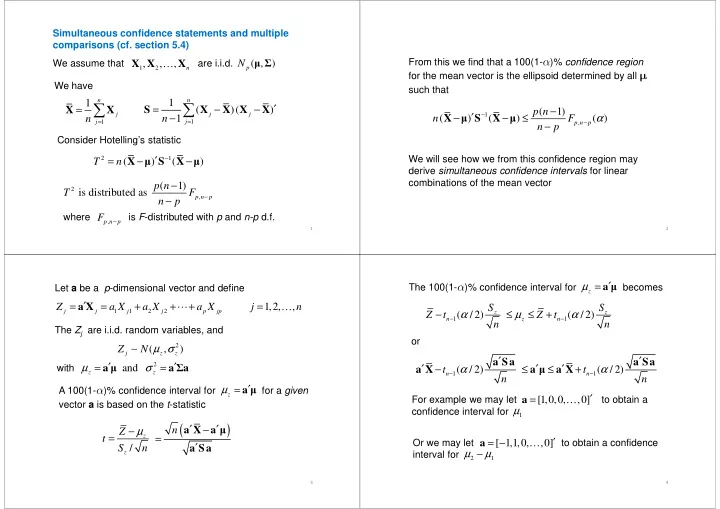
s x x x x x x 1 p n j 1 j j x s
play

= S ( X X )( X X ) X X = ( 1) p n j 1 j - PowerPoint PPT Presentation

Jul 05, 2023 •280 likes •330 views

Simultaneous confidence statements and multiple comparisons (cf. section 5.4) From this we find that a 100(1- )% confidence region , , , ( , ) X X X N We assume that are i.i.d. 1 2 n p for the

  1. Simultaneous confidence statements and multiple comparisons (cf. section 5.4) From this we find that a 100(1- α )% confidence region , , , ( , ) X X X N … � Σ We assume that are i.i.d. 1 2 n p for the mean vector is the ellipsoid determined by all � We have such that 1 1 n n = ∑ − ∑ S ( X X )( X X ) ′ X X = − − ( 1) p n − j 1 j j ( X � S ) ′ − 1 ( X ) ( ) n − − ≤ F α n n � 1 = 1 j = j , − p n p n n − − p p Consider Hotelling’s statistic 2 = ( X − � S ) ′ − 1 ( X − ) T n � We will see how we from this confidence region may derive simultaneous confidence intervals for linear ( − 1) p n combinations of the mean vector 2 is distributed as T F , − p n p n − p where is F -distributed with p and n-p d.f. F , p n p − 1 2 = a � ′ The 100(1- α )% confidence interval for becomes µ Let a be a p -dimensional vector and define z ′ = a X = + + + = 1,2, , Z a X a X a X j n ⋯ … S S j j 1 j 1 2 j 2 p jp − ( α / 2) ≤ µ ≤ + ( α / 2) Z t z Z t z − 1 − 1 n z n n n The Z j are i.i.d. random variables, and or N µ σ ( , 2 ) Z ∼ j z z a Sa ′ a Sa ′ a � ′ and 2 a Σ a ′ µ = σ = a X ′ − ( α / 2) ≤ a � ′ ≤ a X ′ + ( α / 2) t t with z z − 1 − 1 n n n n n n = a � ′ A 100(1- α )% confidence interval for for a given µ z a [1,0,0, ,0] ′ = … For example we may let to obtain a vector a is based on the t -statistic µ confidence interval for 1 ( ) a X ′ − a � ′ n − µ Z = t z = a [ 1,1,0, ,0] ′ = − … Or we may let to obtain a confidence / a Sa ′ S n µ − µ z interval for 2 1 3 4

  2. For a given vector a the confidence interval given above is [1,0,0, ,0] ′ a = For we obtain the standard t -interval: … a � ′ the set of values for which s s ( ) a X ′ a � ′ n − ( / 2) 11 ( / 2) 11 X − t α ≤ µ ≤ X + t α 1 ( / 2) 1 − 1 1 1 − 1 = ≤ α n n t t n n n − ′ a Sa For illustration we will consider the t- intervals for the or equivalently , , , p means for the particular case where X X X … 1 2 n ( ( ( ( a X a X ′ ′ )) )) 2 − − n n � � ( , ( , σ σ 2 2 ) ) N N 2 2 2 2 ( ( / 2) / 2) � � Ι Ι = = ≤ ≤ α α t t t t are i.i.d. are i.i.d. p 1 a Sa ′ n − Then We will derive confidence intervals that hold (all -intervals contain the s) µ P t simultaneously for all possible choices of a with overall i confidence coefficient 100(1- α )% = (1 − α ) (1 ⋅ − α ) ⋅ ⋅ (1 − α ) = (1 − α ) p ⋯ 2 1 ( α / 2) t We then have to replace by a larger value − This will be (much) smaller than 1- α n 5 6 a [1,0,0, ,0] ′ We are then lead to the determination of = … In particular for one obtains the µ following confidence interval for (similarly for the ( ( a X ′ )) 2 max n − � ( ( a X ′ )) 2 1 − � max 2 = t max = n other means) a Sa ′ a Sa ′ a a a   ( − 1) s ( − 1) s p n p n − ( ) α 11 , + ( ) α 11  X F X F  Using the maximization lemma we obtain 1 , − 1 , − − p n p − p n p n p n p n n     max 2 ( X � S ) ′ − 1 ( X ) t = n − − � a S S − − 1 ( 1 ( X X ) ) For p =2 the simultaneous For p =2 the simultaneous with the maximum occurring for a proportional to − − � � confidence intervals for It follows that simultaneously for all a , the interval the means are the projections of the   a Sa ′ a Sa ′ ( − 1) ( − 1) p n p n confidence ellipse a X ′ − ( ) α , a X ′ + ( ) α F F   , − , − − p n p − p n p n p n p n n     (a similar results holds in higher dimensions) ′ will contain with probability 1- α a � 7 8

  3. If we are only interested in a fixed number of linear ′ ′ ′ a � a � , , , a � Note that while one in the “one-at-a-time” confidence … combinations we may use the 1 2 m ′ 1 ( / 2) a Sa / α n t intervals multiplies by one for n − Bonferroni method the simultaneous confidence intervals has to multiply by ′ ( − 1) a � p n For we then use the modified t- interval ( ) α F i , − − p n p n p   a Sa ′ a Sa ′ a X a X ′ ′ − − ( ( α α / 2) / 2) i i i i , , a X a X ′ ′ + + ( ( α α / 2) / 2) i i i i t t t t     − 1 − 1 i n i i n i n n     Ratio for p = 2, 5 and 10: a � ′ Let C i denote the confidence statement about i ( is true) = − 1 α P C Then i i 9 10 In particular the Bonferroni confidence intervals for the We obtain p means become (all are true) P C i   s s  α   α  , X − t ii X + t ii       1 (at least one is false) = − P C i n − 1 2 i n − 1 2 p n p n         i 1 ({ is false} { is false} { is false}) = − ∪ ∪ ∪ P C C ⋯ C 1 2 m m ≥ − ∑ ≥ − ∑ 1 1 ( ( is false) is false) P C P C i i = 1 i 1 ( ) = − α + α + ⋯ α 1 2 m α = α / m If we let we obtain i (all are true) ≥ − 1 α P C i 11 12

  4. Note that while the simultaneous confidence intervals ( 1) p n − a Sa ′ / ( ) α n F multiplies by one for , p n p − n − p 1 ( α / 2 ) t m the Bonferroni intervals multiplies by n − Ratio for p = 2, 5 and 10 (when m = p ): 13

Recommend

More recommend