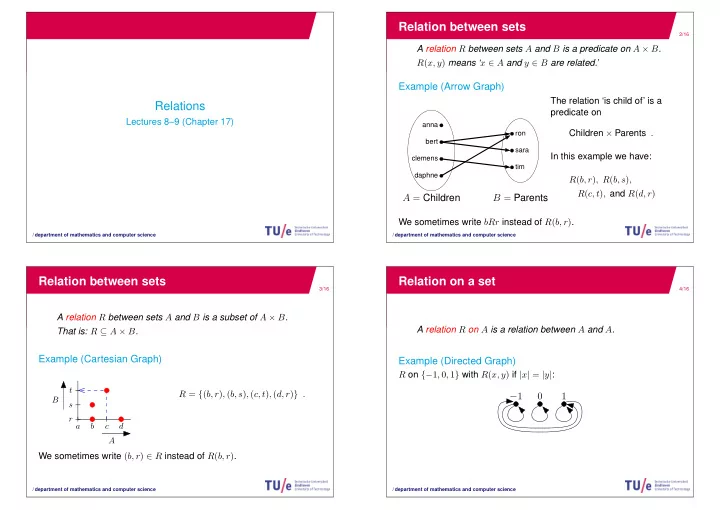
Relation between sets 2/16 A relation R between sets A and B is a - PowerPoint PPT Presentation

Relation between sets 2/16 A relation R between sets A and B is a predicate on A × B . R ( x, y ) means ‘ x ∈ A and y ∈ B are related.’ Example (Arrow Graph) The relation ‘is child of’ is a Relations predicate on Lectures 8–9 (Chapter 17) anna Children × Parents . ron bert sara In this example we have: clemens tim daphne R ( b, r ) , R ( b, s ) , R ( c, t ) , and R ( d, r ) A = Children B = Parents We sometimes write bRr instead of R ( b, r ) . / department of mathematics and computer science / department of mathematics and computer science Relation between sets Relation on a set 3/16 4/16 A relation R between sets A and B is a subset of A × B . A relation R on A is a relation between A and A . That is: R ⊆ A × B . Example (Cartesian Graph) Example (Directed Graph) R on {− 1 , 0 , 1 } with R ( x, y ) if | x | = | y | : t R = { ( b, r ) , ( b, s ) , ( c, t ) , ( d, r ) } . − 1 0 1 B s r a b c d A We sometimes write ( b, r ) ∈ R instead of R ( b, r ) . / department of mathematics and computer science / department of mathematics and computer science

Reflexive relations Symmetric relations 5/16 6/16 A relation R on A is symmetric if if A relation R on A is reflexive if ∀ x,y [ x, y ∈ A : xRy ⇒ yRx ] . x y then ∀ x [ x ∈ A : xRx ] . x for all x, y ∈ A . for all x ∈ A . Symmetric: Not symmetric: val val Reflexive: Not reflexive: ◮ | = = on abstr. props = = on abstr. props ◮ val val ◮ > on R = = , | = = on abstr. props ◮ ◮ = on R ◮ > , ≥ on R ◮ = , ≥ on R ◮ R on N with xRy if x = 3 y ◮ ‘ is married ’ on people ◮ ‘ is child of ’ on people (Counterexample: ¬ (1 R 1) .) ◮ . . . ◮ R on N with xRy if x = 3 y ◮ . . . ◮ . . . (Counterexample: 6 R 2 , but ¬ (2 R 6) ) ◮ . . . / department of mathematics and computer science / department of mathematics and computer science Transitive relations Equivalence relation 7/16 8/16 R on A is an equivalence relation if and only if if 1. R is reflexive, and A relation R on A is transitive if 2. R is symmetric, and x y z ∀ x,y,z [ x, y, z ∈ A : ( xRy ∧ yRz ) ⇒ xRz ] . then 3. R is transitive. for all x, y, z ∈ A . Examples Transitive: Not transitive: val = = on abstr. props ◮ val val ◮ ‘is sister of’ on people = = , | = = on abstr. props ◮ = on N , Z , R , C , . . . ◮ ∃ k [ k ∈ Z : x − y = k · 6] ◮ = on sets ◮ = , > , ≥ on R ◮ R on N with xRy if x = 3 y ◮ the relation ≡ 6 on Z defined by (Counterexample: 18 R 6 and ◮ . . . 6 R 2 , but ¬ (18 R 2) ) x ≡ 6 y if x − y is a multiple of 6 ◮ . . . (e.g., 10 ≡ 6 4 , 61 ≡ 6 1 , − 2 ≡ 6 10 , 9 ≡ 6 9 ) / department of mathematics and computer science / department of mathematics and computer science

≡ 6 on Z ≡ 6 is transitive: 10/16 11/16 Define the binary relation ≡ 6 on Z by var x, y, z ; x, y, z ∈ Z x ≡ 6 y if ∃ k [ k ∈ Z : x − y = 6 · k ] . x ≡ 6 y ∧ y ≡ 6 z Fact ∃ k [ k ∈ Z : x − y = 6 · k ] ( ∧ -elim + definition ≡ 6 ) ≡ 6 is an equivalence relation on Z . Pick a k with k ∈ Z and x − y = 6 · k ( ∃ ∗ -elim) ∃ ℓ [ ℓ ∈ Z : y − z = 6 · ℓ ] ( ∧ -elim + definition ≡ 6 ) Proof: We need to prove that Pick an ℓ with ℓ ∈ Z and y − z = 6 · ℓ ( ∃ ∗ -elim) x − z = ( x − y ) + ( y − z ) = 6 · k + 6 · ℓ = 6 · ( k + ℓ ) (Mathematics) 1. ≡ 6 is reflexive; x − z = 6 · ( k + ℓ ) (Mathematics) [Proof: see book.] k + ℓ ∈ Z (since k ∈ Z and ℓ ∈ Z ) 2. ≡ 6 is symmetric; ∃ m [ m ∈ Z : x − z = 6 · m ] ( ∃ ∗ -intro) [Proof: see book.] x ≡ 6 z (definition ≡ 6 ) 3. ≡ 6 is transitive. ∀ x,y,z [ x, y, z ∈ Z : x ≡ 6 y ∧ y ≡ 6 z ⇒ x ≡ 6 z ] [Proof on next slide; see also book] Note: It is important to choose k and ℓ distinct in this proof. / department of mathematics and computer science / department of mathematics and computer science ≡ 6 is transitive: Equivalence classes 12/16 13/16 Let A be a set, let R be an equivalence relation on A . Proof: To prove that x ≡ 6 y and y ≡ 6 z implies x ≡ 6 z for all x, y, z ∈ Z , let K ( a ) def The equivalence class of = { x ∈ A | aRx } x, y, z ∈ Z and suppose that x ≡ 6 y and y ≡ 6 z ; we need to establish a ∈ A is the set of all end- that x ≡ 6 z . points of arrows from a . From x ≡ 6 y and y ≡ 6 z it follows, by the definition of ≡ 6 , that there exists k and ℓ with x − y = 6 · k and y − z = 6 · ℓ . Since R is an equivalence relation, b K ( a ) a c Hence, everything within a class is related. d For instance: b ′ x − z = ( x − y ) + ( y − z ) = 6 · k + 6 · ℓ = 6 · ( k + ℓ ) . K ( a ′ ) a ′ c ′ d ′ � symm. aRd dRa trans. Since k, ℓ ∈ Z , we also have that ( k + ℓ ) ∈ Z . � � dRc aRc So we have that x − z is a multiple of 6 , and hence, according to the Note: definition of ≡ 6 , it follows that x ≡ 6 z . K(a)=K(b)=K(c)=K(d) / department of mathematics and computer science / department of mathematics and computer science

Equivalence classes Equivalence classes 14/16 15/16 Example Consider the equivalence relation ≡ 5 on Z . Example Let R be an equivalence relation on a set A , and let a, a ′ ∈ A . Prove that K (0) 0 Then, e.g., ¬ ( aRa ′ ) ⇒ K ( a ) ∩ K ( a ′ ) = ∅ K (1) 1 K (2) 2 K (3) = { . . . , − 7 , − 2 , 3 , 8 , 13 , . . . } . K (3) [Proof on next slide] 3 Note: K (4) = K (9) = K (14) = · · · 4 K (4) / department of mathematics and computer science / department of mathematics and computer science Example: ¬ ( aRa ′ ) ⇒ K ( a ) ∩ K ( a ′ ) = ∅ 16/16 (1) ¬ ( a R a ′ ) (2) var x ; x ∈ K ( a ) ∩ K ( a ′ ) (3) x ∈ K ( a ) ∧ x ∈ K ( a ′ ) (4) a R x a ′ R x (5) (6) x R a ′ ( ∀ -elim on ‘ R is symmetric’ followed by ⇒ -elim) (7) a R a ′ ( ∀ -elim on ‘ R is transitive’ followed by ∧ -intro and ⇒ -elim) (8) False (9) K ( a ) ∩ K ( a ′ ) = ∅ ¬ ( aRa ′ ) ⇒ K ( a ) ∩ K ( a ′ ) = ∅ (10) / department of mathematics and computer science