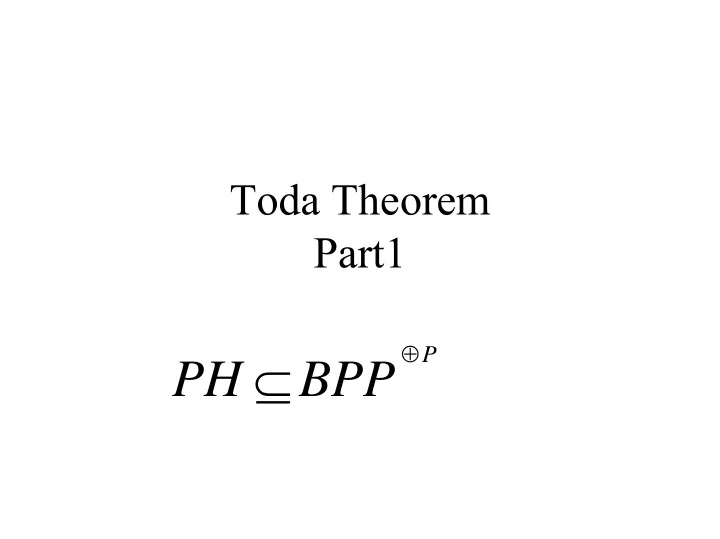

Toda Theorem Part1 ⊕ PH ⊆ P BPP
Basic Definitions • Language L belongs to class PP if exists polynomial NTM such that ∈ ⇔ = > { ( ) 1 } 0 . 5 x L P M x • Language L belongs to class BPP if exists probabilistic polynomial algorithm such that x from L is accepted with probability more than 0.75 and x not form L is declined with the same probability.
⊕ Class P • Language L belongs to class ⊕ (parity P) if exists P polynomial-time non-deterministic Turing machine, such x lies in L is equivalent to that fact, that number of accepting computations is odd(or, exists polynomially checked relation R such that the number of y(R(x, y)= 1 ) is odd. ⊕ •It can be quickly checked that is complete for SAT ⊕ P
Polynomial hierarchy Σ = 0 P P + Σ Ρ Σ = i i 1 P NP U = Σ i - Polynomial Hierarchy PH P ≥ 0 i
General Idea of proof ⊕ ⊕ ⊆ ⊆ P P NP BPP NP RP A ⊕ ⊆ A P NP BPP • because because of Valiant-Vazirani Lemma Σ ⊆ ⊕ ∀ i ∈ i P N P BPP ⊆ ⊕ P PH BPP • because Valiant-Vazirani Lemma can be relativised •We will prove, that by the mathematical induction. It will prove, that •Base i =1 is obvious
Idea of Proof A A • Lemma 2: ⊕ ⊕ ⊆ BPP P P BPP • Lemma 3: ⊕ ⊕ ⊆ ⊕ P P P A • Lemma 4: ⊆ BPP A BPP BPP So, considering this Lemmas being truth we’ll receive ⊕ P ⊕ ⊕ ⊕ ⊕ P P P P BPP Σ + = Σ ⊆ ⊆ ⊕ ⊆ ⊆ ⊆ ⊕ 1 k k P BPP P BPP BPP P P NP NP BPP BPP BPP BPP and the first part of Toda’s Theorem will be proved.
Lemma 4 − • In definition of BPP we can use, instead of (1-3/4), 2 p i where is a polynomially function of input length p i • M uses L as oracle, M is wrong with pr= − ( ) L ∈ e n A 2 BPP • We can consider, that the lengths of all branches of NTM L are equal and the number of its accesses to L is equal to l(n) in all branches • We can replace every access to oracle L by the branch of machine N(from the definition of BPP) with the probability of mistake − ( ) i n 2 • The number of branches, which status will change is equal to − ( ) 2 e n − − + − − ( ) ( ) ( ) that can be made less than 0.25 2 1 ( 1 2 ) e n i n l n
Lemma 2 A ∈ ⊕ B ∈ • so exists NTM with oracle accepting BPP A A L P BPP γ ∈ ∈ ( ) { 0 , 1 } { 0 , 1 } n n every for odd number of y. x y R is corresponding relationship A A A ∈ ∈ ∈ ∈ B BPP BPP A R P P BPP BPP • So = ∈ Π ≥ − − π ς ( ) ( ) { |# { :# { : ( , , ) ( )} ( 1 2 ) 2 } } A n n L x y z x y z L is odd • And we need: ς = ∈ Π ≥ ( n ) { |# { :# { : ( , , ) ( )} } 0 . 75 * 2 } A L x z y x y z L is odd
Lemma 2- end • We can consider a table, (for fixed x, WLOG x is in L), in (y, z) we’ll put result of П for given (y, z).The number of rows with more than − π ς − ( ) ( ) ( 1 2 ) 2 } n n ones is odd and the number of columns, which have 1 in intersection with this columns is more than ς − π ς γ − ( ) ( ) ( ) ( ) 2 2 * 2 * 2 n n n n • In other rows is not many 1,so the number of ‘good’ rows is ς − π ς γ − π ς γ ς − − ≥ ( ) ( ) ( ) ( ) ( ) ( ) ( ) ( ) 2 2 * 2 * 2 2 * 2 * 2 0 . 75 * 2 n n n n n n n n for π ≥ γ + ( ) ( ) 3 n n
P ⊕ Lemma 5 − co = P ⊕
Recommend
More recommend