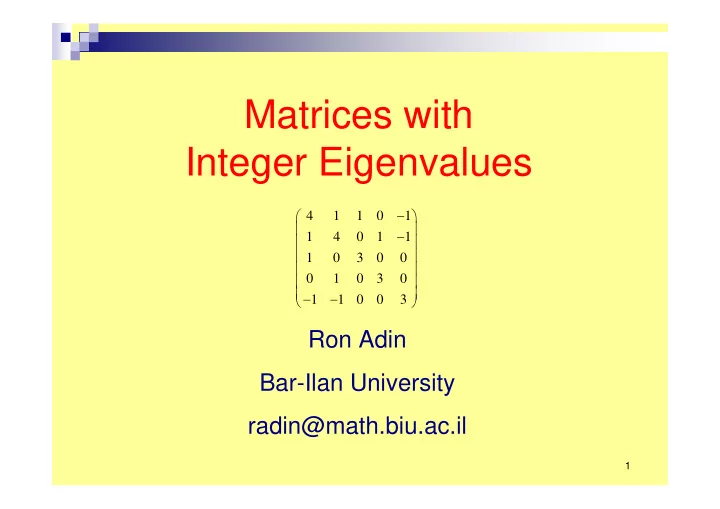
Matrices with Integer Eigenvalues 4 1 1 0 1 1 4 0 1 - PowerPoint PPT Presentation

Matrices with Integer Eigenvalues 4 1 1 0 1 −   1 4 0 1 1   −   1 0 3 0 0    0 1 0 3 0    1 1 0 0 3   − −   Ron Adin Bar-Ilan University radin@math.biu.ac.il 1

A Recent Monthly Paper Almost All Integer Matrices Have No Integer Eigenvalues , G. Martin and E. B. Wong, Amer. Math. Monthly 116 (2009), 588-597. We want to deal with (specific) exceptions. 2

Highlights � Certain matrices with integer entries have, conjecturally, integer eigenvalues only. � Partial results are known. � The multiplicity of the zero eigenvalue has an algebraic interpretation. 3

A Signed Graph: Vertices [0, ]: {0,1, , } r r � = … 0 0 , 1 k ≥ a b k � For and let ≤ ≤ + ( , ) : k a b ( , ) U V set of all pairs s.t. Ω = , [0, ], , . U V k U a V b ⊆ = = ( , ) : wt U V u v � Weight: ∑ ∑ = + u U v V ∈ ∈ ( , , ) : ( , ) ( , ) k a b w U V a b set of all Ω = ∈Ω k � . w with weight 4

A Signed Graph: Vertices (cont.) 2, 4. a b w � Example: = = = 0,1: (2,2,4) k = Ω = ∅ k � 2: (2,2,4) {(12,01),(02,02),(01,12)} k = Ω = � k 3: (2,2,4) {(03,01),(12,01),(02,02), k = Ω = � k (01,12),(01,03)} a b a b         , ( ) w w k a b � Note: = + = + − − min max 2 2 2 2                 5

A Signed Graph: Edges ( , ),( , ) ( , , ) U V U V a b w ɶ ɶ � For ∈Ω k , , , u U v V z and write ∈ ℤ ∈ ∈ ( , ) ( , ) U V U V ɶ ɶ if ∼ ( , , ) u v z ( \{ }) { } U U u u z ɶ 1. = ∪ + ( \{ }) { } V V v v z ɶ 2. = ∪ − u v k 3. + ≤ 6

A Signed Graph: Edges ( , ),( , ) ( , , ) U V U V a b w ɶ ɶ � For ∈Ω k , , , u U v V z and write ∈ ℤ ∈ ∈ ( , ) ( , ) U V U V ɶ ɶ if ∼ ( , , ) u v z U u v V ( \{ }) { } U U u u z ɶ 1. u v = ∪ + + ( \{ }) { } V V v v z ɶ 2. = ∪ − U u z v z V ɶ ɶ u v k 3. + − + ≤ 7

A Signed Graph ( , , ) ( , , ) G a b w = k a b w graph with vertex set k Ω � and edges corresponding to the various ( , ) ( , ). U V U V ɶ ɶ ∼ ( , , ) u v z � Loops and multiple edges may occur. � Attach signs to edges: 8

Signs ( , , ), ( , , ) u u u u u u ɶ ɶ ɶ � For define: … … 1 1 a a = = ( , ) 0 u u u u if or has repeated elements; ɶ ɶ ε = ( , ) ( ) ( ) u u sign sign ɶ if ε σ τ = , . u u u u ɶ ɶ … … (1) ( ) (1) ( ) a a < < < < σ σ τ τ { , , }, { , , , , } U u u U u u z u ɶ � For … … … 1 1 a i a = = + u u where , define 1 a < < … ( , ) : (( , , ),( , , , , )). U U u u u u z u ɶ … … … 1 1 a i a = + ε ε (( , ),( , ) ) : ( , ) ( , ) U V U V U U V V ɶ ɶ ɶ ɶ � ε ε ε = ⋅ 9

Signed Adjacency Matrix ( , , ) T a b w = the (signed) adjacency matrix k � ( , , ). G a b w of the graph k Note: � Diagonal elements are nonnegative integers. 0, 1 1. Off-diagonal elements are or − 10

Signed Adjacency Matrix 3, 2, 3, 8 k a b w � Example: = = = = 4 1 1 0 1 −   1 4 0 1 1   −   ( , , ) 1 0 3 0 0 T a b w   = k   0 1 0 3 0   1 1 0 0 3   − −   11

Conjectures � Conjecture: [Hanlon, ’92] ( , , ) T a b w All the eigenvalues of are k nonnegative integers. 12

Conjectures (cont.) r ( , , ) : ( , , ) a b M x y m a b r x y λ � Let = ∑ λ k k , , a b r ( , , ) m a b r = r where multiplicity of as an e.v. k ( , ) ( , , ) . T a b T a b w of = ⊕ k k w � Conjecture: [Hanlon, ’92] k ( , , ) 1 1 i M x y x y xy λ + ( ) λ ∏ k = + + + 0 i = Still open (in general)! 13

Background � Macdonald’s root system conjecture 14

Background � Macdonald’s root system conjecture � Hanlon’s property M conjecture: ( [ ]/( 1 )) ( ) ( 1) k k H L t t H L ℂ + ⊗ + * ⊗ ≅ * L where the Lie algebra is either * semisimple, or * upper triangular (nilpotent), or * Heisenberg (nilpotent) 15

Background � Macdonald’s root system conjecture � Hanlon’s property M conjecture: ( [ ]/( 1 )) ( ) ( 1) k k H L t t H L ℂ + ⊗ + * * ⊗ ≅ L where the Lie algebra is either * semisimple, or * upper triangular (nilpotent), or * Heisenberg (nilpotent) 16

Background � Macdonald’s root system conjecture TRUE [Cherednik, ’95] � Hanlon’s property M conjecture: ( [ ]/( 1 )) ( ) ( 1) k k H L t t H L ℂ + ⊗ + * * ⊗ ≅ L where the Lie algebra is either * semisimple, or * upper triangular (nilpotent), or * Heisenberg (nilpotent) 17

Background � Macdonald’s root system conjecture TRUE [Cherednik, ’95] � Hanlon’s property M conjecture: ( [ ]/( 1 )) ( ) ( 1) k k H L t t H L ℂ + ⊗ + * * ⊗ ≅ L where the Lie algebra is either * semisimple, or TRUE [FGT, ’08] * upper triangular (nilpotent), or * Heisenberg (nilpotent) 18

Background � Macdonald’s root system conjecture TRUE [Cherednik, ’95] � Hanlon’s property M conjecture: ( [ ]/( 1 )) ( ) ( 1) k k H L t t H L ℂ + ⊗ + * * ⊗ ≅ L where the Lie algebra is either * semisimple, or TRUE [FGT, ’08] * upper triangular (nilpotent), or FALSE [Kumar, ’99] * Heisenberg (nilpotent) 19

Background � Macdonald’s root system conjecture TRUE [Cherednik, ’95] � Hanlon’s property M conjecture: ( [ ]/( 1 )) ( ) ( 1) k k H L t t H L ℂ + ⊗ + * * ⊗ ≅ L where the Lie algebra is either * semisimple, or TRUE [FGT, ’08] * upper triangular (nilpotent), or FALSE [Kumar, ’99] * Heisenberg (nilpotent) ? 20

Background (cont.) 0 * *     0 0 * ,   L   � For the 3-dim Heisenberg =  3  { , , },   e f x with basis 0 0 0     * *     the Laplacian ∂∂ + ∂ ∂ [ ]/( 1 ) k L t t + for ⊗ ℂ 3 ( , , ) a b w T a b w is (in a suitable basis). ⊕ k , , � The property M conjecture is then equivalent to (an extension of) ( , ,0) (1 ) k 1 M x y x y + k = + + 21

Partial Results � [Hanlon, ’92] Explicit eigenvalues in the stable case: a b * ≤ a b     w ≤ + * 2 2         a b     ( 1) ( 1) k a b w ≥ − + − + − − * 2 2         22

Partial Results (cont.) � Theorem: [Hanlon, ’92] 1:1 ( , ) ( , ) k a b In the stable case, pairs Ω ↔ λ µ min . w w of partitions with λ µ + = − . ( , ) ab T a b The eigenvalues of are λ µ k − + The proof uses Schur functions in two sets of variables. 23

Partial Results (cont.) � [A.-Athanasiadis, ’96] 1, 2, : a b k 1. The nonzero eigenvalues = = ∀ 1, , 1, ( , , ) k T a b w of are ⊕ λ = w k + … k each with multiplicity (and explicit w distribution over ). 1, , : a b k 2. The multiplicity of the zero e.v.: = ∀ 1 k k +     (1, ,0) ( 1) m b k = = + k 1  b k b    b −     24

Partial Results (cont.) � [Hanlon-Wachs, ’02] , , : a b k Extend result 2 above to ∀ 1 k +   ( , ,0) m a b =  k 1 a b k a b  + − −   25

Partial Results (cont.) � [Kuflik, ’06] ( , , ) : w a b k Distribution over ∀ ( , , ,0) w w m a b w q min coefficient of in − k = 1 k +   1 a b k a b   + − −   q where [ ]! : [1] [2] [ ] , m m ⋯ q q q q = ⋅ [ ] : 1 1 . m m q q − … q = + + + 26

הבשקהה לע הדות ! 27