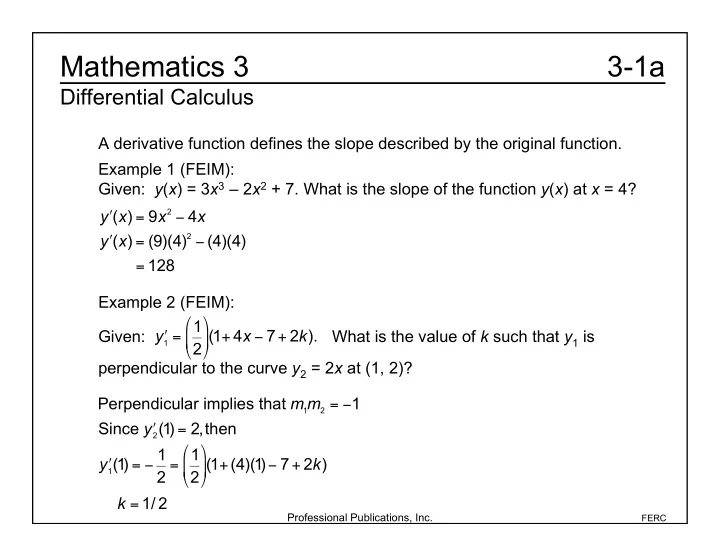
Mathematics 3 3-1a Differential Calculus A derivative function - PowerPoint PPT Presentation

Mathematics 3 3-1a Differential Calculus A derivative function defines the slope described by the original function. Example 1 (FEIM): Given: y ( x ) = 3 x 3 – 2 x 2 + 7. What is the slope of the function y ( x ) at x = 4? 2 � 4 x y ( x ) = 9 x � 2 � (4)(4) y ( x ) = (9)(4) � = 128 Example 2 (FEIM): 1 = 1 � � y (1 + 4 x � 7 + 2 k ). Given: What is the value of k such that y 1 is � � � 2 � � perpendicular to the curve y 2 = 2 x at (1, 2)? Perpendicular implies that m 1 m 2 = � 1 Since � y 2 (1 ) = 2,then ) = � 1 2 = 1 � � y 1 (1 (1 + (4)(1 ) � 7 + 2 k ) � � � 2 � � k = 1/ 2 Professional Publications, Inc. FERC

Mathematics 3 3-1b Differential Calculus Maxima Minima Example (FEIM): (maxima) What is the maximum of the function y = – x 3 + 3 x for x ≥ –1? 2 + 3 y = � 3 x � y = � 6 x � � 2 + 3 When � y = 0 = � 3 x 2 = 1 x ; x = ± 1 y (1 ) = � 6 < 0; therefore, this is a maximum. � � y ( � 1 ) = 6 > 0; therefore, this is a minimum. � � 3 + 3 = 2 y (1 ) = � (1 ) Professional Publications, Inc. FERC

Mathematics 3 3-1c Differential Calculus Inflection Point f ′′ ( a ) changes sign about x = a Example (FEIM): What is the point of inflection of the function y = – x 3 + 3 x – 2? 2 + 3 y = � 3 x � y = � 6 x � � y = 0 when x = 0 and � y > 0 for x < 0; � y < 0 for x > 0 � � � � Therefore this is an inflection point. 3 + (3)(0) � 2 = � 2 y (0) = � (0) Professional Publications, Inc. FERC

Mathematics 3 3-1d Differential Calculus Partial Derivative • A derivative taken with respect to only one independent variable at a time. Example (FEIM): What is the partial derivative of P ( R , S , T ) taken with respect to T ? 1/ 2 + R P = 2 R 3 S 2 T 3 / 4 S cos2 T P = 2 R 3 S 2 ( T 1/ 2 ) + R 3 / 4 S (cos2 T ) � P � T = 2 R 3 S 2 ( 1 2 T � 1/ 2 ) + R 3 / 4 S ( � 2sin2 T ) � 1/ 2 � 2 R = R 3 S 2 T 3 / 4 S sin2 T Professional Publications, Inc. FERC

Mathematics 3 3-1e Differential Calculus Curvature Radius of Curvature Example (FEIM): What is the curvature of y = – x 3 + 3 x for x = –1? (A) –2 (B) –1 (C) 0 (D) 6 2 + 3 � y = � 3 x y = � 6 x � � y ( � 1 ) = 0 � y ( � 1 ) = 6 � � y 6 � � K = 3 / 2 = 6 3 / 2 = (1 + ( � y ) 2 ) (1 + (0) 2 ) Therefore, (D) is correct. Professional Publications, Inc. FERC

Mathematics 3 3-1f Differential Calculus Limits Look at what the function does as it approaches the limit. If the limit goes to plus or minus infinity: • look for constants that become irrelevant • look for functions that blow up fast: a factorial, an exponential If the limit goes to a finite number: • look at what happens at both plus and minus a small number � � � � f ( x ) , f ( a ) � = 0 0 or = � For lim � : � � � g ( x ) g ( a ) x � a � � � � • Use L’Hôpital’s rule NOTE: Use L’Hôpital’s rule only when the next derivative of f ( x ) and g ( x ) exist. Professional Publications, Inc. FERC

Mathematics 3 3-1g1 Differential Calculus Example 1 (FEIM): � � x + 4 What is the value of lim ? � � x � 4 x �� � � (A) 0 (B) 1 (C) ∞ (D) undefined Divide the numerator and denominator by x . � � 1 + 4 � � � � x + 4 = 1 + 0 x lim � = lim 1 � 0 = 1 � � � x � 4 1 � 4 x �� x �� � � � � x � � Therefore, (B) is correct. Professional Publications, Inc. FERC

Mathematics 3 3-1g2 Differential Calculus Example 2 (FEIM): 2 � 4 � x � What is the value of lim ? � � x � 2 x � 2 � � (A) 0 (B) 2 (C) 4 (D) ∞ Factor out an ( x – 2) term in the numerator. 2 � 4 � � � � x ( x � 2)( x + 2) lim � = lim � = lim x � 2 ( x + 2) = 2 + 2 = 4 � � x � 2 x � 2 x � 2 x � 2 � � � � Therefore, (C) is correct. Professional Publications, Inc. FERC

Mathematics 3 3-1h Differential Calculus Example 3 (FEIM): � � 1 � cos x What is the value of lim ? � � x 2 x � 0 � � (A) 0 (B) 1/4 (C) 1/2 (D) ∞ Both the numerator and denominator approach 0, so use L’Hôpital’s rule. � � � � 1 � cos x sin x lim � = lim � � � x 2 2 x x � 0 x � 0 � � � � Both the numerator and denominator are still approaching 0, so use L’Hôpital’s rule again. � � � � sin x cos x � = cos(0) lim � = lim = 1/ 2 � � 2 x 2 2 x � 0 x � 0 � � � � Therefore, (C) is correct. Professional Publications, Inc. FERC

Mathematics 3 3-2a Integral Calculus Constant of Integration • added to the integral to recognize a possible term Example (FEIM): 2 x + 2 x ) dx if y = 1 when x = 1? y ( x ) = ( e What is the constant of integration for � (A) 2 � e 2 (B) � 1 2 e 2 (C) 4 � e 2 (D) 1 + 2 e 2 2 x + x 2 + C y ( x ) = 1 2 e 2 + 1 + C = 1 y (1 ) = 1 2 e C = � 1 2 e 2 Therefore, (B) is correct. Professional Publications, Inc. FERC

Mathematics 3 3-2b Integral Calculus Indefinite Integrals 1. Look for ways to simplify the formula with algebra before integrating. 2. Plug in initial value(s). 3. Solve for constant(s). 4. Indefinite integrals can be solved by differentiating the answers, but this is usually the hard way. Professional Publications, Inc. FERC

Mathematics 3 3-2c Integral Calculus Method of Integration – Integration by Parts Example (FEIM): Find x 2 e x dx . � Let g ( x ) = e x and f ( x ) = x 2 x � so dg ( x ) = e x dx x 2 e x dx = x 2 e 2 xe x dx � � ax dx = e ax xe 2 ( ax � 1 ) . From the NCEES Handbook: � a x � 2( xe x � e Therefore, x 2 e x dx = x 2 e x ) + C � Notice that chosing dg ( x ) = x 2 dx and f ( x ) = e x does not improve the integral. Professional Publications, Inc. FERC

Mathematics 3 3-2d Integral Calculus Method of Integration – Integration by Substitution • Trigonometric Substitutions: Example (FEIM): x + 2 x ) x + 2) dx Find ( e 2 ( e . � x + 2 x Let u ( x ) = e x + 2) dx so, du = ( e 2 du = u 3 + C = 1 3 x + 2 x ) x + 2) dx x + 2 x ) 3 + C ( e 2 ( e u 3( e � � = Professional Publications, Inc. FERC

Mathematics 3 3-2e Integral Calculus Method of Integration – Partial Fractions • Transforms a proper polynomial fraction of two polynomials into a sum of simpler expressions Example 1 (FEIM): 2 + 9 x � 3 6 x Find dx , using the partial fraction expression. � x ( x + 3)( x � 1 ) 2 + 9 x � 3 6 x ) = A x + 3 + C B x � 1 = A ( x + 3)( x � 1 ) B ( x )( x � 1 ) ) + C ( x )( x + 3) x + ) + x ( x + 3)( x � 1 x ( x + 3)( x � 1 x ( x + 3)( x � 1 x ( x + 3)( x � 1 ) So, 6 x 2 + 9 x – 3 = A ( x + 3)( x –1) + B ( x )( x – 1) + C ( x )( x + 3) Solve using the three simultaneous equations: A + B + C = 6 2 A – B + 3 C = 9 –3 A = –3 A = 1, B = 2, and C = 3 2 + 9 x � 3 6 x 1 2 3 ) dx = x dx + x � 1 dx = In x + 2In x + 3 + 3In x � 1 + C � � � � x + 3 + x ( x + 3)( x � 1 Professional Publications, Inc. FERC

Mathematics 3 3-2f Integral Calculus If the denominator has repeated roots, then the partial fraction expansion will have all the powers of that root. Example 2 (FEIM): 4 x � 9 2 . Find the partial fraction expansion of ( x � 3) 4 x � 9 A B A ( x � 3) B 2 = x � 3 + 2 = x � 3( x � 3) + ( x � 3) ( x � 3) ( x � 3) 2 4 x – 9 = Ax – 3 A + B Solve using the two simultaneous equations. A = 4 –9 = –3 A + B A = 4 and B = 3 4 x � 9 4 3 Therefore, 2 = x � 3 + ( x � 3) ( x � 3) 2 Professional Publications, Inc. FERC

Mathematics 3 3-2g Integral Calculus Definite Integrals 1. Solve the indefinite integral (without the constant of integration). 2. Evaluate at upper and lower bounds. 3. Subtract lower bound value from upper bound value. Example (FEIM): Find the integral between π /3 and π /4 of f ( x ) = cos x. � � 3 cos xdx = � cos � 3 � � 4 4 � � = � cos � 3 � � cos � � � 4 � � = � 0.5 + 0.707 = 0.207 Professional Publications, Inc. FERC

Mathematics 3 3-2h Integral Calculus Average Value 1 b Average = f ( x ) dx � b � a a Example (FEIM): What is the average value of y ( x ) = 2 x + 4 between x = 0 and x = 4? 4 � � � � 1 (2 x + 4) dx = 1 � 2 x 2 1 2 + (4)(4) 4 Average = 4 4 ( ) = 8 � = � � � 4 � 0 4 2 0 � � � � 0 Professional Publications, Inc. FERC

Mathematics 3 3-2i Integral Calculus Area Problems y f 1 ( x ) b area = ( f 1 ( x ) � f 2 ( x )) dx � a f 2 ( x ) a b x Example (FEIM): What is the area between y 1 = (1/4) x + 3 and y 2 = 6 x – 1 between x = 0 and x = 1/2? � � � � � � 1 � 23 1 1 Area = 4 x + 3 � � (6 x � 1 ) dx = 4 x + 4 dx � � 2 2 � � � � � 0 0 � � � � � � 1 2 � � � � � � = � 23 = � 23 � 1 + 4 2 = 41 2 2 + 4 x 8 x � � � � � 8 2 32 � � � � � � 0 Professional Publications, Inc. FERC

Mathematics 3 3-2j Integral Calculus Centroid First Moment of Area Moment of Inertia Professional Publications, Inc. FERC

Mathematics 3 3-3a Differential Equations First-Order Homogeneous Equations General form: General solution: Initial condition: usually y ( b ) = constant or y ′ ( b ) = constant C = y ( b ) y ( b ) � � ab or C = e e � ab Professional Publications, Inc. FERC