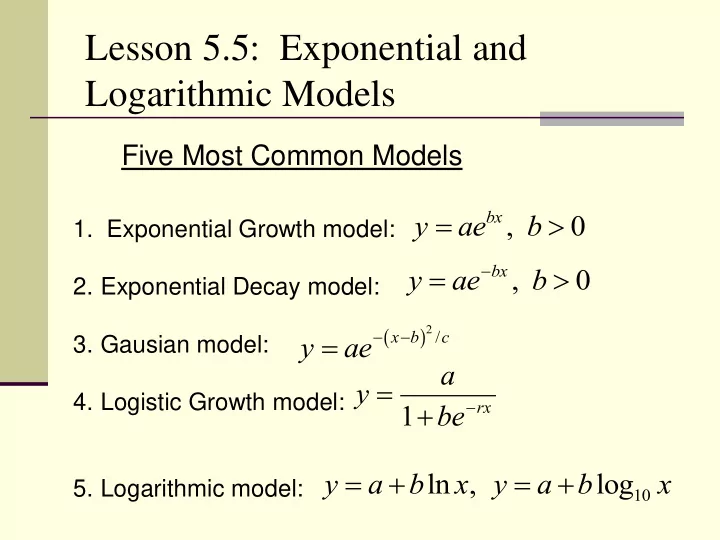
Lesson 5.5: Exponential and Logarithmic Models Five Most Common - PowerPoint PPT Presentation

Lesson 5.5: Exponential and Logarithmic Models Five Most Common Models   bx y ae , b 0 1. Exponential Growth model:  ,   bx y ae b 0 2. Exponential Decay model: b g 2 /    x b c y ae 3. Gausian model: a  y 4. Logistic Growth model:   be rx 1     y a b ln , x y a b log 10 x 5. Logarithmic model:

Ex 1: Exponential Growth In a research experiment, a population of fruit flies is increasing according to the law of exponential growth. After 2 days there are 100 flies, and after 4 days, there are 300 flies. How many flies are there after 5 days?  ae bx y x = time, y = # of flies

 ae bx y x = time, y = # of flies a  100  ae b  33 0 549 2 100 . x y e  100 3 a b g  33 0 549 5 .  33 e b 2 y e  e b 2 ln ln 3  ae b  514 4 300 y flies  F I 100 b  ln 2 3 H K 4 b 300 e 2 b e b  ln3  100 2 e b 300 2  e b 2 3  0549 .

Ex 2: Exponential Decay In living organic material, the ratio of the number of radioactive carbon isotopes (carbon-14) to the non- radioactive carbon isotopes (carbon-12) is about 1 to 10 12 . When organic material dies, its carbon-12 content remains fixed while the carbon-14 begins to decay with a half-life of 5700 years. To estimate the age of dead organic material, scientists us the following formula, which denotes the ratio of carbon-14 to carbon-12 present at 1 any time t (in years)   e t / 8223 R 10 12 Find the age of a newly discovered fossil if the ratio of 1 carbon-14 to carbon-12 is 10 13

Ex 2: Continued. Half-life of Carbon-14 = 5715 years. How long until only 25% of fossil is remaining? b g   0 00012 . x . 25 C Ce  k  ln .5 ae kx y   0 00012 . x 5715 . 25 e  5715 k b g c h .5 C Ce   000012 .   0 00012 . x ln . 25 ln e  e 5715 k .5 b g   000012 . x ln . 25 b g c h b g  5715 k ln . 5 ln e ln . 25 x   b g 000012 . k  ln . 5715 5  11552 x , years

Ex 3: Logarithmic Model On the Richter Scale, the magnitude, R, of an earthquake intensity, I, is I  log 10 R I 0 where I 0 is the minimum intensity used for comparison. Find the intensities per unit of area for the following earthquakes. (Intensity is a measure of the wave energy of an earthquake.) A. Tokyo and Yokohama, Japan 1923: R = 8.3 B. El Salvador, 2001: R = 7.7

Ex 3: Continued. I I   7 7 . log 8 3 . log 199 526 2315 , , . 10 10 1 1 50118 7234 , , .  3981 .  83 . log I  7 7 . log I 10 10 Conclusion: The  7 7 . log I  log I 8 3 . 10 10 10 10 10 1923 earthquake 10 had an intensity I  10 7 7 . I  10 8 3 approximately . four times I  50118 7234 I  199 526 2315 , , . greater than the , , . 2001 earthquake Homework: p.416-417 #37, 39, 42, 51, 52