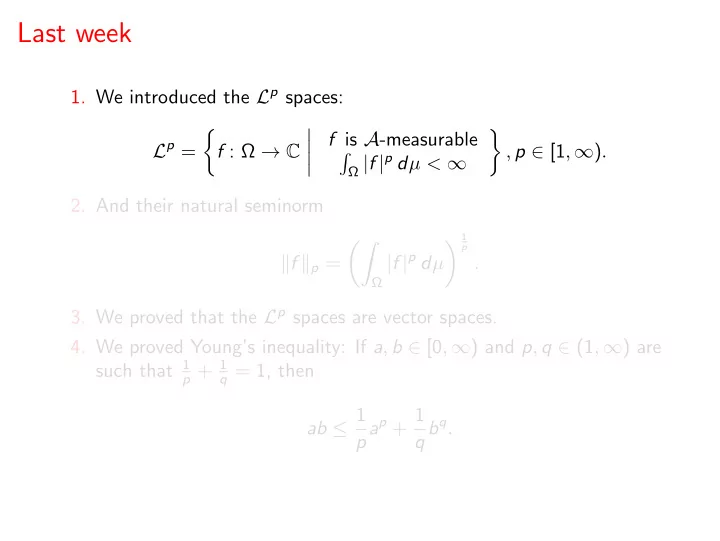

Last week 1. We introduced the L p spaces: � � � f is A -measurable L p = � f : Ω → C , p ∈ [1 , ∞ ) . Ω | f | p d µ < ∞ � � � 2. And their natural seminorm � 1 �� p | f | p d µ � f � p = . Ω 3. We proved that the L p spaces are vector spaces. 4. We proved Young’s inequality: If a , b ∈ [0 , ∞ ) and p , q ∈ (1 , ∞ ) are such that 1 p + 1 q = 1, then ab ≤ 1 p a p + 1 q b q .
Last week 1. We introduced the L p spaces: � � � f is A -measurable L p = � f : Ω → C , p ∈ [1 , ∞ ) . Ω | f | p d µ < ∞ � � � 2. And their natural seminorm � 1 �� p | f | p d µ � f � p = . Ω 3. We proved that the L p spaces are vector spaces. 4. We proved Young’s inequality: If a , b ∈ [0 , ∞ ) and p , q ∈ (1 , ∞ ) are such that 1 p + 1 q = 1, then ab ≤ 1 p a p + 1 q b q .
Last week 1. We introduced the L p spaces: � � � f is A -measurable L p = � f : Ω → C , p ∈ [1 , ∞ ) . Ω | f | p d µ < ∞ � � � 2. And their natural seminorm � 1 �� p | f | p d µ � f � p = . Ω 3. We proved that the L p spaces are vector spaces. 4. We proved Young’s inequality: If a , b ∈ [0 , ∞ ) and p , q ∈ (1 , ∞ ) are such that 1 p + 1 q = 1, then ab ≤ 1 p a p + 1 q b q .
Last week 1. We introduced the L p spaces: � � � f is A -measurable L p = � f : Ω → C , p ∈ [1 , ∞ ) . Ω | f | p d µ < ∞ � � � 2. And their natural seminorm � 1 �� p | f | p d µ � f � p = . Ω 3. We proved that the L p spaces are vector spaces. 4. We proved Young’s inequality: If a , b ∈ [0 , ∞ ) and p , q ∈ (1 , ∞ ) are such that 1 p + 1 q = 1, then ab ≤ 1 p a p + 1 q b q .
Today 1. We will prove H¨ older’s Inequality: If f , g are A -measurable and p , q ∈ (1 , ∞ ) are conjugate exponents ( 1 p + 1 q = 1), then � | fg | d µ = � f � p � g � q . Ω 2. We will prove Minkowski’s Inequality: If p ∈ [1 , ∞ ) and f , g ∈ L p , then � f + g � p ≤ � f � p + � g � p . 3. We will discuss how to make L p into a normed space called L p . 4. We will prove that the L p spaces are Banach spaces. 5. We will discuss the space of essentially bounded functions L ∞ .
Today 1. We will prove H¨ older’s Inequality: If f , g are A -measurable and p , q ∈ (1 , ∞ ) are conjugate exponents ( 1 p + 1 q = 1), then � | fg | d µ = � f � p � g � q . Ω 2. We will prove Minkowski’s Inequality: If p ∈ [1 , ∞ ) and f , g ∈ L p , then � f + g � p ≤ � f � p + � g � p . 3. We will discuss how to make L p into a normed space called L p . 4. We will prove that the L p spaces are Banach spaces. 5. We will discuss the space of essentially bounded functions L ∞ .
Today 1. We will prove H¨ older’s Inequality: If f , g are A -measurable and p , q ∈ (1 , ∞ ) are conjugate exponents ( 1 p + 1 q = 1), then � | fg | d µ = � f � p � g � q . Ω 2. We will prove Minkowski’s Inequality: If p ∈ [1 , ∞ ) and f , g ∈ L p , then � f + g � p ≤ � f � p + � g � p . 3. We will discuss how to make L p into a normed space called L p . 4. We will prove that the L p spaces are Banach spaces. 5. We will discuss the space of essentially bounded functions L ∞ .
Today 1. We will prove H¨ older’s Inequality: If f , g are A -measurable and p , q ∈ (1 , ∞ ) are conjugate exponents ( 1 p + 1 q = 1), then � | fg | d µ = � f � p � g � q . Ω 2. We will prove Minkowski’s Inequality: If p ∈ [1 , ∞ ) and f , g ∈ L p , then � f + g � p ≤ � f � p + � g � p . 3. We will discuss how to make L p into a normed space called L p . 4. We will prove that the L p spaces are Banach spaces. 5. We will discuss the space of essentially bounded functions L ∞ .
Today 1. We will prove H¨ older’s Inequality: If f , g are A -measurable and p , q ∈ (1 , ∞ ) are conjugate exponents ( 1 p + 1 q = 1), then � | fg | d µ = � f � p � g � q . Ω 2. We will prove Minkowski’s Inequality: If p ∈ [1 , ∞ ) and f , g ∈ L p , then � f + g � p ≤ � f � p + � g � p . 3. We will discuss how to make L p into a normed space called L p . 4. We will prove that the L p spaces are Banach spaces. 5. We will discuss the space of essentially bounded functions L ∞ .
Recommend
More recommend