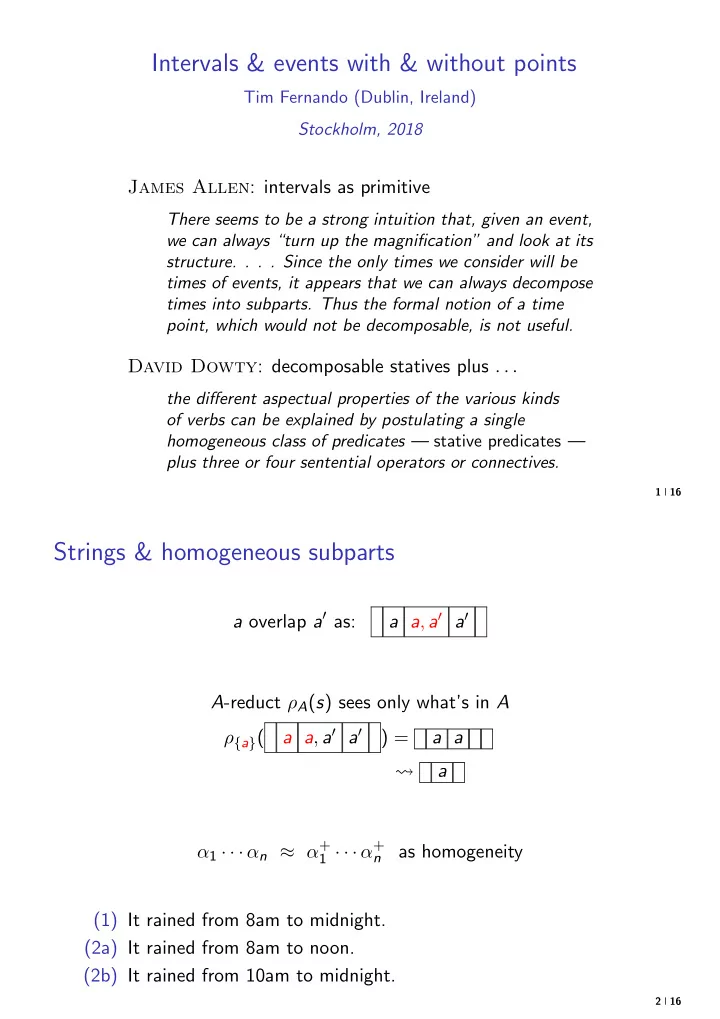
Intervals & events with & without points Tim Fernando - PDF document

Intervals & events with & without points Tim Fernando (Dublin, Ireland) Stockholm, 2018 James Allen : intervals as primitive There seems to be a strong intuition that, given an event, we can always “turn up the magnification” and look at its structure. . . . Since the only times we consider will be times of events, it appears that we can always decompose times into subparts. Thus the formal notion of a time point, which would not be decomposable, is not useful. David Dowty : decomposable statives plus . . . the different aspectual properties of the various kinds of verbs can be explained by postulating a single homogeneous class of predicates — stative predicates — plus three or four sentential operators or connectives. 1 16 Strings & homogeneous subparts a overlap a ′ as: a a , a ′ a ′ A -reduct ρ A ( s ) sees only what’s in A a a , a ′ a ′ ρ { a } ( ) = a a a � α 1 · · · α n ≈ α + 1 · · · α + as homogeneity n (1) It rained from 8am to midnight. (2a) It rained from 8am to noon. (2b) It rained from 10am to midnight. 2 16

Compression two ways & projection s αα s ′ � s α s ′ α 1 · · · α n is stutterless if α i � = α i +1 for 1 ≤ i < n c − 1 α 1 · · · α n = α + 1 · · · α + for stutterless α 1 · · · α n b n s � s ′ � ss ′ α 1 · · · α n is depadded if α i � = � for 1 ≤ i ≤ n � α 1 · · · α n = � ∗ α 1 � ∗ · · · � ∗ α n � ∗ d − 1 for depadded α 1 · · · α n s projects to s ′ if d � ( ρ voc ( s ′ ) ( s )) = s ′ , where voc ( α 1 · · · α n ) := α 1 ∪ · · · ∪ α n 3 16 Points & intervals via a transduction a is an s-point if s projects to a — i.e., d � ( ρ { a } ( s )) = a s | = ( ∃ x )( ∀ y )( P a ( y ) ≡ x = y ) a is an s-interval if b ( s ) projects to l ( a ) r ( a ) s | =( ∃ x )( ∃ y )( x < y ∧ ( ∀ z )( P a ( z ) ≡ x < z ∧ z ≤ y )) b : (2 A ) ∗ → (2 A • ) ∗ , α 1 · · · α n �→ β 1 · · · β n A • := { l ( a ) | a ∈ A } ∪ { r ( a ) | a ∈ A } β n := { r ( a ) | a ∈ α n } β i := { l ( a ) | a ∈ α i +1 − α i } ∪ { r ( a ) | a ∈ α i − α i +1 } for i < n 4 16

Outline § 1 Allen interval relations - 13 strings - composition via superposition (constrained) § 2 Events under inertia & force § 3 MSO variations 5 16 Allen relations projected = aRa ′ b ( s ) projects to s R ( a , a ′ ) s | ⇐ ⇒ R − 1 aRa ′ s R ( a , a ′ ) s R − 1 ( a , a ′ ) R a before a ′ l ( a ) r ( a ) l ( a ′ ) r ( a ′ ) l ( a ′ ) r ( a ′ ) l ( a ) r ( a ) < > m a meets a ′ l ( a ) r ( a ) , l ( a ′ ) r ( a ′ ) mi l ( a ′ ) r ( a ′ ) , l ( a ) r ( a ) o a overlaps a ′ l ( a ) l ( a ′ ) r ( a ) r ( a ′ ) oi l ( a ′ ) l ( a ) r ( a ′ ) r ( a ) s a starts a ′ l ( a ) , l ( a ′ ) r ( a ) r ( a ′ ) si l ( a ) , l ( a ′ ) r ( a ′ ) r ( a ) d a during a ′ l ( a ′ ) l ( a ) r ( a ) r ( a ′ ) di l ( a ) l ( a ′ ) r ( a ′ ) r ( a ) f a finishes a ′ l ( a ′ ) l ( a ) r ( a ) , r ( a ′ ) fi l ( a ) l ( a ′ ) r ( a ) , r ( a ′ ) = a equal a ′ l ( a ) , l ( a ′ ) r ( a ) , r ( a ′ ) = Each s R ( a , a ′ ) projects to l ( a ) r ( a ) and l ( a ′ ) r ( a ′ ) 6 16

From 2 intervals to 3 a ′ < a ′′ a ′ d a ′′ a < a ′ a o a ′ a < a ′′ a { d,o,s } a ′′ · · · < o d < d m o s · · · < < < · · · o < < m o d o s d < d m o s d · · · < . . . . . . . . . . . . · · · s < ( a , a ′ ) & s < ( a ′ , a ′′ ) = l ( a ′ ) r ( a ′ ) l ( a ′′ ) r ( a ′′ ) l ( a ) r ( a ) s o ( a , a ′ ) & s d ( a ′ , a ′′ ) = a d a ′′ l ( a ′′ ) l ( a ) l ( a ′ ) r ( a ) r ( a ′ ) r ( a ′′ ) a o a ′′ l ( a ) l ( a ′′ ) l ( a ′ ) r ( a ) r ( a ′ ) r ( a ′′ ) + a s a ′′ l ( a ) , l ( a ′′ ) l ( a ′ ) r ( a ) r ( a ′ ) r ( a ′′ ) + 7 16 Superposition l ( a ′ ) r ( a ′ ) , s ) s = s R ( a , a ′ ) for some R &( l ( a ) r ( a ) , ⇐ ⇒ &( s , s ′ , s ′′ ) &( s , s ′ , s ′′ ) &( s , s ′ , s ′′ ) &( α s , α ′ s , ( α ∪ α ′ ) s ′′ ) &( α s , s ′ , α s ′′ ) &( s , α ′ s ′ , α ′ s ′′ ) &( ǫ, ǫ, ǫ ) Constrain through Σ , Σ ′ α ∩ Σ ′ ⊆ α ′ α ′ ∩ Σ ⊆ α & Σ , Σ ′ ( s , s ′ , s ′′ ) & Σ , Σ ′ ( α s , α ′ s , ( α ∪ α ′ ) s ′′ ) α ′ ∩ Σ = ∅ α ∩ Σ ′ = ∅ & Σ , Σ ′ ( s , s ′ , s ′′ ) & Σ , Σ ′ ( s , s ′ , s ′′ ) & Σ , Σ ′ ( α s , s ′ , α s ′′ ) & Σ , Σ ′ ( s , α ′ s ′ , α ′ s ′′ ) ⇒ &( s , s ′ , s ′′ ) and s ′′ projects to s and s ′ & voc ( s ) , voc ( s ′ ) ( s , s ′ , s ′′ ) ⇐ 8 16

Outline § 1 Allen interval relations - 13 strings - composition via superposition (constrained) § 2 Events under inertia & force - inverting Dowty aspect hypothesis - forces beyond borders § 3 MSO variations 9 16 Borders & consequences P l ( a ) ( x ) ≡ ¬ P a ( x ) ∧ ( ∃ y )( xSy ∧ P a ( y )) P r ( a ) ( x ) ≡ P a ( x ) ∧ ¬ ( ∃ y )( xSy ∧ P a ( y )) so that s projects to a string from ( l ( a ) r ( a ) ) ∗ + r ( a ) ( l ( a ) r ( a ) ) ∗ Conversely, P a ( x ) ≡ ( ∃ X )( X ( x ) ∧ a -path( X ) � ) � �� ∀ x ( X ( x ) ⊃ P r ( a ) ( x ) ∨ ∃ y ( xSy ∧ X ( y )) ∧ ¬∃ x ( X ( x ) ∧ P l ( a ) ( x )) 10 16

Forces & inertia: l ( a ) , r ( a ) � f a , f a ¬ P a ( x ) ∧ ( ∃ y )( xSy ∧ P a ( y )) ⊃ P f a ( x ) P a ( x ) ∧ ( ∃ y )( xSy ∧ ¬ P a ( y )) ⊃ P f a ( x ) No change without force (inertia) Moens & Steedman 1988 atomic extended +conseq culmination culminated process ϕ ϕ ϕ ,ap( f ) ϕ ,ap( f ),ef( f ) ef( f ) , ϕ − conseq point process ap( f ) ef( f ) ap( f ) ap( f ),ef( f ) ef( f ) Effects of forces? 11 16 Competition & incrementality P f a ( x ) ∧ xSy ∧ ¬ P a ( x ) ⊃ P a ( y ) may fail because ◮ f a may co-occur with an opposing force ◮ f’s incremental effect falls short. Analyze P a as attribute-value pair ( A , v ) with 0 ≤ v ≤ 1 so that at v �∈ { 0 , 1 } , a force may raise the A -value and/or a force may lower the A -value — i.e., forces may compete, with effects �∈ { 0 , 1 } . 12 16

Outline § 1 Allen interval relations - 13 strings - composition via superposition (constrained) § 2 Events under inertia & force - inverting Dowty aspect hypothesis - forces beyond borders § 3 MSO variations - variable ontology (many-sorted) - reducts & truthmakers (institutions) 13 16 Leibniz’s law: identity of indiscernibles x � = y ⊃ ( ∃ P ) ¬ ( P ( x ) ≡ P ( y )) (LL) - take P from a finite set A � x �≡ A y := ¬ ( P a ( x ) ≡ P a ( y )) b c a ∈ A � ≡ ( ¬ P a ( x ) ∧ P a ( y )) ∨ ( P a ( x ) ∧ ¬ P a ( y )) a ∈ A P l ( a ) ( x ) P r ( a ) ( x ) d � - replace � = by adjacency S “time steps S only with change A ” xSy ⊃ x �≡ A y (LL A , S ) 14 16

Reducts & institutions s | = ϕ ⇐ ⇒ ρ voc ( ϕ ) ( s ) | = ϕ ( ϕ ∈ MSO A ) s projects to s ′ d � ( ρ voc ( s ′ ) ( s )) = s ′ ⇐ ⇒ s | = Σ σ [ ϕ ] ⇐ ⇒ s σ | = Σ ′ ϕ � σ [ · ] : Sen(Σ ′ ) → Sen(Σ) σ Σ ′ → Σ · σ : Mod(Σ) → Mod(Σ ′ ) Satisfaction condition ( Barwise, Goguen & Burstall ) ϕ as: = s ′ e.g. σ [ ϕ ] as: ( ρ voc ( s ′ ) ; d � ) − 1 s ′ (in MSO by B¨ uchi Elgot Trakh) 15 16 Events as truthmakers ( Davidson ) strings to the left & right of | = particular s | = universal ϕ (7) Amundsen flew to the North Pole in May 1926. ∃ x (Amundsen-flew-to-the-North-Pole(x) ∧ In(May1926,x)) “if (7) is true, then there is an event that makes it true” (D 67) s | s ∈ L ( ϕ ) s σ ∈ L σ ( ϕ ) = ϕ � � x � substring of α 1 · · · α n ρ A reduces α i to α i ∩ A “thin” d � may drop an entire α i “thick” 16 16