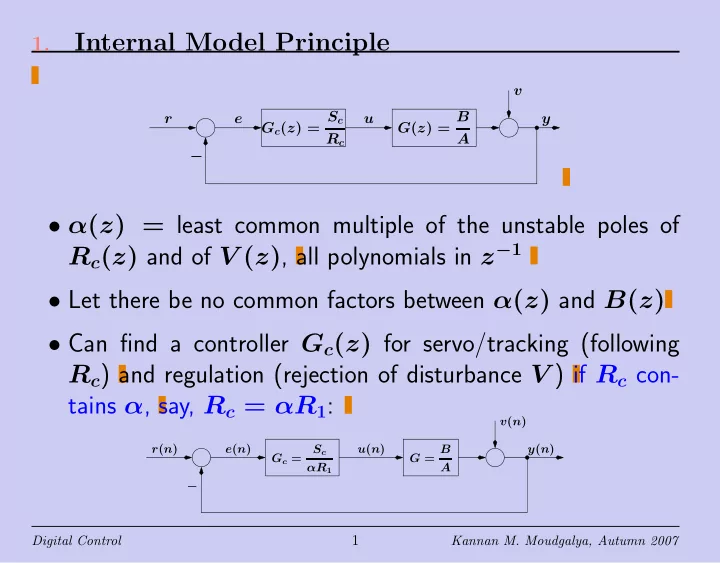
Internal Model Principle 1. v G c ( z ) = S c G ( z ) = B r e u - PowerPoint PPT Presentation

Internal Model Principle 1. v G c ( z ) = S c G ( z ) = B r e u y R c A − • α ( z ) = least common multiple of the unstable poles of R c ( z ) and of V ( z ) , all polynomials in z − 1 • Let there be no common factors between α ( z ) and B ( z ) • Can find a controller G c ( z ) for servo/tracking (following R c ) and regulation (rejection of disturbance V ) if R c con- tains α , say, R c = αR 1 : v ( n ) r ( n ) e ( n ) u ( n ) y ( n ) S c G = B G c = αR 1 A − 1 Digital Control Kannan M. Moudgalya, Autumn 2007

Internal Model Principle - Regulation 2. v ( n ) r ( n ) e ( n ) S c u ( n ) G = B y ( n ) G c = αR 1 A − S c 1 B 1 R 1 α A Y ( z ) = R + V 1 + S c 1 B 1 + S c 1 B R 1 α A R 1 α A S c B R 1 Aα b V = R 1 Aα + S c BR + R 1 Aα + S c B αa V • Unstable pole present in α gets cancelled • Regulation problem verified 2 Digital Control Kannan M. Moudgalya, Autumn 2007

Internal Model Principle - Servo 3. v ( n ) r ( n ) e ( n ) S c u ( n ) G = B y ( n ) G c = αR 1 A − S c B R 1 Aα Y ( z ) = R 1 Aα + S c BR + R 1 Aα + S c BV Servo problem: assume V = 0 : E ( z ) = R ( z ) − Y ( z ) S c B R 1 Aα b R � � = 1 − R = R 1 Aα + S c B R 1 Aα + S c B αa R • Unstable poles of R c are cancelled by zeros of α . • Can choose R c and S c such that R 1 Aα + S c B has roots within the unit circle (pole placement) • IM Principle: unstable poles of V , R c appear in loop thro’ α 3 Digital Control Kannan M. Moudgalya, Autumn 2007

Internal Stability 4. r ( n ) e ( n ) u ( n ) y ( n ) + g c ( n ) g ( n ) − • Notion in UG classes: output has to be stable • Output being stable is not sufficient • Every signal in the loop should be bounded • If any signal is unbounded, will result in saturation / overflow / explosion • When every signal is bounded, called internal stability • If output is stable and if there is no unstable pole-zero can- cellation, internal stability 4 Digital Control Kannan M. Moudgalya, Autumn 2007

Unstb. Pole-Zero Cancel. = No Int. Stability 5. r 2 + r 1 + e 1 G c = n 2 e 2 G = n 1 y + d 2 d 1 −  1 G  − � E 1 � � R 1 � 1 + GG c 1 + GG c   =  G c 1  E 2 R 2   1 + G c G 1 + G c G  d 1 d 2 n 1 d 2  − � R 1 � n 1 n 2 + d 1 d 2 n 1 n 2 + d 1 d 2   =  n 2 d 1 d 1 d 2  R 2   n 1 n 2 + d 1 d 2 n 1 n 2 + d 1 d 2 Suppose d 1 , n 2 have a common factor: d 1 = ( z + a ) d ′ 1 n 2 = ( z + a ) n ′ 2 5 Digital Control Kannan M. Moudgalya, Autumn 2007

Unstable Pole-Zero Cancellation = No Internal 6. Stability Assume the cancellation of z + a , G c ( z ) = ( z + a ) n ′ n 1 ( z ) 2 ( z ) G ( z ) = 1 ( z ) , ( z + a ) d ′ d 2 ( z ) with | a | > 1 . Assume stability of d ′ 1 1 d 2 T E = = d ′ 1 d 2 + n 1 n ′ 1 + GG c 2 T.F. between R 2 and Y can be shown to be unstable. Let R 1 = 0 . Y G n 1 d 2 = = ( d ′ 1 d 2 + n 1 n ′ R 2 1 + GG c 2 )( z + a ) It is unstable and a bounded signal injected at R 2 will produce an unbounded signal at Y 6 Digital Control Kannan M. Moudgalya, Autumn 2007

Forbid Unstable Pole-Zero Cancellation: Loop 7. Variable • Internal stability = all variables in loop are bounded for bounded external inputs at all locations • Can be checked by the following closed loop diagram r 2 + r 1 e 1 e 2 y G c = n 2 G = n 1 + + d 2 d 1 − • Can show that Output is stable + no pole-zero cancellation = internal stability 7 Digital Control Kannan M. Moudgalya, Autumn 2007

Forbid Unstable Pole-Zero Cancellation ⇒ Get 8. Causality Not possible to realize this controller: G c = 1 + z − 1 z − 1 All sampled systems have at least one delay: A ( z − 1 ) = z − k b 0 + b 1 z − 1 + b 2 z − 2 + · · · G ( z − 1 ) = z − k B ( z − 1 ) 1 + a 1 z − 1 + a 2 z − 2 + · · · • Controller not realizable ⇒ there is a common factor z − 1 between plant and controller • z − 1 = 0 ⇒ z = ∞ , an unstable pole • If unstable pole-zero cancellation is forbidden while designing controllers, z − 1 cannot appear in the denominator of the controller - i.e. , controller is realizable 8 Digital Control Kannan M. Moudgalya, Autumn 2007

Delay Specification for Realizability 9. Closed loop delay has to be ≥ open loop delay: A ( z ) = z − k b 0 + b 1 z − 1 + · · · G ( z ) = z − k B ( z ) 1 + a 1 z − 1 + · · · b 0 � = 0 . Suppose that we use a feedback controller of the form R c ( z ) = z − d s 0 + s 1 z − 1 + · · · G c ( z ) = z − d S c ( z ) 1 + r 1 z − 1 + · · · with s 0 � = 0 and d ≥ 0 . Closed loop transfer function: GG c T = 1 + GG c b 0 s 0 + ( b 0 s 1 + b 1 s 0 ) z − 1 + · · · = z − k − d 1 + ( s 1 + r 1 ) z − 1 + · · · + z − k − d ( b 0 s 0 + · · · ) 9 Digital Control Kannan M. Moudgalya, Autumn 2007

Delay Specification for Realizability 10. b 0 s 0 + ( b 0 s 1 + b 1 s 0 ) z − 1 + · · · T = z − k − d 1 + ( s 1 + r 1 ) z − 1 + · · · + z − k − d ( b 0 s 0 + · · · ) • Closed loop delay = k + d ≥ k = open loop delay. • Can make it less only by d < 0 , but controller is unrealizable. 10 Digital Control Kannan M. Moudgalya, Autumn 2007

Example: What if Wrong Delay is Specified? 11. Design a controller for the plant 1 G = z − 2 1 − 0 . 5 z − 1 so that the overall system has smaller delay: 1 T = z − 1 1 − az − 1 Recall the standard closed loop transfer function: T = GG c / (1+ GG c ) . Solving for G c , and substituting for T , G 1 − 0 . 5 z − 1 G c = 1 T 1 1 − T = z − 1 1 − ( a + 1) z − 1 G This controller is unrealizable, no matter what a is. 11 Digital Control Kannan M. Moudgalya, Autumn 2007

Specifications - Step Response 12. Give unit step input in r . Output y y ( n ) should have 1. small rise time n 2. small overshoot e ( n ) 3. small settling time 4. small steady state error n Im( z ) Error e ( n ) of the following form sat- isfies the requirements: × ρ ω e ( n ) = ρ n cos ωn, 0 < ρ < 1 Re( z ) × 12 Digital Control Kannan M. Moudgalya, Autumn 2007

Specifications - Step Response 13. 1. initial error is one y ( n ) 2. decaying oscillations about zero 3. steady state error is zero n e ( n ) Procedure: User will specify the following: 1. a maximum allowable fall time < N r n Im( z ) 2. a maximum allowable undershoot < ε × 3. a minimum required decay ratio < δ ρ ω Re( z ) • We will develop a method to determine ρ and × ω satisfying the above requirements e ( n ) = ρ n cos ωn • Calculate trans. fn. between e ( n ) - r ( n 0 < ρ < 1 • Back calculate the controller G c ( z ) 13 Digital Control Kannan M. Moudgalya, Autumn 2007

Small Fall Time in Error 14. e ( n ) = ρ n cos ωn, y ( n ) 0 < ρ < 1 Error becomes zero, i.e. , n e ( n ) = 0 . e ( n ) for the first time when ωn = π n 2 ⇒ n = π Desired region: 2 ω Im( z ) As want n < N r , some given value, we get Re( z ) π π 2 ω < N r ⇒ ω > 2 N r 14 Digital Control Kannan M. Moudgalya, Autumn 2007

Small Undershoot 15. y ( n ) e ( n ) = ρ n cos ωn When does it reach first min.? de/dn = 0 n e ( n ) • Not applicable, because n is an integer • Look for a simpler expression • Reaches min. approx. when ωn = π n Desired region: e ( n ) | ωn = π = ρ n cos ωn | ωn = π Im( z ) = − ρ n | ωn = π = − ρ π/ω Re( z ) User specified maximum deviation = ε : ρ π/ω < ε, ρ < ε ω/π 15 Digital Control Kannan M. Moudgalya, Autumn 2007

Small Decay Ratio 16. e ( n ) = ρ n cos ωn y ( n ) Ratio of two successive peak/trough to be small • First undershoot in e ( n ) occurs at ωn ≃ π n • First overshoot occurs at ωn ≃ 2 π e ( n ) Want this ratio to be less than user specified δ : � < δ ⇒ ρ n | ωn =2 π � e ( n ) | ωn =2 π � � � < δ n � � ρ n | ωn = π e ( n ) | ωn = π � δ = 0 . 5 ≃ 1/4 decay. δ = 0 . 25 ≃ 1/8 decay. Desired region: Im( z ) ρ 2 π/ω ρ π/ω < δ ⇒ ρ π/ω < δ ⇒ ρ < δ ω/π Re( z ) • Small undershoot: ρ < ε ω/π . Usually ε < δ • Small undershoot satisfies fast decay 16 Digital Control Kannan M. Moudgalya, Autumn 2007

Overall Requirements 17. Im( z ) Im( z ) Im( z ) Re( z ) Re( z ) Re ( z ) Desired region by the current approach Im(z) Re(z) Obtained by discretization of continuous domain result (Astrom and Wittenmark) 17 Digital Control Kannan M. Moudgalya, Autumn 2007

Desired Transfer Function 18. Desired error to a step input is e: e ( n ) = r n cos ωn Taking Z-transform, z ( z − r cos ω ) E ( z ) = z 2 − 2 zr cos ω + r 2 For step input R ( z ) . Transfer function between R ( z ) - E ( z ) : T E ( z ) = E ( z ) z ( z − r cos ω ) z − 1 R ( z ) = z 2 − 2 zr cos ω + r 2 z = ( z − 1)( z − r cos ω ) z 2 − 2 zr cos ω + r 2 One approach: equate this to 1 / (1 + GG c ) and calculate G c 18 Digital Control Kannan M. Moudgalya, Autumn 2007