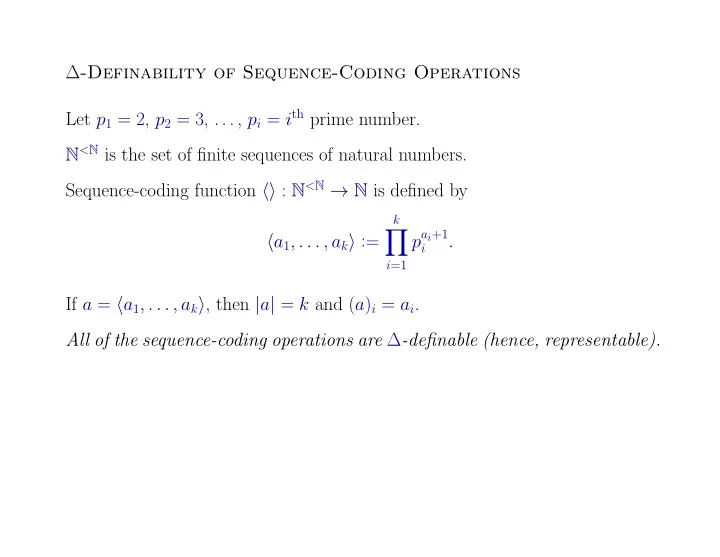
-Definability of Sequence-Coding Operations Let p 1 = 2, p 2 = 3, . - PowerPoint PPT Presentation

∆ -Definability of Sequence-Coding Operations Let p 1 = 2, p 2 = 3, . . . , p i = i th prime number. N < N is the set of finite sequences of natural numbers. Sequence-coding function �� : N < N → N is defined by k � p a i +1 � a 1 , . . . , a k � := . i i =1 If a = � a 1 , . . . , a k � , then | a | = k and ( a ) i = a i . All of the sequence-coding operations are ∆ -definable (hence, representable).

We have ∆-formulas: Divides ( y, x ) : ≡ ( ∃ z ≤ x )[ x = y · z ] , Prime ( x ) : ≡ S 0 < x ∧ ( ∀ y ≤ x )[ Divides ( y, x ) → ( y = 1 ∨ y = x )]

We have ∆-formulas: Divides ( y, x ) : ≡ ( ∃ z ≤ x )[ x = y · z ] , Prime ( x ) : ≡ S 0 < x ∧ ( ∀ y ≤ x )[ Divides ( y, x ) → ( y = 1 ∨ y = x ) ] � �� � ¬ Divides ( y, x ) ∨ ( y = 1 ∨ y = x ) Remark: Technically, ¬ Divides ( y, x ) is not a ∆-formula. However, it is equivalent to a ∆-formula.

The set PrimePair := { ( p i , p i +1 ) : i ≥ 1 } ⊆ N 2 is defined by the ∆-formula PrimePair ( x, y ) : ≡ Prime ( x ) ∧ Prime ( y ) ∧ x < y ∧ ( ∀ z < y )[ Prime ( z ) → z ≤ x ] .

The set PrimePair := { ( p i , p i +1 ) : i ≥ 1 } ⊆ N 2 is defined by the ∆-formula PrimePair ( x, y ) : ≡ Prime ( x ) ∧ Prime ( y ) ∧ x < y ∧ ( ∀ z < y )[ Prime ( z ) → z ≤ x ] . The set : ( a 1 , . . . , a k ) ∈ N < N } ⊆ N Codenumber = { � a 1 , . . . , a k � � �� � p a 1+1 p a 2+1 ··· p ak +1 1 2 k is defined by the ∆-formula Codenumber ( c ) : ≡ Divides ( SS 0 , c ) ∧ ( ∀ z < c )( ∀ y < z ) �� � � PrimePair ( y, z ) ∧ Divides ( z, c ) → Divides ( y, c ) . This formula expresses: “ c is divisible by 2 and for every prime pair ( p i , p i +1 ), if p i +1 divides c , so p i divides c .”

Continuing, we define the set Yardstick := {� 0 , 1 , 2 , . . . , k − 1 � : k ∈ N } � �� � 2 1 3 2 5 3 ... ( p k ) k by the ∆-formula Yardstick ( x ) : ≡ Divides (2 , x ) ∧ ¬ Divides (4 , x ) ∧ ( ∀ y ≤ x )( ∀ z ≤ x )( ∀ i < x ) �� � � PrimePair ( y, z ) ∧ Divides ( z, x ) . � � → Divides ( y E i , x ) ↔ Divides ( z E Si , x ) � �� � ���� z i +1 y i

We next define the set IthPrime := { ( i, p i ) : i ∈ N ≥ 1 } by the ∆-formula   Yardstick ( x ) ∧ Divides ( y i , x ) ∧   IthPrime ( i, y ) : ≡ Prime ( y ) ∧ ( ∃ x ≤ some term)  .  ¬ Divides ( y i +1 , x ) Question: What term suffices for this bounded quantifier?

We next define the set IthPrime := { ( i, p i ) : i ∈ N ≥ 1 } by the ∆-formula   Yardstick ( x ) ∧ IthPrime ( i, y ) : ≡ Prime ( y ) ∧ ( ∃ x ≤ y i 2 ) Divides ( y i , x ) ∧    .  ¬ Divides ( y i +1 , x ) Question: What term suffices for this bounded quantifier? Answer: We want x to be the i -th yardstick number ( p 1 ) 1 ( p 2 ) 2 . . . ( p i ) i . This is at most ( p i )( p i ) 2 ( p i ) 3 · · · ( p i ) i = ( p i )( i +1 2 ) ≤ ( p i ) i 2 . Therefore, if y = p i , it suffices to take x ≤ y i 2 .

We next define the set IthPrime := { ( i, p i ) : i ∈ N ≥ 1 } by the ∆-formula   Yardstick ( x ) ∧ IthPrime ( i, y ) : ≡ Prime ( y ) ∧ ( ∃ x ≤ y i 2 ) Divides ( y i , x ) ∧    .  ¬ Divides ( y i +1 , x ) Question: What term suffices for this bounded quantifier? Answer: We want x to be the i -th yardstick number ( p 1 ) 1 ( p 2 ) 2 . . . ( p i ) i . This is at most ( p i )( p i ) 2 ( p i ) 3 · · · ( p i ) i = ( p i )( i +1 2 ) ≤ ( p i ) i 2 . Therefore, if y = p i , it suffices to take x ≤ y i 2 . Alternatively, since p i ≤ ( i + 1) i (easy fact), we could instead use x ≤ ( i + 1) i 2 .

We next define the set IthPrime := { ( i, p i ) : i ∈ N ≥ 1 } by the ∆-formula   Yardstick ( x ) ∧ IthPrime ( i, y ) : ≡ Prime ( y ) ∧ ( ∃ x ≤ y i · i ) Divides ( y i , x ) ∧    .  ¬ Divides ( y i +1 , x ) Exercise. Convince yourself that IthPrime ( i, y ) indeed defines IthPrime . That is, show that • N | = IthPrime ( k, p k ) for every k ≥ 1, • N | = ¬ IthPrime ( a, b ) whenever a = 0 or b � = p a .

We next define the set IthPrime := { ( i, p i ) : i ∈ N ≥ 1 } by the ∆-formula   Yardstick ( x ) ∧ IthPrime ( i, y ) : ≡ Prime ( y ) ∧ ( ∃ x ≤ y i · i ) Divides ( y i , x ) ∧    .  ¬ Divides ( y i +1 , x ) Remark. The set IthPrime ⊆ N 2 corresponds to the function N → N defined by i �→ p i . Therefore, we say that the formula IthPrime ( i, y ) defines the function i �→ p i .

Continuing, the set � ( � a 1 , . . . , a k � , k ) : k ≥ 1 and ( a 1 , . . . , a k ) ∈ N k � Length := is defined by the ∆-formula Length ( c, ℓ ) : ≡ Codenumber ( c ) � IthPrime ( ℓ, y ) ∧ Divides ( y , c ) � ∧ ( ∃ y ≤ c ) . ∧ ( ∀ z ≤ c )[ PrimePair ( y, z ) → ¬ Divides ( z, c )]

Continuing, the set � ( � a 1 , . . . , a k � , k ) : k ≥ 1 and ( a 1 , . . . , a k ) ∈ N k � Length := is defined by the ∆-formula Length ( c, ℓ ) : ≡ Codenumber ( c ) � IthPrime ( ℓ, y ) ∧ Divides ( y , c ) � ∧ ( ∃ y ≤ c ) . ∧ ( ∀ z ≤ c )[ PrimePair ( y, z ) → ¬ Divides ( z, c )] The set � ( a j , j, � a 1 , . . . , a k � ) : 1 ≤ j ≤ k and ( a 1 , . . . , a k ) ∈ N k � IthElement := is defined by the ∆-formula IthElement ( e, i, c ) : ≡ Codenumber ( c ) ∧ ( ∃ y ≤ c ) � � IthPrime ( i, y ) ∧ Divides ( y Se , c ) ∧ ¬ Divides ( y SSe , c ) .

∆ -Definability of Sequence-Coding Operations For practice, try writing a ∆-formula that defines the set Concatenation ⊆ N 3 of triples of the form ( � a 1 , . . . , a k � , � b 1 , . . . , b ℓ � , � a 1 , . . . , a k , b 1 , . . . , b ℓ � ).

G¨ odel Numbers of Terms and Formulas We assign a unique number to each symbol in L NT as follows: ¬ 1 · 15 ∨ 3 E 17 ∀ 5 < 19 = 7 ( 21 0 9 ) 23 S 11 v i 2 i + 13 Suppose s : ≡ s 1 . . . s n is a string of symbols, which constituting a well-formed term or formula of L NT . Naively, we could encode s by the number � #( s 1 ) , . . . , #( s n ) � where #( s i ) is the number corresponding to the symbol s i . However, it much better to encode s according to the inductive type of terms and formulas.

Def 5.7.1. For each term t and formula ϕ , the G¨ odel numbers � t � and � ϕ � are defined as follows: � ¬ α � = � 1 , � α � � � + t 1 t 2 � = � 13 , � t 1 � , � t 2 � � � ( α ∨ β ) � = � 3 , � α � , � β � � � · t 1 t 2 � = � 15 , � t 1 � , � t 2 � � � ( ∀ v i )( α ) � = � 5 , � v i � , � α � � � Et 1 t 2 � = � 17 , � t 1 � , � t 2 � � � = t 1 t 2 � = � 7 , � t 1 � , � t 2 � � � <t 1 t 2 � = � 19 , � t 1 � , � t 2 � � � 0 � = � 9 � � v i � = � 2 i � . � St � = � 11 , � t � �

Def 5.7.1. For each term t and formula ϕ , the G¨ odel numbers � t � and � ϕ � are defined as follows: � ¬ α � = � 1 , � α � � � + t 1 t 2 � = � 13 , � t 1 � , � t 2 � � � ( α ∨ β ) � = � 3 , � α � , � β � � � · t 1 t 2 � = � 15 , � t 1 � , � t 2 � � � ( ∀ v i )( α ) � = � 5 , � v i � , � α � � � Et 1 t 2 � = � 17 , � t 1 � , � t 2 � � � = t 1 t 2 � = � 7 , � t 1 � , � t 2 � � � <t 1 t 2 � = � 19 , � t 1 � , � t 2 � � � 0 � = � 9 � � v i � = � 2 i � . � St � = � 11 , � t � � Obs. � t � and � ϕ � are never divisible by 7. (Why?)

Def 5.7.1. For each term t and formula ϕ , the G¨ odel numbers � t � and � ϕ � are defined as follows: � ¬ α � = � 1 , � α � � � + t 1 t 2 � = � 13 , � t 1 � , � t 2 � � � ( α ∨ β ) � = � 3 , � α � , � β � � � · t 1 t 2 � = � 15 , � t 1 � , � t 2 � � � ( ∀ v i )( α ) � = � 5 , � v i � , � α � � � Et 1 t 2 � = � 17 , � t 1 � , � t 2 � � � = t 1 t 2 � = � 7 , � t 1 � , � t 2 � � � <t 1 t 2 � = � 19 , � t 1 � , � t 2 � � � 0 � = � 9 � � v i � = � 2 i � . � St � = � 11 , � t � � Example. � =0 S 0 � = � 7 , � 0 � , � S 0 � � = � 7 , � 9 � , � 11 , � 9 ��� = � 7 , 2 10 , � 11 , 2 10 �� = 2 8 3 1025 5 (2 12 3 1025 +1) . Notice how fast � SSSS 0 � grows: � SSSS 0 � = � 11 , � 11 , � 11 , � 11 , � 9 ����� = 2 12 3 2 12 3 21232123210

Next Steps (Section 5.8) ∆-definability of sets Terms := { � t � : terms t } = { a ∈ N : a = � t � for some term t } , Formulas := { � ϕ � : formulas ϕ } = { a ∈ N : a = � ϕ � for some formula ϕ } .