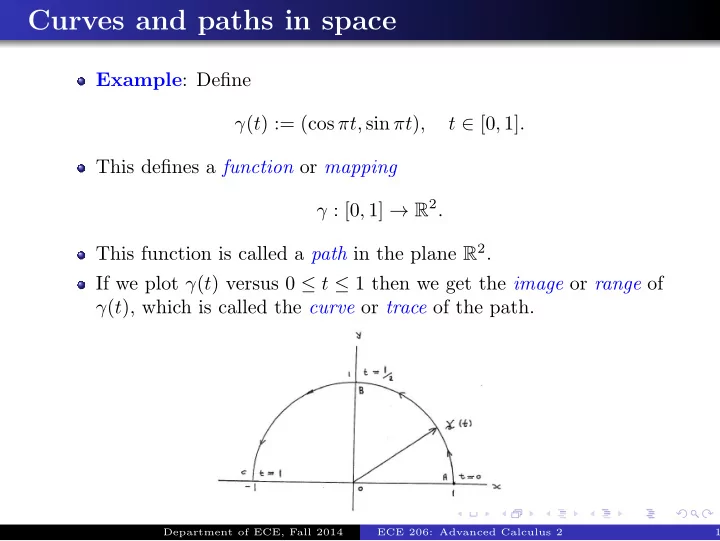
Curves and paths in space Example : Define ( t ) := (cos t, sin t ) - PowerPoint PPT Presentation

Curves and paths in space Example : Define γ ( t ) := (cos πt, sin πt ) , t ∈ [0 , 1] . This defines a function or mapping γ : [0 , 1] → R 2 . This function is called a path in the plane R 2 . If we plot γ ( t ) versus 0 ≤ t ≤ 1 then we get the image or range of γ ( t ), which is called the curve or trace of the path. Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 1/18

Parametric representation of curves Definition A two-dimensional path (or parametric function for a two-dimensional curve) is a given function or mapping γ : [0 , 1] → R 2 , from a specified interval [ a, b ] into R 2 , which maps each a ≤ t ≤ b into the vector γ ( t ) ∈ R 2 . In terms of scalar components, we usually write: γ ( t ) = ( x ( t ) , y ( t )) = x ( t ) i + y ( t ) j , ∀ t ∈ [ a, b ] . The curve of the path is the set of points Γ ⊂ R 2 traced by γ ( t ) as t traverses the interval [ a, b ]. Formally: γ ( t ) ∈ R 2 | t ∈ [ a, b ] � � Γ := . Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 2/18

Parametric representation of curves (cont.) The interval a ≤ t ≤ b is called the parametric interval , t is called the parametric variable of the path, and the whole function γ ( t ) is called a parametric representation of the curve Γ. The starting point of the path is the vector γ ( a ), while the ending point of the path is the vector γ ( b ). The curve Γ has a direction from the starting to the ending point corresponding to t increasing from t = a until t = b . Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 3/18

Parametric curves in R 3 Definition A three-dimensional path (or parametric function for a three-dimen- sional curve) is a given function or mapping γ : [0 , 1] → R 3 , from a specified interval [ a, b ] into R 3 , which maps each a ≤ t ≤ b into the vector γ ( t ) ∈ R 3 . Usually, γ ( t ) is written in the scalar component form γ ( t ) = ( x ( t ) , y ( t ) , z ( t )) = x ( t ) i + y ( t ) j + z ( t ) k , ∀ t ∈ [ a, b ] . The curve of the path is the set of points Γ ⊂ R 3 traced by γ ( t ) as t traverses the interval a ≤ t ≤ b . Formally: γ ( t ) ∈ R 3 | t ∈ [ a, b ] � � Γ := . Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 4/18

Parametric curves in R 3 (cont.) The remaining definitions are word-for-word identical to the R 2 case, except that everywhere we just replace R 2 with R 3 . Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 5/18

More definitions Definition If the path γ : [ a, b ] → R 3 is such that the first derivatives dx ( t ) dy ( t ) dz ( t ) dt , dt , dt exist and are continuous for each t ∈ [ a, b ], then γ is called a C 1 -path and the curve corresponding to γ is called a C 1 -curve. Definition If γ : [ a, b ] → R 3 is a C 1 path and additionally the derivatives d 2 x ( t ) d 2 y ( t ) d 2 z ( t ) , , dt 2 dt 2 dt 2 exist and are continuous for each t ∈ [ a, b ], then γ is called a C 2 -path and the curve corresponding to γ is called a C 2 -curve. Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 6/18

Paths and curves Different paths can have identical curves! For example, define γ ( t ) := (cos(2 πt ) , sin(2 πt )), 0 ≤ t ≤ 1 / 2, which results in a mapping γ : [0 , 1 / 2] → R 2 . The curve corresponding to this path is identical to that of (cos πt, sin πt ) with t ∈ [0 , 1]. Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 7/18

Paths and curves (cont.) To further illustrate the idea, let us define γ 1 ( t ) = (cos πt, sin πt ) with t ∈ [0 , 1]. √ 2] → R , ψ ( s ) := s 2 − 1. Now define the function ψ : [1 , √ √ 2) = 1, ψ ′ ( s ) = 2 s > 0, ∀ s ∈ [1 , We see that ψ (1) = 0, ψ ( 2]. The function ψ ( s ) increases strictly monotonically through the √ interval [0 , 1] as s increases through the interval 1 ≤ s ≤ 2. Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 8/18

Paths and curves (cont.) √ 2] → R 2 as Now define the path γ 2 : [1 , cos( π ( s 2 − 1)) , sin( π ( s 2 − 1)) � � � γ 2 ( s ) := γ 1 ( ψ ( s )) = , 1 ≤ s ≤ (2) . The paths γ 1 and γ 2 are clearly different, but they nevertheless have the same curve. In fact, γ 1 and γ 2 are distinct parametric representations of the same curve . Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 9/18

Re-parametrization More generally, suppose we have γ 1 : [ a 1 , b 1 ] → R 3 . In order to change the parametrization to get a different path with exactly the same curve, we fix some interval [ a 2 , b 2 ] along with some strictly increasing C 1 -function ψ : [ a 2 , b 2 ] → R such that ψ ( a 2 ) = a 1 , ψ ( b 2 ) = b 1 , ψ ′ ( s ) > 0 , ∀ s ∈ [ a 2 , b 2 ] . Then the path γ 2 : [ a 2 , b 2 ] → R 3 defined by γ 2 ( s ) := γ 1 ( ψ ( s )) , a 2 ≤ s ≤ b 2 gives a different parametric representation of the same curve. Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 10/18

Tangent to a curve Given a path γ : [ a, b ] → R 3 represented as γ ( t ) = ( x ( t ) , y ( t ) , z ( t )) = x ( t ) i + y ( t ) j + z ( t ) k , its derivative w.r.t t is the vector in R 3 given by γ (1) ( t ) := dγ ( t ) � dx ( t ) dt , dy ( t ) dt , dz ( t ) � = dx ( t ) dt i + dy ( t ) dt j + dz ( t ) = dt k , dt dt for all a ≤ t ≤ b . Similarly, the second derivative of the path w.r.t. t is given by γ (2) ( t ) := dγ (1) ( t ) � dx 2 ( t ) , dy 2 ( t ) , dz 2 ( t ) � = = dt dt 2 dt 2 dt 2 = dx 2 ( t ) i + dy 2 ( t ) j + dz 2 ( t ) k , dt 2 dt 2 dt 2 for all a ≤ t ≤ b . Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 11/18

Tangent to a curve (cont.) The fact that γ ( t ) = ( x ( t ) , y ( t ) , z ( t ) along with dx x ( t + ∆ t ) − x ( t ) dt = lim ∆ t ∆ t → 0 dy y ( t + ∆ t ) − y ( t ) dt = lim ∆ t ∆ t → 0 dz z ( t + ∆ t ) − z ( t ) dt = lim ∆ t ∆ t → 0 suggests that dγ γ ( t + ∆ t ) − γ ( t ) dt = lim ∆ t ∆ t → 0 Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 12/18

Tangent to a curve (cont.) The difference vector γ ( t + ∆ t ) − γ ( t ) is vey close to tangential to the curve of the path. When ∆ t is small, and the limit of ( γ ( t + ∆ t ) − γ ( t )) / ∆ t is exactly tangent to the curve of the path at γ ( t ). Thus, the derivative γ (1) ( t ) is tangent to the curve of the path γ : [ a, b ] → R 3 at γ ( t ) for each instant a ≤ t ≤ b . Similarly, one can see that the derivative γ (2) ( t ) is tangent to the curve of the path γ (1) : [ a, b ] → R 3 at γ (1) ( t ) for each a ≤ t ≤ b . Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 13/18

Products of curves Given two paths γ 1 : [ a, b ] → R 3 γ 2 : [ a, b ] → R 3 , and the inner product of the vectors γ 1 ( t ) and γ 2 ( t ) in R 3 for each a ≤ t ≤ b gives a scalar-valued function ϕ ( t ) := γ 1 ( t ) · γ 2 ( t ) , a ≤ t ≤ b. By the rule for differentiation of products, we have: dϕ ( t ) 2 ( t ) + γ 1 ( t ) (1) · γ 2 ( t ) , = γ 1 ( t ) · γ (1) ∀ t ∈ [ a, b ] . dt Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 14/18

Velocity and acceleration The parametric variable for a given path γ : [ a, b ] → R 3 does not have any “physical” interpretation, in general. However, in many applications, t may be interpreted as time (and hence [ a, b ] as a time interval ). Moreover, if γ ( t ) represents a physical point moving in space, one can introduce the notions of velocity v and acceleration a , namely v ( t ) := γ (1) ( t ) , a ( t ) := γ (2) ( t ) , for all a ≤ t ≤ b . Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 15/18

Velocity and acceleration (cont.) Now, let F : D → R 3 be a vector field (e.g., a force acting on a particle of mass m ) defined over the domain D ⊂ R 3 . Suppose that, in response to this force, the particle follows the path γ : [ a, b ] → R 3 . Newton’s second law then says that m a ( t ) = F ( γ ( t )) , t ∈ [ a, b ] . Alternatively, one has mγ (2) ( t ) = F ( γ ( t )) , t ∈ [ a, b ] . This is a second order vector differential equation which can, in principle, be solved to get the path γ : [ a, b ] → R 3 followed by the particle if one knows the force vector field F . Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 16/18

Simple curves and closed curves Definition If the path γ : [ a, b ] → R 3 has the property that, for any t 1 , t 2 ∈ [ a, b ], γ ( t 1 ) � = γ ( t 2 ) when t 1 � = t 2 , the corresponding curve is called simple . Simply put, a simple curve has the property that it does not “cross itself” anywhere (as opposed to non-simple curves ). Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 17/18

Simple curves and closed curves (cont.) Definition If the path γ : [ a, b ] → R 3 has the property that γ ( a ) = γ ( b ), the corresponding curve (simple or not) is called closed . A closed curve which is also simple is called a simple closed curve whereas a closed curve which does cross itself somewhere is a non- simple closed curve . Department of ECE, Fall 2014 ECE 206: Advanced Calculus 2 18/18