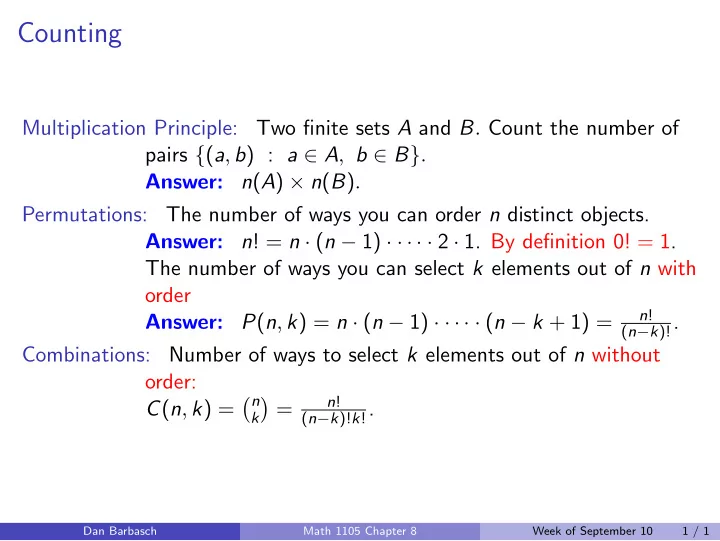
Counting Multiplication Principle: Two finite sets A and B . Count - PowerPoint PPT Presentation

Counting Multiplication Principle: Two finite sets A and B . Count the number of pairs { ( a , b ) : a ∈ A , b ∈ B } . n ( A ) × n ( B ) . Answer: Permutations: The number of ways you can order n distinct objects. n ! = n · ( n − 1) · · · · · 2 · 1. By definition 0! = 1. Answer: The number of ways you can select k elements out of n with order n ! P ( n , k ) = n · ( n − 1) · · · · · ( n − k + 1) = ( n − k )! . Answer: Combinations: Number of ways to select k elements out of n without order: � n n ! � C ( n , k ) = = ( n − k )! k ! . k Dan Barbasch Math 1105 Chapter 8 Week of September 10 1 / 1

Examples I Example (1) Four men and three women are to be seated in seven chairs lined up in a row. 1 In how many ways can this be done, assuming the men and women are individually distinguishable? For example the men are John, David, Steve and Bill, and the women are Mary, Ellen and Ann. 2 In how many ways can this be done, assuming the men and women are not distinguishable? In other words we can only distinguish men from women but not exactly who they are. Dan Barbasch Math 1105 Chapter 8 Week of September 10 2 / 1

Examples II Example (2, Radio Station Call Letters) How many different 4-letter radio station call letters can be made if a. the first letter must be K or W and no letter may be repeated? b. repeats are allowed, but the first letter is K or W? c. the first letter is K or W, there are no repeats, and the last letter is R? Example (3, YOUR TURN 7) A student has 4 pairs of identical blue socks, 5 pairs of identical brown socks, 3 pairs of identical black socks, and 2 pairs of identical white socks. In how many ways can he select socks to wear for the next two weeks? Example (4) In how many ways can we draw three kings and two queens from a standard deck of card? Dan Barbasch Math 1105 Chapter 8 Week of September 10 3 / 1

Examples III Example (5) How many 20 letter words can we make with the letters A , B , C , D so that there are exactly 4 A’s, 5 B’s, 5 C’s and 6 D’s? Example (6) a. How many subsets with k elements can one form from a set with n elements? b. How many subsets with an even number of elements are there? Dan Barbasch Math 1105 Chapter 8 Week of September 10 4 / 1

Examples IV Example (7, Binomial Formula/Theorem) Expand ( a + b ) n . This is often attributed to Newton. According to Wikipedia, Euclid knew n = 2 � n � Ancient Hindus knew formulas for . k Omar Khayam knew the formula for higher n Chinese in the 13 th century knew formulas for small n . Renaissance Mathematicians knew about it. � n � Pascal came up with the Pascal’s Triangle that computes more k efficiently. Newton is credited with the formula when n IS NOT an integer. Answer: n n � n � � n � ( a + b ) n = a k b n − k = � � a n − k b k . k k k =0 k =0 QUESTION: What is the formula for ( a + b + c ) n ? Dan Barbasch Math 1105 Chapter 8 Week of September 10 5 / 1

Dice I A die is tossed 30 times. What is the probability of the Tossing dice: event A that a 2 comes up at least twice? The die is fair, any number is equally likely to come up, and the tosses are independent. Answer. For the number 2 to come up exactly k times, there are C (30 , k ) � 1 � k · � 5 � 30 − k . So the probability is possibilities, each with probability 6 6 � 1 � 5 � k · � 30 − k . We have to add from k = 2 to k = 30 . An C (30 , k ) · 6 6 easier way is to compute 1 − P ( A c ). � 0 � 30 � 1 � 29 � 1 � 5 � 1 � 5 P ( A c ) = C (30 , 0) · · + C (30 , 1) · · . 6 6 6 6 Dan Barbasch Math 1105 Chapter 8 Week of September 10 6 / 1

Birthdays What is the probability of the event A that out of 30 people at least two have the same birthday? There are 365 days in the year. Assume that any person has equal probability of being born on any given day, and these events are independent. There are 365 30 elements in the sample space, equally likely. It is easier to compute 1 − P ( A c ) . The complementary event A c is no two birthdays occur on the same day. Answer. A c is no two birthdays on the same day: P ( A c ) = P (365 , 30) . 365 30 So P ( A ) = 1 − P (365 , 30) . 365 30 Dan Barbasch Math 1105 Chapter 8 Week of September 10 7 / 1

Lottery I Typically in a lottery you choose k numbers out of n . You win prizes if you match some of the numbers. Example (#46 in section 8.2) A state lottery game requires that you pick 6 different numbers from 1 to 99. If you pick all 6 winning numbers, you win the jackpot. a. How many ways are there to choose 6 numbers if order is not important? b. How many ways are there to choose 6 numbers if order matters? Answer. For a), P (99 , 6) . For b), C (99 , 6) . Dan Barbasch Math 1105 Chapter 8 Week of September 10 8 / 1

Lottery II Example (#47 in section 8.2) In Exercise 46, if you pick 5 of the 6 numbers correctly, you win $250,000. In how many ways can you pick exactly 5 of the 6 winning numbers without regard to order? Answer. 6 · 93 = 558 . Example (#66 in 8.3) In the previous section, we found the number of ways to pick 6 different numbers from 1 to 99 in a state lottery. Assuming order is unimportant, what is the probability of picking all 6 numbers correctly to win the big prize? Dan Barbasch Math 1105 Chapter 8 Week of September 10 9 / 1

Lottery III Answer. C (99 , 6) = 8 . 924 × 10 − 10 . 1 P ( picking all 6 ) = Example (#67 in section 8.3) In Exercise 66, what is the probability of picking exactly 5 of the 6 numbers correctly? Answer. 6 × 93 / C (99 , 6) = 4 . 980 × 10 − 7 . Dan Barbasch Math 1105 Chapter 8 Week of September 10 10 / 1

Binomial Distribution Binomial Experiment 1 The same experiment is repeated a fixed number of times. 2 There are only two possible outcomes, success and failure.; P ( success ) = p , P ( failure ) = 1 − p . 3 The repeated trials are independent, so that the probability of success remains the same for each trial. The Binomial Distribution is P ( exactly k successes in n trials) = p k (1 − p ) n − k C ( n , k ) . Examples are a 2 showing in a rool of dice, or H in a toss of coins from before, but NOT birthdays. Dan Barbasch Math 1105 Chapter 8 Week of September 10 11 / 1

Binomial Distribution, Examples I Example A hospital receives 1 / 5 = 0 . 2 of its flu vaccine shipments from Company X and the remainder of its shipments from other companies. Each shipment contains a very large number of vaccine vials. For Company X’s shipments, 10% of the vials are ineffective. For every other company, 2% of the vials are inef- fective. The hospital tests 30 randomly selected vials from a shipment and finds that one vial is ineffective. What is the prob- ability that this shipment came from Company X? Dan Barbasch Math 1105 Chapter 8 Week of September 10 12 / 1

Binomial Distribution, Examples II Answer. P ( X ) = 0 . 2 P ( NX ) = 0 . 8 P ( D | X ) = 0 . 1 P ( D | NX ) = 0 . 02 P (1 D / 30 | X ) = C (30 , 1) × (0 . 1) 1 × (0 . 9) 29 P (1 D / 30 | NX ) = C (30 , 1) × (0 . 02) 1 × (0 . 98) 29 Draw the usual tree diagram for Bayes’s theorem and compute. For X , p = 0 . 1 and 1 − p = 0 . 9, for NX , p = 0 . 02 and 1 − p − 0 . 98 . Dan Barbasch Math 1105 Chapter 8 Week of September 10 13 / 1