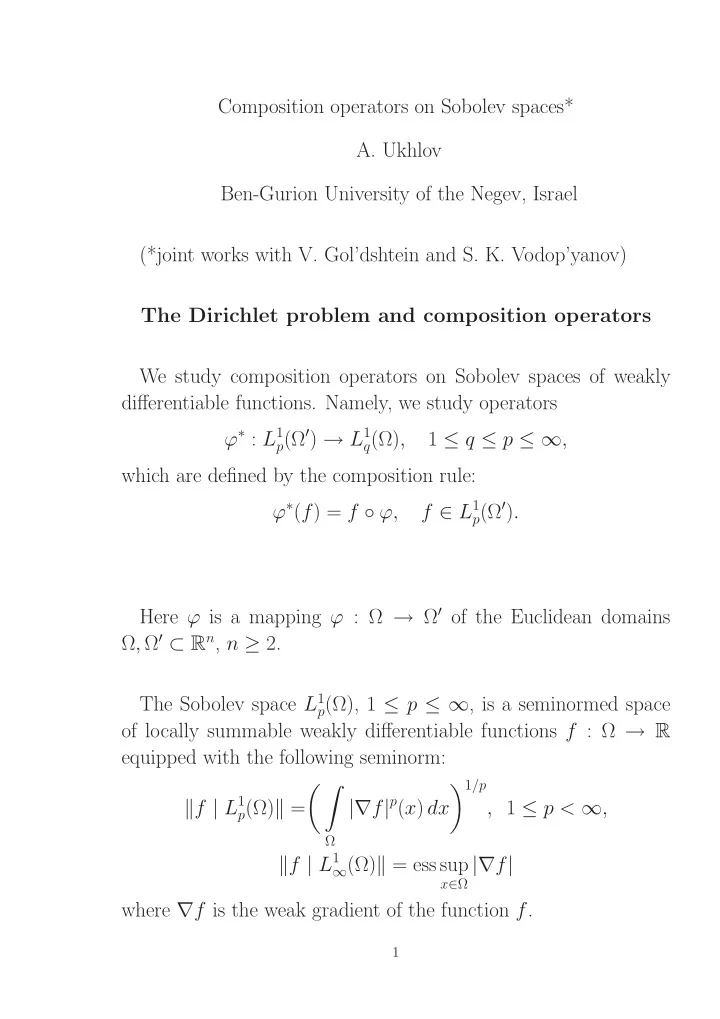

Composition operators on Sobolev spaces* A. Ukhlov Ben-Gurion University of the Negev, Israel (*joint works with V. Gol’dshtein and S. K. Vodop’yanov) The Dirichlet problem and composition operators We study composition operators on Sobolev spaces of weakly differentiable functions. Namely, we study operators ϕ ∗ : L 1 p (Ω ′ ) → L 1 1 ≤ q ≤ p ≤ ∞ , q (Ω) , which are defined by the composition rule: ϕ ∗ ( f ) = f ◦ ϕ, f ∈ L 1 p (Ω ′ ) . Here ϕ is a mapping ϕ : Ω → Ω ′ of the Euclidean domains Ω , Ω ′ ⊂ R n , n ≥ 2. The Sobolev space L 1 p (Ω), 1 ≤ p ≤ ∞ , is a seminormed space of locally summable weakly differentiable functions f : Ω → R equipped with the following seminorm: � 1 /p � � � f | L 1 |∇ f | p ( x ) dx p (Ω) � = , 1 ≤ p < ∞ , Ω � f | L 1 ∞ (Ω) � = ess sup |∇ f | x ∈ Ω where ∇ f is the weak gradient of the function f . 1
The composition operators theory is closely connected with the elliptic equations theory. For example, consider the classical Dirichlet problem for the Laplace operator in the plane domain Ω ⊂ R 2 . ∆ u = 0 , u | ∂ Ω = f. u ∈ C 2 (Ω), ∆ u = ∂ 2 u ∂x 2 + ∂ 2 u ∂y 2 . It is well known that for the unit disc D ⊂ R 2 we have the unique solution of this problem 2 π 1 − r 2 u ( r 0 , θ 0 ) = 1 � 0 0 − 2 r 0 cos( θ − θ 0 ) f ( θ ) dθ 1 + r 2 2 π 0 (the Poisson formula), obtained by means composition of the av- erage value function with the conformal mapping ϕ : D → D . 2
If Ω be a connected simply connected plane domain with non- empty boundary then by the Riemannian Mappings Theorem there exists a conformal homeomorphism ϕ : Ω → D . Recall that a mapping ϕ = u ( x, y ) + iv ( x, y ) : Ω → D is called a conformal homeomorphism if ϕ is differentiable (ana- lytic) in Ω and ϕ ′ ( z ) � = 0, z ∈ Ω. It is well known that a composition of an analytic function and harmonic function is an harmonic function again. So, if a function u is a solution of the Dirichlet problem for the unit disc, then a function u ◦ ϕ is a solution of the Dirichlet problem in the domain Ω ⊂ R 2 (with a corresponding boundary value). Let Ω = { ( x, y ) : y > 0 } (upper half of the plane R 2 ) and u ( x, 0) = f ( x ). The conformal mapping w = ϕ ( z ) = z − z 0 z − z 0 : Ω → D . Then the solution is given by + ∞ u ( x, y ) = 1 y � ( t − x ) 2 + y 2 f ( x ) dx. π −∞ 3
In analytical terms the condition of conformity we can rewrite as: ϕ : Ω → D , is a smooth mapping such that | Dϕ ( x ) | 2 = | J ( x, ϕ ) | , x ∈ Ω . Here Dϕ is the Jacobi matrix of ϕ and J ( x, ϕ ) = det( Dϕ ( x )). Note, that conformal mappings preserve the Dirichlet integral: � � |∇ u ◦ ϕ ) | 2 dz = |∇ u | 2 dw Ω D and we can consider solution of the Dirichlet problem as an applica- tion of the Dirichlet’s principle to the energy integral (R. Courant. ”Dirichlet principle, Conformal mappings and Minimal Surfaces”). In another words we can say that: Conformal mappings generate a bounded composition operator (isomorphism) ϕ ∗ : L 1 2 ( D ) → L 1 ϕ ∗ ( u ) = u ◦ ϕ. 2 (Ω) , Here L 1 2 (Ω) is a semi-normed Sobolev space of weakly differentiable functions with finite Dirichlet integral. 4
The boundary value problem for the p -Laplace operator ∆ p u = div( |∇ u | p − 2 ∇ u ) leads to composition operators in more general Sobolev spaces, namely, L 1 p (Ω). Such type composition operators were studied by many authors (V. Gol’dshtein, S. Hencl, P. Koskela, I. Markina, V. G. Maz’ya, Yu. G. Reshetnyak, A. S. Romanov, A. Ukhlov, S. K. Vodop’yanov ...). The theory of composition operators on Sobolev spaces arises to the Yu. G. Reshetnyak problem (1968) about description of all isomorphisms ϕ ∗ of homogeneous Sobolev spaces L 1 n , generated by quasiconformal mappings ϕ of Euclidean spaces R n , n ≥ 2, by the composition rule ϕ ( f ) = f ◦ ϕ . S. K. Vodop’yanov and V. Gol’dshtein (1975) proved that a homeomorphism ϕ : D → D ′ induces a composition operator ϕ ∗ n ( D ′ ) to L 1 from L 1 n ( D ) if and only if ϕ is a quasiconformal home- omorphism. Because a homeomorphism inverse to quasiconformal is also quasiconformal one then ϕ ∗ is an isomorphism of L 1 n ( D ′ ) and L 1 n ( D ). 5
Recall that a homeomorphism ϕ : Ω → Ω ′ is called quasiconfor- mal if ϕ belongs to the Sobolev class W 1 , 1 loc (Ω) and there exists a constant 1 ≤ K < ∞ such that | Dϕ ( x ) | n ≤ K | J ( x, ϕ ) | for almost all x ∈ Ω . For p � = n a homeomorphisms ϕ : D → D ′ induces an iso- morphisms of Sobolev spaces W 1 p ( D ′ ) and W 1 p ( D ) if and only if ϕ it is a bi-Lipschitz one. This result was proved for p > n S. K. Vodop’yanov and V. Gol’dshtein (1975), for n − 1 < p < n by V. Gol’dshtein and A. S. Romanov (1984) and for 1 ≤ p < n by I. Markina (1990). Homeomorphisms that induce bounded composition operators p ( D ′ ) to L 1 from Sobolev space L 1 p ( D ) were studied S. K. Vodop’ya- nov (1988). A geometric description of such homeomorphisms was obtained for p > n − 1 by V. Gol’dshtein, L. Gurov and A. S. Ro- manov (1995). The multipliers theory was applied to the composition operators theory by V. G. Maz’ya and T. O. Shaposhnikova (1986). 6
New effects arise when we study composition operators with de- creasing summability. In 1993 by A. Ukhlov was proved: A homeomorphism ϕ : Ω → Ω ′ generates by the composition rule ϕ ∗ f = f ◦ ϕ the bounded operator ϕ ∗ : L 1 p (Ω ′ ) → L 1 q (Ω) , 1 ≤ q ≤ p < ∞ , if and only if ϕ ∈ L 1 1 , loc (Ω), has finite distortion, and � | Dϕ | p � q � p − q �� p − q pq K p,q ( f ) = dx < ∞ . | J ( x, ϕ ) | Ω A mapping ϕ : Ω → R n belongs to L 1 1 , loc (Ω) if its coordinate functions ϕ j belong to L 1 1 , loc (Ω), j = 1 , . . . , n . In this case formal � ∂ϕ i � Jacobi matrix Dϕ ( x ) = ∂x j ( x ) , i, j = 1 , . . . , n , and its determi- nant (Jacobian) J ( x, ϕ ) = det Dϕ ( x ) are well defined at almost all points x ∈ Ω. The norm | Dϕ ( x ) | of the matrix Dϕ ( x ) is the norm of the corresponding linear operator Dϕ ( x ) : R n → R n defined by the matrix Dϕ ( x ). We will use the same notation for this matrix and the corresponding linear operator. A mappings ϕ : Ω → R n has a finite distortion if Dϕ ( x ) = 0 for almost all points x that belongs to set Z = { x ∈ D : J ( x, ϕ ) = 0 } . 7
Necessity of studying of Sobolev mappings with integrable distor- tion arises in problems of the non-linear elasticity theory. J. M. Ball (1976, 1981) introduced classes of mappings, defined on bounded domains Ω ∈ R n : A + p,q (Ω) = { ϕ ∈ W 1 p (Ω) : adj Dϕ ∈ L q (Ω) , J ( x, ϕ ) > 0 a. e. in Ω } , p, q > n , where adj Dϕ is the formal adjoint matrix to the Jacobi matrix Dϕ : adj Dϕ ( x ) · Dϕ ( x ) = I · J ( x, ϕ ) . Mappings that generate composition operators on Sobolev spaces are mappings of finite distortion. The theory of mappings of fi- nite distortion is under intensive development at the last decades. In series of works the geometrical and topological properties of these mappings were studied (S. Hencl, J. Heinonen, I. Holopainen, T. Iwaniec, P. Koskela, J. Mal´ y, J. Manfredi, G. Martin, O. Mar- tio, P. Pankka, V. Ryazanov, U. Srebro, V. ˇ Sver´ ak, E. Villamor, E. Yakubov). 8
Mappings that generate bounded composition operators on So- bolev spaces ϕ ∗ : L 1 p (Ω ′ ) → L 1 q (Ω) , 1 ≤ q ≤ p ≤ ∞ , are natural generalization of (quasi)conformal mappings (mappings of bounded distortion and we call them mappings of bounded ( p, q )-distortion. In the description of composition operators on Sobolev spaces the significant role belongs the additive set function which allows to localize the composition operators. Let a mapping ϕ : Ω → Ω ′ , where Ω , Ω ′ are domains of Eu- clidean space R n , generates a bounded composition operator ϕ ∗ : L 1 p (Ω ′ ) → L 1 q (Ω) , 1 ≤ q < p ≤ ∞ . Then � r �� � ϕ ∗ f | L 1 � q (Ω) � Φ( A ′ ) = sup , � � � f | L 1 p ( A ′ ) p ( A ′ ) ∩ C 0 ( A ′ ) f ∈ L 1 � where number r is defined according to 1 /r = 1 /q − 1 /p , is a bounded monotone countably additive function defined on open bounded subsets A ′ ⊂ Ω ′ . 9
The description of the composition operators is based on the following local estimate: � p − q | Dϕ ( x ) | q ≤ p | J ( x, ϕ | Φ ′ ( ϕ ( x )) � where Φ ′ is the volume derivative of the set function Φ. Let a monotone finitely additive set function Φ be defined on open subsets of a domain Ω ⊂ R n . Then for almost all points x ∈ Ω the volume derivative Φ( B δ ) Φ ′ ( x ) = lim | B δ | δ → 0 ,B δ ∋ x is finite and for any open set U ⊂ Ω, the inequality � Φ ′ ( x ) dx ≤ Φ( U ) U is valid. 10
It is well known that the mapping inverse to a quasiconformal homeomorphism is quasiconformal also. We have the similar result for homeomorphisms with bounded ( p, q )-distortion. Let ϕ : Ω → Ω ′ be a homeomorphism with bounded ( p, q )- distortion, q > n − 1. Then the inverse mapping ϕ − 1 : Ω ′ → Ω is a mapping with bounded ( q ′ , p ′ )-distortion, q ′ = q/ ( q − n + 1) and p ′ = p/ ( p − n + 1). The main difficult here is to prove that the mapping which is inverse to a homeomorphism with bounded ( p, q )-distortion is the Sobolev mapping. 11
Recommend
More recommend