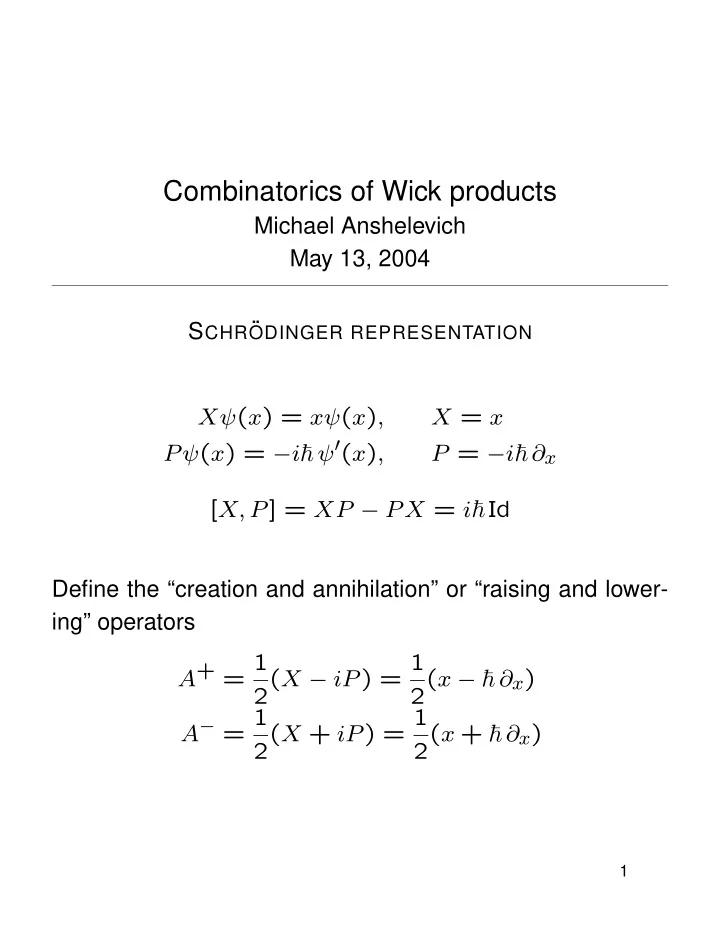
Combinatorics of Wick products Michael Anshelevich May 13, 2004 S - PDF document

Combinatorics of Wick products Michael Anshelevich May 13, 2004 S CHR ¨ ODINGER REPRESENTATION Xψ ( x ) = xψ ( x ) , X = x Pψ ( x ) = − i � ψ ′ ( x ) , P = − i � ∂ x [ X, P ] = XP − PX = i � Id Define the “creation and annihilation” or “raising and lower- ing” operators A + = 1 2( X − iP ) = 1 2( x − � ∂ x ) A − = 1 2( X + iP ) = 1 2( x + � ∂ x ) 1

Thus X = A + + A − , P = i ( A + − A − ) . Then [ A − , A + ] = 1 4[ X, − iP ] + 1 4[ iP, X ] = ( � / 2)Id . Mathematics: set � / 2 = 1 . n - DIMENSIONAL CASE X j ψ = x j ψ P j ψ = − i∂ x j ψ X j ’s commute among themselves. j = 1 j = 1 A + A − 2( X j − iP j ) , 2( X j + iP j ) , j , A + [ A − k ] = δ jk . 2

F OCK REPRESENTATION Let H 0 = real Hilbert space, for example R k . Let H = its complexification, for example C k . Denote H ⊗ n = H ⊗ s H ⊗ . . . ⊗ H , H ⊗ 0 = C Ω , Ω = � �� � n special vector. n = number of particles, Ω = zero particles, vacuum vector. Define the symmetric Fock space ∞ � H ⊗ n F s ( H ) = n =0 = C Ω ⊕ H ⊕ ( H ⊗ s H ) ⊕ ( H ⊗ s H ⊗ s H ) ⊕ . . . . A Hilbert space, with an inner product making levels orthog- onal. 3

For f ∈ H 0 , define a + ( f ) , a − ( f ) : F s ( H ) → F s ( H ) a + ( f ) Ω = f, a + ( f ) ( g 1 ⊗ . . . ⊗ g n ) = f ⊗ g 1 ⊗ . . . ⊗ g n , a − ( f ) Ω = 0 , a − ( f ) g = � f, g � , n � a − ( f ) ( g 1 ⊗ . . . ⊗ g n ) = � f, g k � g 1 ⊗ . . . ⊗ ˆ g k ⊗ . . . ⊗ g n . k =1 Note a − ( f ) a + ( h )( g 1 ⊗ . . . ⊗ g n ) = � f, h � ( g 1 ⊗ . . . ⊗ g n ) + a + ( h ) a − ( f )( g 1 ⊗ . . . ⊗ g n ) , so [ a − ( f ) , a + ( h )] = � f, h � Id . If f 1 , . . . , f n = orthonormal basis for H 0 , � � [ a − ( f j ) , a + ( f k )] = f j , f k = δ jk . 4

Denote X ( f ) = a + ( f ) + a − ( f ) . Thus a + ( f ) , a − ( h ) in general do not commute. But the po- sition operators do: [ X ( f ) , X ( h )] = [ a + ( f ) , a − ( h )] + [ a − ( f ) , a + ( h )] = � f, h � − � h, f � = 0 since f, g real . Question 1. � Ω , X ( f 1 ) X ( f 2 ) . . . X ( f n ) Ω � =? This equals to � � � � � � a + ( f n ) + a − ( f n ) Ω , a + ( f 1 ) + a − ( f 1 ) Ω . . . � � � Ω , a ε (1) ( f 1 ) . . . a ε ( n ) ( f n ) Ω = , ε ∈ I ( n ) I ( n ) = { + , −} n = { ε : ε ( i ) = + or −} . Question 0 . � � Ω , a ε (1) ( f 1 ) . . . a ε ( n ) ( f n ) Ω =? 5

Example 1. � � Ω , a − ( f 1 ) a − ( f 2 ) a + ( f 3 ) a − ( f 4 ) a + ( f 5 ) a + ( f 6 ) Ω f 6 f 5 ⊗ f 6 � f 4 , f 5 � f 6 + � f 4 , f 6 � f 5 � f 4 , f 5 � f 3 ⊗ f 6 + � f 4 , f 6 � f 3 ⊗ f 5 � f 4 , f 5 � � f 2 , f 3 � f 6 + � f 4 , f 6 � � f 2 , f 3 � f 5 + � f 4 , f 5 � � f 2 , f 6 � f 3 + � f 4 , f 6 � � f 2 , f 5 � f 3 � f 4 , f 5 � � f 2 , f 3 � � f 1 , f 6 � + � f 4 , f 6 � � f 2 , f 3 � � f 1 , f 5 � + � f 4 , f 5 � � f 2 , f 6 � � f 1 , f 3 � + � f 4 , f 6 � � f 2 , f 5 � � f 1 , f 3 � 6

Let P 2 ( n ) = a set of all pairings (perfect matchings, pair partitions, Feynman diagrams) � � � � γ = γ − (1) , γ + (1) γ − (2) , γ + (2) , . . . (4 , 5)(2 , 3)(1 , 6) , etc. Clearly P 2 ( odd ) = ∅ . A pairing γ ∈ P 2 ( n ) is consistent with ε if ε ( γ − ( i )) = − , ε ( γ + ( i )) = + . Write γ ∈ P ( ε ) . For ε = ( − , − , + , − , + , +) , the consistent pairings are (1 , 3)(2 , 5)(4 , 6) , (1 , 3)(2 , 6)(4 , 5) , (1 , 5)(2 , 3)(4 , 6) , (1 , 6)(2 , 3)(4 , 5) . Answer 0 . � � Ω , a ε (1) ( f 1 ) . . . a ε ( n ) ( f 2 n ) Ω � � � � � = f γ − (1) , f γ + (1) . . . f γ − ( n ) , f γ + ( n ) γ ∈P 2 ( ε ) and 0 if the number of f ’s is odd. 7

Answer 1 (Wick formula). � Ω , X ( f 1 ) X ( f 2 ) . . . X ( f 2 n ) Ω � � � � � � = f γ − (1) , f γ + (1) . . . f γ − ( n ) , f γ + ( n ) γ ∈P 2 (2 n ) and 0 if the number of f ’s is odd. Example 2. = � f � 2 n |P 2 (2 n ) | = � f � 2 n (2 n )! � � Ω , X ( f ) 2 n Ω 2 n n ! � 2 π � f � e − x 2 / 2 � f � 2 dx. 1 R x 2 n √ = So X ( f ) has the normal distribution with mean 0 , standard deviation � f � . 8

W ICK PRODUCTS A Wick product W ( f 1 , f 2 , . . . , f n ) =: X ( f 1 ) X ( f 2 ) . . . X ( f n ) : is obtained by expanding � a ε (1) ( f 1 ) . . . a ε ( n ) ( f n ) X ( f 1 ) X ( f 2 ) . . . X ( f n ) = ε ∈ I ( n ) and moving all a + to the left! Non-commutative ⇒ get a different operator. Example 3. W ( f 1 , f 2 ) =: X ( f 1 ) X ( f 2 ) : =: ( a + 1 a + 2 + a + 1 a + 1 a − 2 + a − 2 + a − 1 a − 2 ) : = a + 1 a + 2 + a + 2 + a + 1 a − 2 a − 1 + a − 1 a − 2 1 x + 2 + a + 1 , a + = X 1 X 2 − a − 2 a − 1 = X 1 X 2 − [ a − 2 ] = X ( f 1 ) X ( f 2 ) − � f 1 , f 2 � a polynomial in X ( f 1 ) , X ( f 2 ) ! 9

True in general, follows from the recursion W ( f, f 1 , . . . , f n ) = X ( f ) W ( f 1 , . . . , f n ) n � � f, f k � W ( f, . . . , ˆ − f k , . . . , f n ) k =1 (exercise) Also note W ( f 1 , . . . , f n ) Ω = a + ( f 1 ) . . . a + ( f n ) Ω = f 1 ⊗ . . . ⊗ f n since only the first term in the sum makes a non-zero contri- bution. Question 2. Express W ( f 1 , . . . , f n ) in terms of the usual products. Question 3. Express X ( f 1 ) . . . X ( f n ) in terms of the Wick products. Denote by P 2 , 1 ( n ) the set of all incomplete pairings (match- ings, left-open pair partitions, incomplete Feynman diagrams): γ ∈ P 2 , 1 ( n ) breaks { 1 , . . . n } into 2 -element classes ( γ − ( i ) , γ + ( i )) and one-element classes ( γ 0 ( j )) . Denote by | γ | the total number of classes in γ . 10

Answer 2. Using the recursion relation, W ( f 1 , . . . , f n ) � � � � ( − 1) | γ | � = f γ − ( i ) , f γ + ( i ) X ( f γ 0 ( j ) ) . i j γ ∈P 2 , 1 ( n ) Example 4. X ( f 1 ) X ( f 2 ) X ( f 3 ) Ω = X ( f 1 ) X ( f 2 ) f 3 = X ( f 1 )( f 2 ⊗ f 3 + � f 2 , f 3 � Ω) = f 1 ⊗ f 2 ⊗ f 3 + � f 1 , f 2 � f 3 + � f 1 , f 3 � f 2 + � f 2 , f 3 � f 1 . In general, X ( f 1 ) . . . X ( f n ) Ω � � � � = f γ − ( i ) , f γ + ( i ) f γ 0 (1) ⊗ . . . ⊗ f γ 0 ( k ) . i γ ∈P 2 , 1 ( n ) Therefore Answer 3. X ( f 1 ) . . . X ( f n ) � � � � = f γ − ( i ) , f γ + ( i ) W ( f γ 0 (1) , . . . , f γ 0 ( k ) ) . i γ ∈P 2 , 1 ( n ) 11

Let Y p = W ( f p, 1 , . . . , f p,n p ) , p = 1 , . . . , t . Answer 4. � � � � � Ω , Y 1 . . . Y t Ω � = f γ − ( i ) , f γ + ( i ) γ ∈P 2 i where γ does not connect the elements in the same block. Answer 5. � � � � Y 1 . . . Y t = W ( f γ 0 (1) , . . . , f γ 0 ( k ) ) f γ − ( i ) , f γ + ( i ) γ ∈P 2 , 1 i where γ does not connect the elements in the same block. Use the normal-ordering representation for the proofs. 12