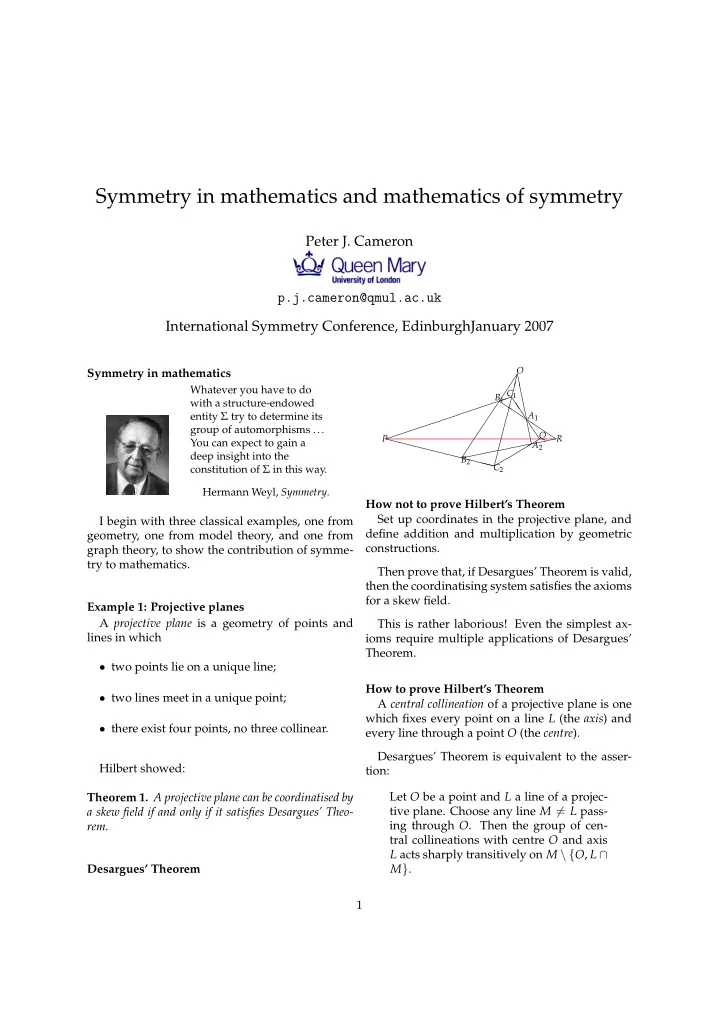

Symmetry in mathematics and mathematics of symmetry Peter J. Cameron p.j.cameron@qmul.ac.uk International Symmetry Conference, EdinburghJanuary 2007 O Symmetry in mathematics ✡ ✄✄ ❉ ❉ Whatever you have to do ✡ B 1 C 1 ✏ ✏✏✏✏✏✏✏✏✏✏ ✄ ❉ ✡ ◗ ❏ with a structure-endowed ◗ ❏ ✄ ❉ ✡ ◗ entity Σ try to determine its A 1 ❏ ✄ ❉ ◗ ✡ group of automorphisms . . . ❏ ◗ ✥✥✥✥✥✥✥✥ ❳❳❳❳❳❳❳❳❳ ✄ ❉ Q ✡ ✧ P R You can expect to gain a ✧✧✧ A 2 ✄ ✡ deep insight into the ❳ ✄ B 2 C 2 constitution of Σ in this way. Hermann Weyl, Symmetry . How not to prove Hilbert’s Theorem Set up coordinates in the projective plane, and I begin with three classical examples, one from define addition and multiplication by geometric geometry, one from model theory, and one from constructions. graph theory, to show the contribution of symme- try to mathematics. Then prove that, if Desargues’ Theorem is valid, then the coordinatising system satisfies the axioms for a skew field. Example 1: Projective planes A projective plane is a geometry of points and This is rather laborious! Even the simplest ax- lines in which ioms require multiple applications of Desargues’ Theorem. • two points lie on a unique line; How to prove Hilbert’s Theorem • two lines meet in a unique point; A central collineation of a projective plane is one which fixes every point on a line L (the axis ) and • there exist four points, no three collinear. every line through a point O (the centre ). Desargues’ Theorem is equivalent to the asser- Hilbert showed: tion: Let O be a point and L a line of a projec- Theorem 1. A projective plane can be coordinatised by tive plane. Choose any line M � = L pass- a skew field if and only if it satisfies Desargues’ Theo- ing through O . Then the group of cen- rem. tral collineations with centre O and axis L acts sharply transitively on M \ { O , L ∩ M } . Desargues’ Theorem 1
Now the additive group of the coordinatising Example 4 . Cantor showed that Q is the unique skew field is the group of central collineations countable dense linearly ordered set without end- with centre O and axis L where O ∈ L ; the multi- points. So Q (as ordered set) is countably categor- plicative group is the group of central collineations ical. ∈ L . We saw that Aut ( Q ) is oligomorphic. where O / So all we have to do is prove the distributive laws (geometrically) and the commutative law of Oligomorphic groups and counting addition (which follows easily from the other ax- The proof of the E–RN–S theorem shows that the number of orbits of Aut ( M ) on M n is equal ioms). to the number of n-types in the theory of M . Example 2: Categorical structures The counting sequences associated with oligo- A first-order language has symbols for variables, morphic groups often coincide with important constants, relations, functions, connectives and combinatorial sequences. quantifiers. A structure M over such a language A number of general properties of such se- consists of a set with given constants, relations, quences are known. To state the next results, we and functions interpreting the symbols in the lan- let G be a permutation group on Ω ; let F n ( G ) be guage. It is a model for a set Σ of sentences if every the number of orbits of G on ordered n -tuples of sentence in Σ is valid in M . distinct elements of Ω , and f n ( G ) the number of orbits on n -element subsets of Ω . A set Σ is categorical in power α (an infinite car- Typically, F n ( G ) counts labelled combinatorial dinal) if any two models of Σ of cardinality α are structures and f n ( G ) counts unlabelled structures. isomorphic. Morley showed that a set of sentences Both sequences are non-decreasing. over a countable language which is categorical in some uncountable power is categorical in all. Sequences from oligomorphic groups So there are only two types of categoricity: Theorem 5. There exists an absolute constant c such countable and uncountable. that, if G is an oligomorphic permutation group on Ω which is primitive (i.e. preserves no non-trivial parti- Oligomorphic permutation groups tion of Ω ), then either Let G be a permutation group on a set Ω . We say that G is oligomorphic if it has only a finite number • f n ( G ) = 1 for all n; or of orbits on the set Ω n for all natural numbers n . • f n ( G ) ≥ c n / p ( n ) and F n ( G ) ≥ n ! c n / q ( n ) , Example 2 . Let G be the group of order-preserving where p and q are polynomials. permutations of the set Q of rational numbers. Two n -tuples a and b of rationals lie in the same Merola gave c = 1.324 . . . . No examples are G -orbit if and only if they satisfy the same equal- known with c < 2. ity and order relations, that is, Theorem 6. Let G be a group with f n ( G ) = 1 for all a i = a j ⇔ b i = b j , a i < a j ⇔ b i < b j . n (in the above notation). Then either So the number of orbits of G on Q n is equal to • G preserves or reverses a linear or circular order the number of preorders on an n -set. on Ω ; or • F n ( G ) = 1 for all n. (In this case we say that G is The theorem of Engeler, Ryll-Nardzewski and highly transitive on Ω .) Svenonius Axiomatisability is equivalent to symmetry! Example 3: Random graphs Theorem 3. Let M be a countable first-order struc- To choose a graph at random, the simplest ture. Then the theory of M is countably categorical if model is to fix the set of vertices, then for each pair and only if the automorphism group Aut ( M ) is oligo- of vertices, toss a fair coin: if it shows heads, join morphic. the two vertices by an edge; if tails, do not join. 2
As a permutation group 2 3 q q ❅ Given a permutation group G on a set Ω , is there ❅ a structure M on Ω of some specified type such ❅ that G = Aut ( M ) ? ❅ 1 4 q q The most interesting case is where M is a re- lational structure over an arbitrary relational lan- guage. { 1, 2 } { 1, 3 } { 1, 4 } { 2, 3 } { 2, 4 } { 3, 4 } • A permutation group on a finite set is the au- tomorphism group of a relational structure. Finite random graphs • A permutation group on a countable set is the Let X be a random graph with n vertices. Then automorphism group of a relational structure • for every n -vertex graph G , the event X ∼ if and only if it is closed in the symmetric = G group (in the topology of pointwise conver- has non-zero probability; gence). • The probability that X ∼ = G is inversely pro- Problem 8. Which permutation groups of countable portional to the number of automorphisms of degree are automorphism groups of relational struc- G ; tures over finite relational languages? • P ( X has non-trivial automorphisms ) → 0 as n → ∞ (very rapidly!) As an abstract group Frucht showed that every abstract group is the So random finite graphs are almost surely asym- automorphism group of some (simple undirected) metric. graph. There are many variations on this theme. But . . . Here are a couple of open questions. The Erd˝ os–R´ enyi Theorem • Every group is the collineation group of a pro- jective plane. But is every finite group the au- Theorem 7. There is a countable graph R such that a tomorphism group of a finite projective plane? random countable graph X satisfies • Is every finite group the outer automorphism P ( X ∼ = R ) = 1. group (automorphisms modulo inner auto- morphisms) of some finite group? Moreover, the automorphism group of R is infinite. We will say more about R and its automorphism Finite permutation groups group later. The study of finite permutation groups has been revolutionised by CFSG (the Classification of Fi- Symmetry and groups nite Simple Groups): The symmetries of any object form a group. Theorem 9. A finite simple group is one of the follow- Is every group the symmetry group of something? ing: This ill-defined question has led to a lot of inter- esting research. We have to specify • a cyclic group of prime order; • whether we consider the group as a permu- • an alternating group A n , for n ≥ 5 ; tation group (so the action is given) or as an • a group of Lie type, roughly speaking a matrix abstract group; group of specified type over a finite field modulo • what kinds of structures we are considering. scalars; 3
Recommend
More recommend