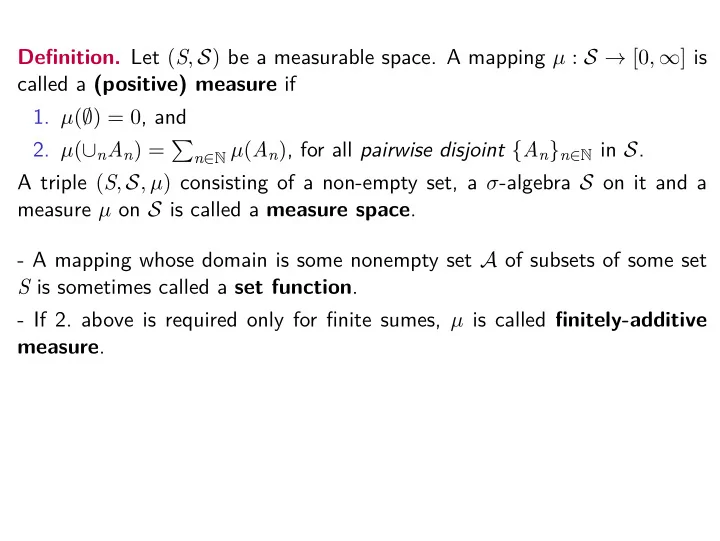

S is sometimes called a set function . called a (positive) measure if measure . Defjnition. Let ( S , S ) be a measurable space. A mapping µ : S → [ 0 , ∞ ] is 1. µ ( ∅ ) = 0 , and 2. µ ( ∪ n A n ) = ∑ n ∈ N µ ( A n ) , for all pairwise disjoint { A n } n ∈ N in S . A triple ( S , S , µ ) consisting of a non-empty set, a σ -algebra S on it and a measure µ on S is called a measure space . - A mapping whose domain is some nonempty set A of subsets of some set - If 2. above is required only for fjnite sumes, µ is called fjnitely-additive
A measure µ on the measurable space ( S , S ) is called 1. a probability measure , if µ ( S ) = 1 , 2. a fjnite measure , if µ ( S ) < ∞ , 3. a σ -fjnite measure , if there exists a sequence { A n } n ∈ N in S such that ∪ n A n = S and µ ( A n ) < ∞ , 4. difguse or atom-free , if µ ( { x } ) = 0 , whenever x ∈ S and { x } ∈ S . A set N ∈ S is said to be null if µ ( N ) = 0 .
uniform measure on S . 1. Measures on countable sets. Suppose that S is a fjnite or countable 3. The counting measure. Defjne the counting measure set. Then each measure µ on S = 2 S is of the form ∑ µ ( A ) = p ( x ) , x ∈ A for some function p : S → [ 0 , ∞ ] . In particular, for a fjnite set S with N elements, if p ( x ) = 1 / N then µ is a probability measure called the 2. Dirac measure. For x ∈ S , we defjne the set function δ x on S by { 1 , x ∈ A , δ x ( A ) = 0 , x ̸∈ A . { # A , A is fjnite , µ : S → [ 0 , ∞ ] by µ ( A ) = ∞ , A is infjnite . .
n (Finite additivity) (Monotonicity of measures) (Continuity with respect to increasing sequences) Let ( S , S , µ ) be a measure space. 1. For A 1 , . . . , A n ∈ S with A i ∩ A j = ∅ , for i ̸ = j , we have ∑ µ ( A i ) = µ ( ∪ n i = 1 A i ) i = 1 2. If A , B ∈ S , A ⊆ B , then µ ( A ) ≤ µ ( B ) 3. If { A n } n ∈ N in S is increasing, then µ ( ∪ n A n ) = lim n µ ( A n ) = sup n µ ( A n ) .
(Subadditivity) (Continuity with respect to decreasing sequences) 4. If { A n } n ∈ N in S is decreasing and µ ( A 1 ) < ∞ , then µ ( ∩ n A n ) = lim n µ ( A n ) = inf n µ ( A n ) . 5. For a sequence { A n } n ∈ N in S , we have ∑ µ ( ∪ n A n ) ≤ µ ( A n ) . n ∈ N
n n Let { A n } n ∈ N be a sequence of subsets of S . We defjne lim inf n A n by lim inf A n = ∪ n B n , where B n = ∩ k ≥ n A k , is called the limit inferior of the sequence A n . It is also denoted by lim n A n or { A n , ev. } ( ev. stands for eventually ). Similarly, the subset lim sup n A n of S , defjned by lim sup A n = ∩ n B n , where B n = ∪ k ≥ n A k , is called the limit superior of the sequence A n . It is also denoted by lim n A n or { A n , i.o. } ( i.o. stands for infjnitely often ). Problem. Let A n = { 0 , 1 n , 2 n , . . . , n 2 n } . Find lim sup A n , lim inf A n . How about if A n = { 0 , 1 2 n } ? 2 n , 2 2 n , . . . , 4 n
n Remember lim sup n A n = ∩ n ∪ k ≥ n A k . Proposition. (The Borel-Cantelli Lemma) Let ( S , S , µ ) be a measure space, and let { A n } n ∈ N be a sequence of sets in S with the property that ∑ n ∈ N µ ( A n ) < ∞ . Then µ (lim sup A n ) = 0 .
cylinders. In the language of elementary probability, each cylinder corresponds to the (1) k times 2 The measure (probability) of this event can only be given by Remember, product cylinders on {− 1 , 1 } N are { } s = ( s 1 , s 2 , . . . ) ∈ {− 1 , 1 } N : s n 1 = b 1 , . . . , s n k = b k C n 1 ,..., n k ; b 1 ,..., b k = , for some k ∈ N , and a choice of 1 ≤ n 1 < n 2 < · · · < n k ∈ N of coordinates and the corresponding values b 1 , b 2 , . . . , b k ∈ {− 1 , 1 } . event when the outcome of the n i -th coin is b i ∈ {− 1 , 1 } , for i = 1 , . . . , n . = 2 − k . µ C ( C n 1 ,..., n k ; b 1 ,..., b k ) = 1 2 × 1 2 × · · · × 1 � �� � The hard part is to extend this defjnition to all elements of S , and not only
Theorem. (Caratheodory’s Extension Theorem) Let S be a non-empty set, with the following properties: Proposition. (Existence of the coin-toss measure) There exists a measure let A be an algebra of its subsets and let µ : A → [ 0 , ∞ ] be a set-function 1. µ ( ∅ ) = 0 , and 2. µ ( A ) = ∑ ∞ n = 1 µ ( A n ) , if { A n } n ∈ N ⊆ A is a partition of A . µ C on ( {− 1 , 1 } N , S ) with the “obvious” values on the cylinders.
the “obvious” values on the cylinders. Theorem. (Dynkin) Let P be a π -system on a non-empty set S , and let Λ be a λ -system which contains P . Then Λ also contains the σ -algebra σ ( P ) generated by P . Proposition. Let ( S , S ) be a measurable space, and let P be a π -system which generates S . Suppose that µ 1 and µ 2 are two measures on S with the property that µ 1 ( S ) = µ 2 ( S ) < ∞ and µ 1 ( A ) = µ 2 ( A ) , for all A ∈ P . Then µ 1 = µ 2 , i.e., µ 1 ( A ) = µ 2 ( A ) , for all A ∈ S . Proposition. The measure µ C is the unique measure on ( {− 1 , 1 } N , S ) with
2 Defjnition. Let ( S , S , µ ) be a measure space and let ( T , T ) be a measurable space. The measure f ∗ µ on ( T , T ) , defjned by f ∗ µ ( B ) = µ ( f − 1 ( B )) , for B ∈ T , is called the push-forward of the measure µ by f . Let f : {− 1 , 1 } N → [ 0 , 1 ] be the mapping given by ∞ ( ) ∑ 2 − k , s ∈ {− 1 , 1 } N . 1 + s k f ( s ) = k = 1 The continuity of f implies that f : ( {− 1 , 1 } N , S ) → ([ 0 , 1 ] , B ([ 0 , 1 ])) is a measurable mapping. Therefore, the push-forward λ = f ∗ ( µ ) is well defjned on ([ 0 , 1 ] , B ([ 0 , 1 ])) , and we call it the Lebesgue measure on [ 0 , 1 ] .
Problem. Show that the Lebesgue measure is translation invariant . More . Proposition. The Lebesgue measure λ on ([ 0 , 1 ] , B ([ 0 , 1 ])) satisfjes λ ([ a , b )) = b − a , λ ( { a } ) = 0 for 0 ≤ a < b ≤ 1 . precisely, for B ∈ B ([ 0 , 1 ]) and x ∈ [ 0 , 1 ) , we have 1. B + 1 x = { b + x (mod 1 ) : b ∈ B } is in B ([ 0 , 1 ]) and 2. λ ( B + 1 x ) = λ ( B ) , where, for a ∈ [ 0 , 2 ) , we defjne a (mod 1 ) = a − 1 { a > 1 } . (( ) For a general B ∈ B ( R ) , we set λ ( B ) = ∑ ∞ ) n = −∞ λ B ∩ [ n , n + 1 ) − n Problem. Let λ be the Lebesgue measure on ( R , B ( R )) . Show that 1. λ ([ a , b )) = b − a , λ ( { a } ) = 0 for a < b , 2. λ is σ -fjnite but not fjnite, 3. λ ( B + x ) = λ ( B ) , for all B ∈ B ( R ) and x ∈ R .
Recommend
More recommend