Linear functors and modal logic natural transformations: L : A ( B - PowerPoint PPT Presentation

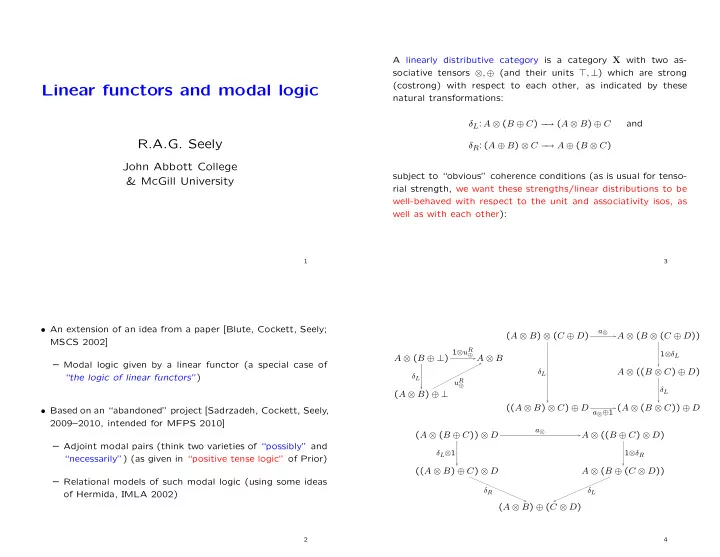
� � � � � � � � � � A linearly distributive category is a category X with two as- sociative tensors ⊗ , ⊕ (and their units ⊤ , ⊥ ) which are strong (costrong) with respect to each other, as indicated by these Linear functors and modal logic natural transformations: δ L : A ⊗ ( B ⊕ C ) − → ( A ⊗ B ) ⊕ C and R.A.G. Seely δ R : ( A ⊕ B ) ⊗ C − → A ⊕ ( B ⊗ C ) John Abbott College subject to “obvious” coherence conditions (as is usual for tenso- & McGill University rial strength, we want these strengths/linear distributions to be well-behaved with respect to the unit and associativity isos, as well as with each other): 1 3 • An extension of an idea from a paper [Blute, Cockett, Seely; a ⊗ ( A ⊗ B ) ⊗ ( C ⊕ D ) A ⊗ ( B ⊗ ( C ⊕ D )) MSCS 2002] 1 ⊗ u R 1 ⊗ δ L ⊕ � A ⊗ ( B ⊕ ⊥ ) A ⊗ B – Modal logic given by a linear functor (a special case of � � � � � A ⊗ (( B ⊗ C ) ⊕ D ) � δ L � � � � “the logic of linear functors” ) δ L � � � � � u R � � � � � � ⊕ � � � δ L � ( A ⊗ B ) ⊕ ⊥ � ( A ⊗ ( B ⊗ C )) ⊕ D (( A ⊗ B ) ⊗ C ) ⊕ D a ⊗ ⊕ 1 • Based on an “abandoned” project [Sadrzadeh, Cockett, Seely, 2009–2010, intended for MFPS 2010] a ⊗ ( A ⊗ ( B ⊕ C )) ⊗ D A ⊗ (( B ⊕ C ) ⊗ D ) – Adjoint modal pairs (think two varieties of “possibly” and δ L ⊗ 1 1 ⊗ δ R “necessarily”) (as given in “positive tense logic” of Prior) (( A ⊗ B ) ⊕ C ) ⊗ D A ⊗ ( B ⊕ ( C ⊗ D )) � � � ���������������������������� – Relational models of such modal logic (using some ideas � � � � � � � � � � � � δ R � δ L � � of Hermida, IMLA 2002) � � � � � � � � � � � ( A ⊗ B ) ⊕ ( C ⊗ D ) 2 4

� � � � � � � � � � � � � � � � � � � � � � Given linearly distributive categories X , Y , a linear functor F : X − → Y consists of: F � ( a ⊕ ) F � (( A ⊕ B ) ⊕ C ) F � ( A ⊕ ( B ⊕ C )) ν L ν R • a pair of functors F � , F � : X − → Y so that F � is monoidal with ⊗ ⊗ respect to ⊗ , and F � is comonoidal with respect to ⊕ : F � ( A ⊕ B ) ⊕ F � ( C ) F � ( A ) ⊕ F � ( B ⊕ C ) m ⊤ : ⊤ − → F � ( ⊤ ) m � : F � ( A ) ⊗ F � ( B ) − → F � ( A ⊗ B ) ν R 1 ⊕ ν L ⊗ ⊕ 1 ⊗ n ⊥ : F � ( ⊥ ) − → ⊥ n � : F � ( A ⊕ B ) − → F � ( A ) ⊕ F � ( B ) � F � ( A ) ⊕ ( F � ( B ) ⊕ F � ( C )) ( F � ( A ) ⊕ F � ( B )) ⊕ F � ( C ) a ⊕ 1 ⊗ ν R • natural transformations (called “linear strengths”): ⊗ F � ( A ) ⊗ F � ( B ⊕ C ) F � ( A ) ⊗ ( F � ( B ) ⊕ F � ( C )) ν R � : F � ( A ⊕ B ) − → F � ( A ) ⊕ F � ( B ) m ⊗ δ L ν L � : F � ( A ⊕ B ) − → F � ( A ) ⊕ F � ( B ) F � ( A ⊗ ( B ⊕ C )) ( F � ( A ) ⊗ F � ( B )) ⊕ F � ( C ) ν R � : F � ( A ) ⊗ F � ( B ) − → F � ( A ⊗ B ) ν R F � ( δ L ) ⊕ ⊕ 1 ν L � : F � ( A ) ⊗ F � ( B ) − → F � ( A ⊗ B ) � F � ( A ⊗ B ) ⊕ F � ( C ) F � (( A ⊗ B ) ⊕ C ) ν R ⊗ satisfying the following coherence conditions: 5 7 1 ⊗ ν L F � ( u L ⊕ ) ⊗ F � ( A ) ⊗ F � ( B ⊕ C ) F � ( A ) ⊗ ( F � ( B ) ⊕ F � ( C )) � F � ( A ) F � ( ⊥ ⊕ A ) m ⊗ δ L ν R u L ⊗ ⊕ F � ( A ⊗ ( B ⊕ C )) ( F � ( A ) ⊗ F � ( B )) ⊕ F � ( C ) � ⊥ ⊕ F � ( A ) F � ( ⊥ ) ⊕ F � ( A ) n ⊥ ⊕ 1 m ⊗ ⊕ 1 F � ( δ L ) � F � ( A ⊗ B ) ⊕ F � ( C ) F � ( a ⊕ ) F � (( A ⊗ B ) ⊕ C ) F � (( A ⊕ B ) ⊕ C ) F � ( A ⊕ ( B ⊕ C )) ν L ⊗ ν R ν R ⊗ ⊗ F � ( A ⊕ B ) ⊕ F � ( C ) F � ( A ) ⊕ F � ( B ⊕ C ) 1 ⊕ ν R n ⊕ ⊕ 1 Of course, all this is much easier to “see” using a graphical calculus with ⊗ “linear functor boxes”, but for a change (!) I won’t use them in this talk . . . � F � ( A ) ⊕ ( F � ( B ) ⊕ F � ( C )) ( F � ( A ) ⊕ F � ( B )) ⊕ F � ( C ) a ⊕ 6 8

� � � � � � � � In “the” process calculus ( e.g. Hennessy & Milner 1985), the Given linear functors F, G : X − → Y , a linear transformation following rule is basic: α : F − → G consists of a pair: A 1 , A 2 , · · · , A m , B ⊢ C 1 , C 2 , · · · , C n � A 1 , � A 2 , · · · , � A m , ♦ B ⊢ ♦ C 1 , ♦ C 2 , · · · , ♦ C n • α � , a monoidal natural transformation F � − → G � This rule holds in basic linear modal logic. • α � , a comonoidal natural transformation G � − → F � . Our intention now is to generalize this logic, to include a second These must satisfy the “obvious” coherence conditions: pair of modalities, � , � , corresponding to a second linear functor α ⊗ G : X − → X , G � = � , G � = � . (In fact, we could generalise the F � ( A ⊕ B ) G � ( A ⊕ B ) situation to F : X − → Y and G : Y − → X , but for simplicity, we ν R ν R shall not do that now.) � � F � ( A ) ⊕ F � ( B ) G � ( A ) ⊕ G � ( B ) � � � ������������������������ � � � � � � The key idea is that of a linear adjunction: Given two linear � � 1 ⊕ α � � α � ⊕ 1 � � � � � � � � functors � � � F : X − → Y and G : Y − → X � F � ( A ) ⊕ G � ( B ) we say that F is left linear adjoint to G , F ⊣ G if this is so in the 2-categorical sense, in the 2-category Lin of linearly distributive (and dual conditions) categories, linear functors, and linear transformations. 9 11 Key example: Basic linear modal logic In essence this means we have (ordinary) natural transformations η � : A − → G � F � ( A ) and ǫ � : F � G � ( A ) − → A Consider a linear functor F : X − → X ; we’ll write � for F � and ♦ η � : G � F � ( A ) − and ǫ � : A − → F � G � ( A ) for F � . A complete description of the modal logic one obtains → A from this is in [BCS 2002], but here are some highlights: plus coherence conditions such as ν L η � � : � ( A ⊕ B ) − → � A ⊕ ♦ B A ⊕ B G � F � ( A ⊕ B ) m � : � A ⊗ � B − → � ( A ⊗ B ) G � ( ν R 1 ⊕ η � � ) In a classical setting, these would be equivalent to A ⊕ G � F � ( B ) G � ( F � ( A ) ⊕ F � ( B )) � � � ������������������������������ � � ( A ⇒ B ) − → ( � A ⇒ � B ) � � � � � � � � � η � ⊕ 1 � ν R � � � � � A ∧ � B − → � ( A ∧ B ) � � � � � � � � � � G � F � ( A ) ⊕ G � F � ( B ) the first being “normality” of the logic, and the second being one half (the linear half!) of the standard isomorphism In other words, we have ordinary adjunctions F � ⊣ G � and G � ⊣ F � → � ( A ∧ B ) � A ∧ � B ← which are “coherent” with respect to one another. 10 12