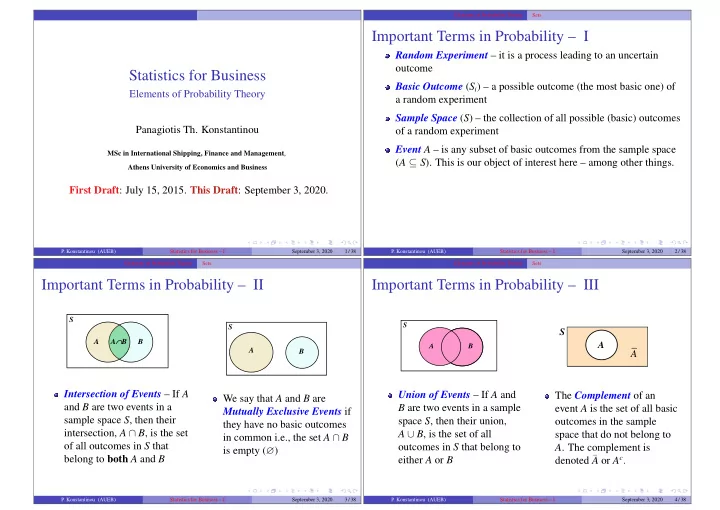

Elements of Probability Theory Sets Important Terms in Probability – I Random Experiment – it is a process leading to an uncertain outcome Statistics for Business Basic Outcome ( S i ) – a possible outcome (the most basic one) of Elements of Probability Theory a random experiment Sample Space ( S ) – the collection of all possible (basic) outcomes Panagiotis Th. Konstantinou of a random experiment Event A – is any subset of basic outcomes from the sample space MSc in International Shipping, Finance and Management , ( A ⊆ S ). This is our object of interest here – among other things. Athens University of Economics and Business First Draft : July 15, 2015. This Draft : September 3, 2020. P. Konstantinou (AUEB) Statistics for Business – I September 3, 2020 1 / 38 P. Konstantinou (AUEB) Statistics for Business – I September 3, 2020 2 / 38 Elements of Probability Theory Sets Elements of Probability Theory Sets ∩ Important Terms in Probability – II Important Terms in Probability – III ∩ ∪ S S S S A ∩ B A B A A B A B A Intersection of Events – If A Union of Events – If A and The Complement of an We say that A and B are and B are two events in a B are two events in a sample event A is the set of all basic Mutually Exclusive Events if sample space S , then their space S , then their union, outcomes in the sample they have no basic outcomes intersection, A ∩ B , is the set A ∪ B , is the set of all space that do not belong to in common i.e., the set A ∩ B of all outcomes in S that outcomes in S that belong to A . The complement is is empty ( ∅ ) denoted ¯ belong to both A and B either A or B A or A c . P. Konstantinou (AUEB) Statistics for Business – I September 3, 2020 3 / 38 P. Konstantinou (AUEB) Statistics for Business – I September 3, 2020 4 / 38
Elements of Probability Theory Sets Elements of Probability Theory Sets Important Terms in Probability – IV Important Terms in Probability – V Events E 1 , E 2 , ..., E k are Collectively Exhaustive events if Examples (Continued) E 1 ∪ E 2 ∪ ... ∪ E k = S , i.e., the events completely cover the sample space. Examples A = { 2 , 4 , 6 } B = { 4 , 5 , 6 } Let the Sample Space be the collection of all possible outcomes of Collectively exhaustive : A and B are not collectively exhaustive. rolling one die S = { 1 , 2 , 3 , 4 , 5 , 6 } . A ∪ B does not contain 1 or 3. Complements : ¯ A = { 1 , 3 , 5 } and ¯ B = { 1 , 2 , 3 } Intersections : A ∩ B = { 4 , 6 } ; ¯ A ∩ B = { 5 } ; A ∩ ¯ B = { 2 } ; Let A be the event “Number rolled is even”: A = { 2 , 4 , 6 } ¯ A ∩ ¯ B = { 1 , 3 } . Let B be the event “Number rolled is at least 4” : B = { 4 , 5 , 6 } Unions : A ∪ B = { 2 , 4 , 5 , 6 } ; A ∪ ¯ A = { 1 , 2 , 3 , 4 , 5 , 6 } = S . Mutually exclusive : A and B are not mutually exclusive. The outcomes 4 and 6 are common to both. P. Konstantinou (AUEB) Statistics for Business – I September 3, 2020 5 / 38 P. Konstantinou (AUEB) Statistics for Business – I September 3, 2020 6 / 38 Elements of Probability Theory Probability Elements of Probability Theory Probability Assessing Probability – I Assessing Probability – II Classical Definition of Probability : 1 Probability – the chance that an uncertain event A will occur is Probability of an event A = N A always between 0 and 1. N number of outcomes that satisfy the event A 0 ≤ Pr( A ) ≤ 1 = ���� total number of outcomes in the sample space S ���� Impossible Certain ◮ Assumes all outcomes in the sample space are equally likely to There are three approaches to assessing the probability of an occur. uncertain event: ◮ Example : Consider the experiment of tossing 2 coins. The sample space is S = { HH , HT , TH , TT } . ◮ Event A = { one T } = { TH , HT } . Hence Pr( A ) = 0 . 5 – assuming that all basic outcomes are equally likely. ◮ Event B = { at least one T } = { TH , HT , TT } . So Pr( B ) = 0 . 75. P. Konstantinou (AUEB) Statistics for Business – I September 3, 2020 7 / 38 P. Konstantinou (AUEB) Statistics for Business – I September 3, 2020 8 / 38
Elements of Probability Theory Probability Elements of Probability Theory Probability Assessing Probability – III Assessing Probability – IV Probability as Relative Frequency : 2 Probability of an event A = n A Subjective Probability : an individual has opinion or belief about 3 n the probability of occurrence of A . = number of events in the population that satisfy event A ◮ When economic conditions or a company’s circumstances change total number of events in the population rapidly, it might be inappropriate to assign probabilities based solely on historical data ◮ The limit of the proportion of times that an event A occurs in a ◮ We can use any data available as well as our experience and large number of trials, n . intuition, but ultimately a probability value should express our degree of belief that the experimental outcome will occur. P. Konstantinou (AUEB) Statistics for Business – I September 3, 2020 9 / 38 P. Konstantinou (AUEB) Statistics for Business – I September 3, 2020 10 / 38 Elements of Probability Theory Probability Elements of Probability Theory Probability Measuring Outcomes – I Measuring Outcomes – II Classical Definition of Probability Classical Definition of Probability Counting Rule for Combinations (Number of Combinations of n Objects taken k at a time): A second useful counting rule enables Basic Rule of Counting : If an experiment consists of a sequence us to count the number of experimental outcomes when k objects of k steps in which there are n 1 possible results for the first step, are to be selected from a set of n objects (the ordering does not n 2 possible results for the second step, and so on, then the total matter) number of experimental outcomes is given by ( n 1 )( n 2 ) ... ( n k ) – � n � n ! C n k = = tree diagram... k !( n − k )! , k where n ! = n ( n − 1 )( n − 2 ) ... ( 2 )( 1 ) and 0 ! = 1. P. Konstantinou (AUEB) Statistics for Business – I September 3, 2020 11 / 38 P. Konstantinou (AUEB) Statistics for Business – I September 3, 2020 12 / 38
Elements of Probability Theory Probability Elements of Probability Theory Probability Measuring Outcomes – III Measuring Outcomes – IV Classical Definition of Probability Classical Definition of Probability ◮ Example : Suppose we flip three coins. How many are the possible ◮ Example : How many possible half-a-dozens we can put together, combinations with (exactly) 1 T ? preserving the ratio 4 : 2 ? � 3 � 3 ! C 3 1 = = 1 !( 3 − 1 )! = 3 . � 6 � � 4 � 1 × = 15 × 6 = 90 . 4 2 ◮ Example : Suppose we flip three coins. How many are the possible ◮ Probability : What is the probability of selecting a particular combinations with at least 1 T ? ◮ Example : Suppose that there are two groups of questions. Group half-a-dozen (with ratio 4 : 2), when we choose at random? Using the classical definition of probability A with 6 questions and group B with 4 questions. How many are the possible half-a-dozens we can put together? 90 210 = 0 . 4286 � 10 � 10 ! n = 6 + 4 = 10 ; C 10 6 = = 6 !( 10 − 6 )! = 210 . 6 P. Konstantinou (AUEB) Statistics for Business – I September 3, 2020 13 / 38 P. Konstantinou (AUEB) Statistics for Business – I September 3, 2020 14 / 38 Elements of Probability Theory Probability Elements of Probability Theory Probability Measuring Outcomes – V Measuring Outcomes – VI Classical Definition of Probability Classical Definition of Probability ◮ Example : How many 3-digit lock combinations can we make from Counting Rule for Permutations (Number of Permutations of n Objects taken k at a time): A third useful counting rule enables us the numbers 1, 2, 3, and 4? The order of the choice is important! So to count the number of experimental outcomes when k objects are to be selected from a set of n objects, where the order of 3 = 4 ! P 4 1 ! = 4 ! = 4 ( 3 )( 2 )( 1 ) = 24 . selection is important n ! ◮ Example : Let the characters A , B , Γ . In how many ways can we P n k = ( n − k )! . combine them in making triads? 3 = 3 ! P 3 0 ! = 3 ! = 3 ( 2 )( 1 ) = 6 . These are: AB Γ , A Γ B , BA Γ , B Γ A , Γ AB , and Γ BA . P. Konstantinou (AUEB) Statistics for Business – I September 3, 2020 15 / 38 P. Konstantinou (AUEB) Statistics for Business – I September 3, 2020 16 / 38
Recommend
More recommend