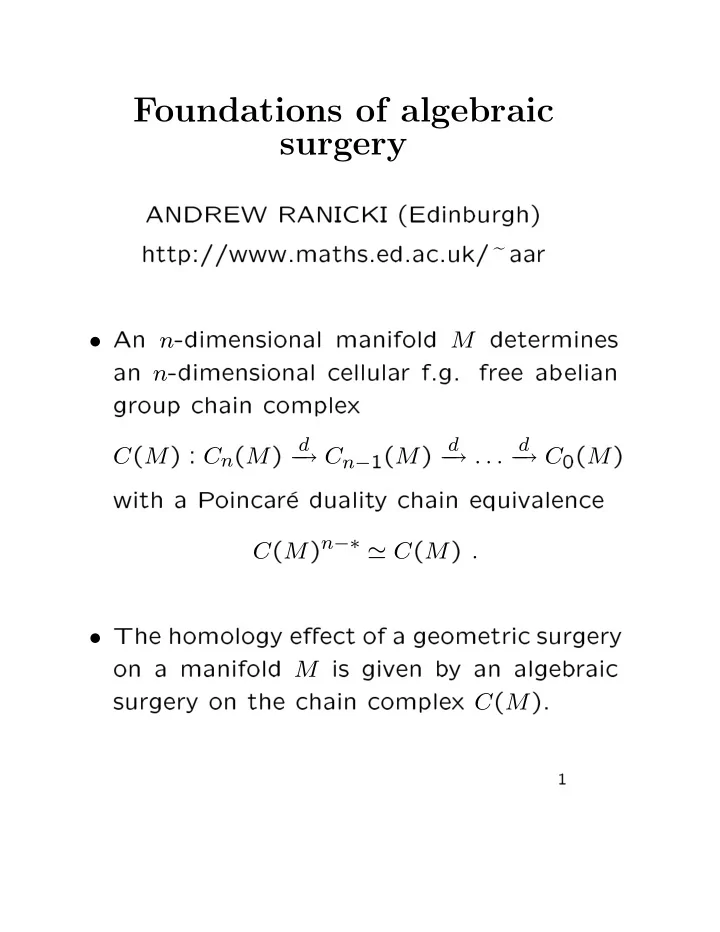
Foundations of algebraic surgery ANDREW RANICKI (Edinburgh) - PDF document

Foundations of algebraic surgery ANDREW RANICKI (Edinburgh) http://www.maths.ed.ac.uk/ � aa r • An n -dimensional manifold M determines an n -dimensional cellula r f.g. free ab elian group chain complex ) d ) d → . . . d ( M ) : C n ( M ( M ( M ) C − → C n − − − → C 1 0 with a P oinca r � e dualit y chain equivalence ) n −∗ ≃ C C ( M ( M ) . • The homology e�ect of a geometric surgery on a manifold M is given b y an algeb raic surgery on the chain complex C ( M ). 1

The algeb raic surgery machine • The algeb raic pa rt of the machine stud- ies chain complexes with P oinca r � e dualit y , which a re quadratic fo rms on chain com- plexes. Algeb raic surgery on such objects mo dels the surgery of manifolds and no r- mal maps. • The geometric pa rt reduces top ological surgery p roblems to algeb raic ones. • F o r n -dimensional no rmal maps with n ≥ 5 there is a one-one co rresp ondence b et w een algeb raic and geometric surgery b elo w and in the middle dimensions. 2

Symmetric and quadratic P oinca r � e dualit y • General theo ry applies to chain complexes C over any ring with involution A . There y C n −∗ ≃ C a re t w o t yp es of P oinca r � e dualit , co rresp onding to t yp e I and t yp e I I sym- metric fo rms. • Use symmetric P oinca r � e dualit y fo r surgery on manifolds. In general, cannot realize symmetric P oinca r � e surgeries on manifolds. • Use quadratic P oinca r � e dualit y fo r surgery on no rmal maps. • Theo rem The W all surgery obstruction group ( Z [ π ]) is the cob o rdism group of n -dimensional L n quadratic P oinca r � e complexes over Z [ π ]. 3

Geometric surgery • The n -dimensional manifold obtained from an n -dimensional manifold M b y surgery on S i × D n − i ⊂ M is ( M \ S i × D n − i ) ∪ D i +1 × S n − i − 1 . M ′ = Call this the e�ect of the surgery . obtain M ′ • Can from M b y surgery on D i +1 × S n − i − 1 ⊂ M ′ . ( D i +1 × D n − i View S n • Example = ∂ ) as = S i × D n − i ∪ D i +1 × S n − i − 1 . S n on S i × D n − i ⊂ S n Surgery gives 1 . D i +1 × S n − i − 1 ∪ D i +1 × S n − i − 1 = S i +1 × S n − i − 4

Cob o rdism e�ect of surgery on S i × D n − i ⊂ M • The trace of surgery with e�ect M ′ ; M, M ′ is the cob o rdism ( W ) obtained from M × [0 , 1] b y attaching an ( i + 1)-handle at S i × D n − i × { 1 } ⊂ M × { 1 } 1] ∪ D i +1 × D n − i . W = M × [0 , • Theo rem (Thom, Milno r) Every ( n +1)-dimensional cob o rdism ( L ; M, N ) is a union of the traces of surgeries. Pro of A Mo rse function f : ( L ; M, N ) → ([0 , 1]; { 0 } , { 1 } ) determines a handle decomp osition of L on M n +1 � � D k × D n +1 − k L = M × [0 , 1] ∪ k =0 with one k -handle fo r each critical p oint of in- dex k . 5

Homotop y e�ect of surgery : S i → M The mapping cone of a map x is • space M ∪ x D i +1 the adjunction obtained from M b y attaching an ( i + 1)-cell. ; M, M ′ • Prop osition If ( W ) is the trace of a on S i × D n − i ⊂ M surgery there a re de�ned homotop y equivalences ≃ M ∪ x D i +1 ≃ M ′ ∪ x ′ D n − i W = S i × { 0 } ⊂ S i × D n − i ⊂ M : S i with x . • Surgery on an n -dimensional manifold at- taches an ( i + 1)-cell and then detaches an ( n − i )-cell. ; S n , S i +1 × S n − i − 1 • Example The trace ( W ) on S i × D n − i ⊂ S n of the surgery has ≃ S n ∨ S i +1 . W 6

Homology e�ect of attaching a cell • The algeb raic mapping cone of a chain map f : C → D is the chain complex C ( f ) with � � d C 0 C ( f ) r = C r − 1 ⊕ D r , d C = . ( f ) ± f d D • F o r i ≥ 0 de�ne the chain complex S i Z : · · · → 0 → Z → 0 → . . . concentrated in dimension i . • The homology e�ect of attaching an : S i → M ( i + 1)-cell to M at x is to attach an algeb raic ( i + 1)-cell to C ( M ) : = M ∪ x D i +1 if W then : S i Z → C C ( W ) = C ( x ( M )) In pa rticula r, H i ( W ) = H i ( M ) / � x � is ob- • tained from H i ( M ) b y killing x ∈ H i ( M ). 7

Homology e�ect of surgery ; M, M ′ • If ( W ) is the trace of a surgery on S i × D n − i ⊂ M then there a re de�ned chain equivalences : S i Z → C C ( W ) ≃ C ( x ( M )) ( x ′ : S n − i − 1 Z → C ( M ′ ( W ) ≃ C )) . C ( M ′ ) obtained from C ( M ) b y an algeb raic • C surgery which kills x ∈ H i ( M ), b y �rst at- taching an algeb raic ( i + 1)-cell and then detaching an algeb raic ( n − i )-cell. • Need P oinca r � e dualit y to describ e the re- and x ′ ∈ lationship b et w een x ∈ H i ( M ) ( M ′ H n − i − ). 1 8

P oinca r � e dualit y Cap p ro duct with fundamental class [ M ] ∈ • H n ( M ) is a chain equivalence ) n −∗ → C [ M ] ∩ − : C ( M ( M ) inducing the P oinca r � e dualit y isomo rphisms ) ∼ H n −∗ ( M = H ∗ ( M ) . • P oinca r � e-Lefschetz dualit y fo r any cob o r- ; M, M ′ dism ( W ) ) ∼ H n +1 −∗ ( W, M ′ ( W, M = H ∗ ) . • Can use P oinca r � e dualit y to decide which elements in H i ( M ) can b e rep resented b y S i × D n − i ⊂ M and so killed b y surgery . Rega rding dualit y as a quadratic fo rm, can only kill isotropic elements. 9

Principle of Algeb raic Surgery • F o r any cob o rdism of n -dimensional mani- ; M, M ′ folds ( W ) the chain homotop y t yp e ( M ′ of C ) and its P oinca r � e dualit y can b e obtained from the chain homotop y t yp e of C ( M ) and { its P oinca r � e dualit y the chain homotop y class of the chain { ( W, M ′ map j : C ( M ) → C ) ( W, M ′ { j [ M ] = 0 ∈ H n ) on the chain level using algeb raic surgery on symmetric P oinca r � e complexes. • An algeb raic surgery co rresp onds to a se- quence of geometric surgeries. 10

Symmetric P oinca r � e complexes • An n -dimensional symmetric P oinca r � e complex ( C, φ ) is an n -dimensional f.g. free chain complex C with mo rphisms : C r φ s = Hom Z ( C r , Z ) → C n − r ( s ≥ 0) + s such that (up to signs) : C r → C n − r + φ s d ∗ + φ ∗ dφ s + φ s − = 0 1 + s − 1 s − 1 with s ≥ 0, φ − = 0 and 1 : C n −∗ φ = Hom Z ( C, Z ) n −∗ → C 0 is a chain equivalence. • Symmetric fo rm on chain complex. Theo rem (Mishchenk o) An n -dimensional • manifold M determines an n -dimensional symmetric P oinca r � e complex ( C ( M ) , φ ), with ≃ ) n −∗ φ = [ M ] ∩ − : C ( M − → C ( M ) 0 11

Symmetric algeb raic surgery • An algeb raic surgery on ( C, φ ) has input a chain map j : C → D with chain homotop y 0 j ∗ ≃ : D n −∗ → D . δφ : jφ 0 0 The e�ect is the n -dimensional symmetric ( C ′ , φ ′ P oinca r � e complex ) with +1 , C ′ +1 ⊕ D n − r = C r ⊕ D r r   0 j ∗ d C 0 ± φ   d C ′ = ± j d D δφ   0 ) ∗ 0 0 ( d D • Generalization of the op eration which re- = λ ∗ places a symmetric fo rm ( K, λ : K → K ∗ ) fo r any x ∈ K with λ ( x )( x ) = 0 (isotropic) b y the sub quotient fo rm ( K ′ , λ ′ ) = ( { y ∈ K | λ ( x )( y ) = 0 } / � x � , [ λ ]) 12

Algeb raic and geometric surgery on S i × D n − i ⊂ M • Surgery determines al- geb raic surgery on ( C ( M ) , φ ) with input ) → S n − i Z j : C ( M a co cycle rep resenting dual j ∈ H n − i the P oinca r � e ( M ) of x = [ S i ] ∈ H i ( M ), and δφ determined b y fram- of S i ⊂ M ing . • Theo rem The symmetric P oinca r � e complex ( M ′ ) , φ ′ e�ect M ′ ( C ) of the geometric is the e�ect of the algeb raic surgery on ( C ( M ) , φ ). • Exercise W o rk out the algeb raic surgery co rresp onding to the geometric surgery on S i × D n − i ⊂ S n e�ect S i +1 × S n − i − 1 with . 13

The algeb raic e�ect of a surgery • The Theo rem is an example of the Alge- b raic Surgery Principle in action. ; M, M ′ on S i × • If ( W ) is the trace of surgery D n − i ⊂ M then ( W, M ′ ) ≃ S n − i Z . j : C ( M ) → C • W rite C ( M ) = C , and let x ∈ C i b e cycle = j ∈ C n − i b eing killed, y the dual co cycle. ( M ′ • The chain complex C ) is chain equiva- lent to d ⊕ y 1 ⊕ Z d ⊕ 0 C ′ : · · · → C n − i − − → C n − i − − − − → C n − i − 2 0 d ⊕ → C i +1 ⊕ Z d ⊕ x → · · · → C i +2 − − − − − − → C i → . . . 14