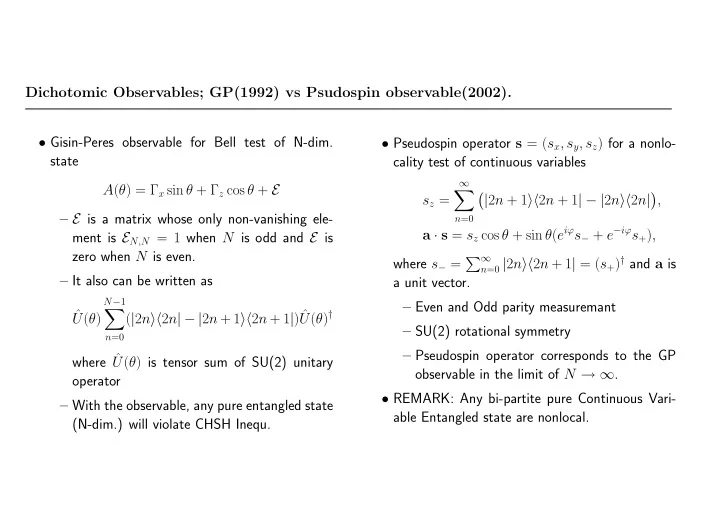
Dichotomic Observables; GP(1992) vs Psudospin observable(2002). - PowerPoint PPT Presentation

Dichotomic Observables; GP(1992) vs Psudospin observable(2002). • Gisin-Peres observable for Bell test of N-dim. • Pseudospin operator s = ( s x , s y , s z ) for a nonlo- state cality test of continuous variables ∞ A ( θ ) = Γ x sin θ + Γ z cos θ + E � � � s z = | 2 n + 1 �� 2 n + 1 | − | 2 n �� 2 n | , – E is a matrix whose only non-vanishing ele- n =0 a · s = s z cos θ + sin θ ( e iϕ s − + e − iϕ s + ) , ment is E N,N = 1 when N is odd and E is zero when N is even. n =0 | 2 n �� 2 n + 1 | = ( s + ) † and a is where s − = � ∞ – It also can be written as a unit vector. N − 1 – Even and Odd parity measuremant ˆ � ( | 2 n �� 2 n | − | 2 n + 1 �� 2 n + 1 | ) ˆ U ( θ ) † U ( θ ) – SU(2) rotational symmetry n =0 – Pseudospin operator corresponds to the GP where ˆ U ( θ ) is tensor sum of SU(2) unitary observable in the limit of N → ∞ . operator • REMARK: Any bi-partite pure Continuous Vari- – With the observable, any pure entangled state able Entangled state are nonlocal. (N-dim.) will violate CHSH Inequ.

Dichotomic Observables; Wiger(parity,CHSH) vs Q (present,CH) Banaszek and Wodkiewicz, PRL(1998) • Parity measurement and displacement operation • Photon presence measurement with dispalcement operation Π( α ) = Π + ( α ) − Π − ( α ) Q ( α ) = ˆ ˆ D ( α ) | 0 �� 0 | ˆ D ( α ) † ∞ � � � D † ( α ) = D ( α ) | 2 n �� 2 n | − | 2 n + 1 �� 2 n + 1 | ∞ P ( α ) = ˆ ˆ � | n �� n | ˆ D ( α ) † D ( α ) n =0 n =1 – D ( α ) is the displacement operator D ( α ) = a † − α ∗ ˆ a † . – It satisfies the completeness relation exp[ α ˆ a ] for bosonic operators ˆ a , ˆ Q ( α ) + ˆ ˆ P ( α ) = 1 – The Wigner fuction from the operator – Assign 1 to no counts and 0 to triggering W ( α ) = Tr Π( α )ˆ ρ 1 – The Q-Function W ab ( α ; β ) = Tr Π( α ) ⊗ Π( β )ˆ ρ ab Q ab ( α, β ) = 1 π 2 Tr ˆ Q ( α ) ⊗ ˆ Q ( β ) ρ ab – CHSH inequality can be constructed |�B CHSH �| = π 2 – CH inequality can be constructed 4 | W ( α, β ) + W ( α, β ′ ) − 1 ≤ π 2 [ Q ab ( α, β ) + Q ab ( α, β ′ ) + Q ab ( α ′ , β ) + W ( α ′ , β ) − W ( α ′ , β ′ ) | ≤ 2 , − Q ab ( α ′ , β ′ )] − π [ Q a ( α ) + Q b ( β )] ≤ 0

Continuous Variable State; EPR State • EPR state ; Two mode momentum eigenstate • Optimized violation of CHSH inequality with total momentum p 1 + p 2 = 0 and relative – Psudospin operator (dotted) position x 1 − x 2 = x 0 – Parity op. (BW solid, Our dashed) – EPR state corresponds to a two-mode squeezed state for infinity squeezing limit ∞ (tanh r ) n � | TMSS � = cosh r | n, n � n =0 � = dxC ( x, r ) | x, x � – Gaussian state – It can be generated by beam splitter or para- metric down conversion Kim and Sanders, PRA(1996);Hong and Mandel,PRL(1984)

Continuous Variable State; EPR State • Optimized violation of CH inequality • Violation of CHSH inequality goes maximum as r → ∞ while CH inequality goes 0. – Psudospin operator (CHSH, dotted) • For the case of EPR state, maximum violation of – Photon presence measurement. (BW solid, CHSH is | B BW | max → 8 / 3 9 / 8 ≃ 2 . 32 . Our dashed) • EPR state does not maximally violate CHSH in the generalized BW observable. • Parity meaurement gives higher violaiton for TMSS altough it is experimentally difficult.

Unitary Operators • Rotation of pseudospin operator a · s a · s | 2 n + 1 � = cos θ | 2 n + 1 � + sin θ | 2 n � a · s | 2 n � = − sin θ | 2 n + 1 � + cos θ | 2 n � • The expectation value of the parity operator for a number state P ( n, | α | ) = � n | Π( α ) | n � ∞ = e −| α | 2 | α | 2 n � (2 k )! � 2 L ( n − 2 k ) � ( | α | 2 ) � 2 k | α | 4 k n ! k =0 − (2 k + 1)! � 2 � L ( n − 2 k − 1) ( | α | 2 ) � , 2 k +2 | α | 4 k +2 where L ( p ) q ( x ) is an associated Laguerre polyno- mial.

Bell’s inequality with finite outcome measurement (W.Son et al. , will be appeared) • Collins version of Bell’s inequality (2002) – Bell’s inequality for N-dim. system using finite outcome measurement. – Agree with the results that noise robustness of violations of local realism. ( D. Kaszlikowski et al. ,2000 ) • Bell’s inequality test for TMSS using finite out- come. measurement – Always violate the Bell’s inequality. – Infinite squeezing does not gives maximum vi- olation. – Higher squeezing ; The more outcome the more violation. – Small squeezing ; The more outcome the less violation.

Summary and Future work • Summary • Future work – We discussed for the link between the nolocal- – Noise effect for the nonlocality tests. ity of finite and infinte dimensional systems by – Find the implication of the state and the mea- showing origin of psudospin operator. surements in quantum information precessing. – We found the maximal bound of violation of (cf Quantum cryptography) Bell’s inequality for BW formalism. – Nonlocality test for different CV state with different dichotomic observables – We discussed the relation between the nonlo- cality and unitary operators of measurement process. – Nonlocality test with more than two outcome.