
xford hysics split into two energetic collinear partons emit soft gluons that I do not deflect the I energetic quark I I Automating Calculations in Soft Collinear Effective Theory Guido Bell || Rudi Rahn || Jim Talbert 6 May 2015 || Max-Planck-Institut für Physik, München
Outline 1. Motivating an effective theory of QCD (a) Collider physics and the separation of scales (b) Resummation examples: Z @ small p T and thrust 2. A brief introduction to SCET (c) Formalism, power counting, and momentum mode suppression (d) SCET Lagrangian (e) SCET dijet factorisation (f) Renormalisation group resummation (for thrust) 3. Universal soft functions at NLO (g) Divergence structures, measurement functions, and Laplace space (h) naive subtraction 4. Soft automation to NNLO (i) NLO vs. NNLO: the need for automation (j) Sector Decomposition, parameterising phase space, and SecDec (k) Analytic regulator at NNLO (l) Results 2
Why an EFT for QCD? A perturbative description of collider phenomena with widely separated • momentum scales generically involves large logarithms of the scales’ ratios—these must be resummed : ✓ µ 1 ◆ ↵ n s ln m µ 2 Traditional approaches in QCD based on coherent branching algorithm (CTTW) • which sums probabilities of independent gluon emission diagrammatically Effective field theories allow for analytic resummation using renormalisation • group techniques at the amplitude level…very efficient. Hierarchy of scales implemented at the level of the Lagrangian… • 3
Resummation I: Z @ small p T 1109.6027(Becher/Neubert/Wilhelm) ✓ m 2 ◆ • For pp -> Z + X @ small p T all radiation is s ln 2 n α n Z p 2 confined to the beam or soft T • Perturbative expansion plagued by large 25 logarithms of m Z /p T — resummation 20 required. � pb � GeV � NLL 15 NNLL • Traditional QCD resummation using CSS dq T d Σ 10 ( N. Phys. B250 ) , incomplete NNLL (hep-ph/0302104) 5 0 • Becher, Neubert, and Wilhelm achieve 6 0 2 4 6 8 10 12 14 NNLL resummation via SCET methods. q T � GeV � p T distribution of Z-boson production @ LHC 4
Resummation II: thrust Thrust is an e + e - event shape —a geometric, dimensionless physical observable characterising the momentum distribution of particles two-jet like: T ' 1 spherical: T ' 1 / 2 5
Resummation II: thrust 0803.0342v2(Becher/Schwartz) Traditional QCD resummation achieved by CTTW @ NLL (Nucl. Phys. B407 [1993]) • Recently extended to NNLL (1105.4560; Monni, Gehrmann, Luisoni) • Becher, Schwartz achieve N 3 LL resummation using SCET methods (kind of) • s ln 2 n τ α n τ = 1 � T 2.0 2.0 matched fixed order resummed and matched order 15 15 4 th order 1.5 1.5 1.5 order NNLO 15 3 rd order order NLO 2 nd order 10 10 order LO 1 d Σ 1 d Σ 10 1.0 1 st order 1.0 1.0 dT dT Σ Σ 5 5 5 0.5 0.5 0.5 0 0 0 0.0 0.1 0.2 0.3 0.4 0.0 0.1 0.2 0.3 0.4 0.0 0.1 0.2 0.3 0.4 0.0 0.0 0.0 0.10 0.15 0.20 0.25 0.30 0.10 0.15 0.20 0.25 0.30 0.10 0.15 0.20 0.25 0.30 1 � T 1 � T matched order matched 1.5 1.5 1.5 Red = Aleph data 6 1.0 1.0 1.0 0.5 0.5 0.5 0.0 0.0 0.0 0.10 0.15 0.20 0.25 0.30 0.10 0.15 0.20 0.25 0.30 0.10 0.15 0.20 0.25 0.30
Resummation: 𝛽 s Resummed results used to perform precision extractions of strong coupling: • determination from event shape fits α s ( m Z ) MC power Analytic power [Dissertori et al] corrections corrections 0.125 FO τ , ρ , Y 3 , B ρ [Chien & Schwartz] tail fits moments fits τ , ρ , Y 3 , B τ 0.120 world average τ [Dissertori et al] [Gehrmann et al] [AFHMS] N 2 LL NLL HKMS resummation 0.115 [Becher & Schwartz] τ C-param N 3 LL [Davison Webber] NLL resummation [AFHMS] τ , ρ , Y 3 , B N 3 LL [Gehrmann et al] 0.110 [compilation from A. Hoang, QCD workshop, Singapore, March 2013] -> Higher log resummations reduce uncertainties. • -> Precision fits are lower than the world average. • 7
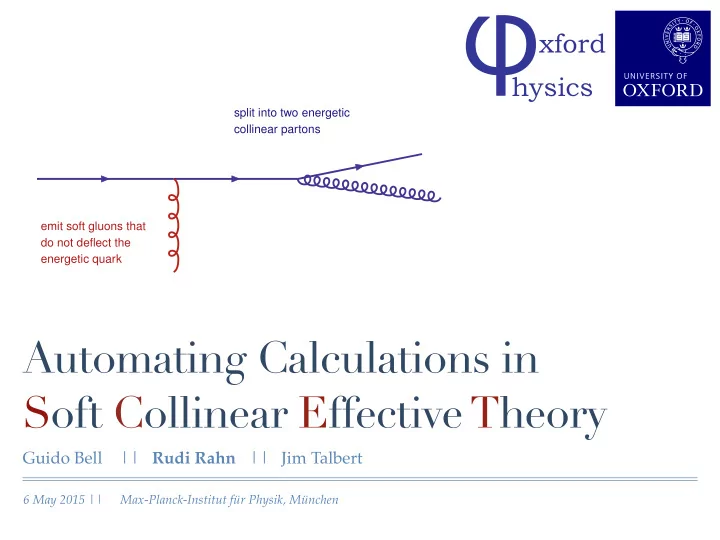
Introducing SCET: intuition SCET is an effective theory whose degrees of freedom are soft and • collinear partons ⌧ split into two energetic collinear partons E J ∼ Q m J split into two energetic collinear partons E s ∼ m 2 J Q I emit soft gluons that I do not deflect the I energetic quark I I split into two energetic collinear partons jet m 2 J ⌧ E 2 ) jet of collinear particles J emit soft gluons that I I soft large-angle radiation E s ⌧ E J do not deflect the I energetic quark I I 8
Introducing SCET: notation SCET is formulated in light-cone basis: • n µ = (1 , 0 , 0 , 1) n µ = (1 , 0 , 0 , − 1) ¯ n · n = 0 = ¯ n · ¯ n · ¯ n = 2 n Such that any vector or invariant can be parameterised as follows: • n µ 2 n · p + n µ p µ = ¯ n · p + p ⊥ ,µ ≡ ( p + , p − , p ⊥ ) 2 ¯ p 2 = p + p − + p 2 ⊥ p · q = 1 2 p + · q − + 1 2 p − · q + + p ⊥ · q ⊥ 9
Introducing SCET: power-counting Separation of scales in EFTs characterised by power counting expansion parameter, • in SCET this parameter changes depending on observable, e.g.: λ = p T (Z @ small p T ) λ = √ τ (thrust) M Z Momentum scaling is then determined for each relevant type of particle. • Consider back-to-back light jets on the light cone, with background soft radiation: Collinear scaling along + p µ ∼ Q (1 , λ 2 , λ ) + , − , ⊥ q µ ∼ Q ( λ 2 , 1 , λ ) Collinear scaling along - k µ ∼ Q ( λ 2 , λ 2 , λ 2 ) Ultrasoft scaling 10
Introducing SCET: SCET I vs. SCET II There are two types of SCET, depending on the relative scaling of soft and collinear modes: • Thrust (SCET I ) Broadening (SCET II ) Thrust (SCET I ) Z @ small p T (SCET II ) k − k − collinear hard collinear hard Q Q soft p T b soft anti-collinear τ Q anti-collinear b 2 /Q p 2T /Q k + k + τ Q Q Q b 2 /Q b p 2T /Q p T p 2 s ⌧ p 2 Thrust I thrust: c Z small p T I broadening: p 2 s ⇠ p 2 c In SCET II scaling alone does not suffice to differentiate—can only distinguish modes from their • rapidity… 11
Introducing SCET: effective Lagrangian · Begin with fundamental QCD fields and split into soft and collinear components: • A µ ( x ) → A µ c ( x ) + A µ Ψ µ ( x ) → Ψ µ c ( x ) + Ψ µ s ( x ) s ( x ) Further project collinear fermion into two components: • n/ ⌘ ( x ) = / ⇣ ( x ) = / n ¯ n/ ¯ n 4 Ψ c ( x ) , 4 Ψ c ( x ) Now consider 2-pt correlators, and determine how field scales: • ζ (0) }| 0 i ⇠ λ 2 ) ζ ( x ) ⇠ λ h 0 |{ ζ ( x )¯ ( η ( x ) ⇠ λ 2 ) Now, integrate out power suppressed modes. Note, this is not a traditional EFT! Let’s look • at the collinear portion of the SCET Lagrangian: ◆ / n ✓ ¯ 1 c c Ψ i / in · D + i / n · Di / L QCD = ¯ D Ψ L collinear = ¯ D ⊥ D ⊥ ⇣ 2 ⇣ ⇒ i ¯ c ✓ ◆ Z in · D = in · ∂ + gn · A c + gn · A s only collinear-soft interaction at leading order in λ 12
Introducing SCET: dijet factorisation We can derive all order factorisation theorems in SCET. Two critical steps. “Hard-Collinear • factorisation” (1) & “Soft-decoupling” (2): Z Ψ (0) � µ Ψ (0) ! ¯ dsdt C V ( s, t ) (¯ n )( sn ) � µ ⊥ ( W † (1) n ⇣ n )( t ¯ n ) ⇣ ¯ n W ¯ ✓ Z · Z 0 ✓ ◆ W c = Pexp ig ds ¯ n · A c ( x + s ¯ n ) −∞ C V (s,t) is a Wilson coefficient to be determined in matching QCD to SCET • Explicit non-locality along light-cone directions manifest -> Wilson lines necessary for gauge • invariance. After a field redefinition, we obtain (some spatial dependence suppressed): ⇣ n ( x ) = S n ( x − ) ⇣ 0 n ( x ) Z Ψ (0) � µ Ψ (0) ! ¯ dsdt C V ( s, t ) ¯ n W 0 , † n S † n � µ ⇣ 0 ⊥ W 0 n S n ⇣ 0 (2) n ¯ ¯ ¯ Now the Lagrangian contains no interactions between collinear and soft fields (at leading order), but • the current still contains both… 13
Thrust w/ SCET: factorisation Σ i | p i · n | thrust T = max n Σ i | p i | We can thus factorise our matrix element for the dijet, two-fermion operator quite simply: • | C V | 2 Σ n | X i | 2 X | h 0 |O n ¯ i † i † ⇤ † | 0 i h 0 | = | C V | 2 h 0 | h i h h i h ¯ ¯ n W 0 , † n W 0 , † S † S † ⇣ 0 ⇣ 0 W 0 n ⇣ 0 W 0 n ⇣ 0 ⇥ ⇤ ⇥ | 0 i h 0 | | 0 i n S n n S n n n ¯ ¯ ¯ ¯ n n ¯ ¯ | | (for dijet R , µ ) S ( τ Q − p 2 L + p 2 1 d σ Z Z d τ = H ( Q 2 , µ ) dp 2 dp 2 J ( p 2 L , µ ) J ( p 2 R , µ ) L R thrust) Q σ 0 J ( p 2 H ( Q 2 ) J ( p 2 L ) R ) “soft-decoupling” S ( µ 2 S ) 14
Thrust w/ SCET: resummation H, J i , and S contain logs of the form (respectively): • ln µ 2 ln µ 2 µ 2 Q 2 , ⌧ Q 2 , ln ⌧ 2 Q 2 We evaluated H, J i , and S at a common scale. Yet there are ‘natural’ scales at which the logarithms are no • ⊥ ⊥ longer large: µ j ∼ Q √ ⌧ µ s ∼ Q ⌧ µ h ∼ Q We thus wish to RG run our functions up to their natural scales. Take H as a simple example: • H ( Q 2 , µ ) = H ( Q 2 , µ h ) U h ( µ h , µ ) Where the function U is a solution to the RG equation for the hard function: � • dH ( Q 2 , µ ) ✓ Q 2 ◆ � H ( Q 2 , µ ) = 2 Γ cusp ln + 4 γ H ( α s ) d ln µ µ 2 Which, at LL approximation, has the following form: • ✓ Q 2 4 ⇡ Γ 0 1 ✓ 1 − 1 ◆� ↵ s ( Q ) ◆ r = ↵ s ( µ ) = 1 − Γ 0 H ( Q 2 , µ ) = exp ln 2 + O ( ↵ 2 r − ln r s ) , � 2 µ 2 ↵ s ( Q ) 2 4 ⇡ ↵ s ( Q ) 0 Similar for jet and soft functions… • 15
Recommend
More recommend